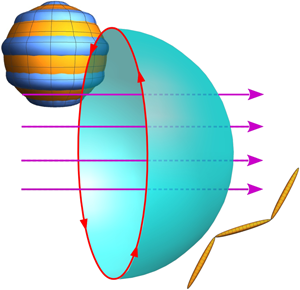
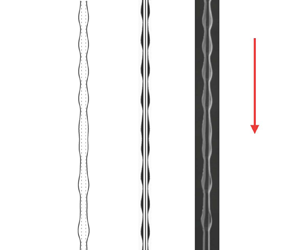
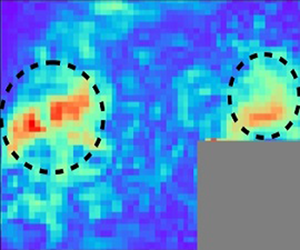
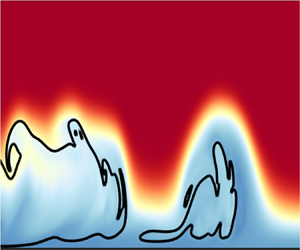
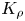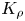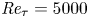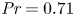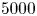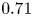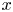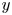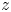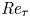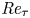Graphical abstract from Boudina, M., Gosselin, F. & Étienne, S. 2021. Vortex-induced vibrations: A soft coral feeding strategy? J. Fluid Mech.916, A50. doi:10.1017/jfm.2021.252.
Contents
JFM Papers
A continuum model to study fluid dynamics within oscillating elastic nanotubes
-
- Published online by Cambridge University Press:
- 06 April 2021, A16
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Geometric phase methods with Stokes theorem for a general viscous swimmer
-
- Published online by Cambridge University Press:
- 06 April 2021, A17
-
- Article
- Export citation
An insulating plate drifting over a thermally convecting fluid: the effect of plate size on plate motion, coupling modes and flow structure
-
- Published online by Cambridge University Press:
- 06 April 2021, A18
-
- Article
- Export citation
Thermally-driven coalescence in thin liquid film flowing down a fibre
-
- Published online by Cambridge University Press:
- 06 April 2021, A19
-
- Article
- Export citation
Suppression of thermoacoustic instability by targeting the hubs of the turbulent networks in a bluff body stabilized combustor
-
- Published online by Cambridge University Press:
- 13 April 2021, A20
-
- Article
- Export citation
A fractional PDE model for turbulent velocity fields near solid walls
-
- Published online by Cambridge University Press:
- 12 April 2021, A21
-
- Article
- Export citation
Extended classification of the buoyancy-driven flows induced by a neutralization reaction in miscible fluids. Part 1. Experimental study
-
- Published online by Cambridge University Press:
- 12 April 2021, A22
-
- Article
- Export citation
Extended classification of the buoyancy-driven flows induced by a neutralization reaction in miscible fluids. Part 2. Theoretical study
-
- Published online by Cambridge University Press:
- 12 April 2021, A23
-
- Article
- Export citation
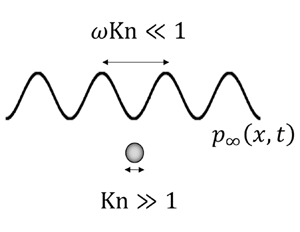
Acoustic levitation of a rigid nano-sphere at non-continuum conditions
-
- Published online by Cambridge University Press:
- 12 April 2021, A24
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Reynolds number effect on the response of a rough wall turbulent boundary layer to local wall suction
-
- Published online by Cambridge University Press:
- 12 April 2021, A25
-
- Article
- Export citation
Bridging the gap between particle-scale forces and continuum modelling of size segregation: application to bedload transport
-
- Published online by Cambridge University Press:
- 12 April 2021, A26
-
- Article
- Export citation
Modelling finger propagation in elasto-rigid channels
-
- Published online by Cambridge University Press:
- 12 April 2021, A27
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Melting driven by rotating Rayleigh–Bénard convection
-
- Published online by Cambridge University Press:
- 12 April 2021, A28
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
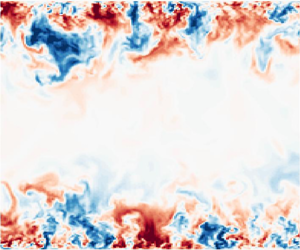
Direct numerical simulation of thermal channel flow for
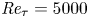 ${\textit {Re}}_\tau =5000$ and
${\textit {Re}}_\tau =5000$ and 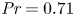 ${\textit {Pr}} = 0.71$
${\textit {Pr}} = 0.71$
-
- Published online by Cambridge University Press:
- 12 April 2021, A29
-
- Article
- Export citation
Removal of a dense bottom layer by a gravity current
-
- Published online by Cambridge University Press:
- 12 April 2021, A30
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Linear stability of shallow morphodynamic flows
-
- Published online by Cambridge University Press:
- 12 April 2021, A31
-
- Article
- Export citation
A rigorous bound on the scaling of dissipation with velocity amplitude in flow past a sphere
-
- Published online by Cambridge University Press:
- 12 April 2021, A32
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Lubricated gravity currents of power-law fluids
-
- Published online by Cambridge University Press:
- 12 April 2021, A33
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Falling jet of dry granular material in water
-
- Published online by Cambridge University Press:
- 14 April 2021, A34
-
- Article
- Export citation
An isolated logarithmic layer
-
- Published online by Cambridge University Press:
- 14 April 2021, A35
-
- Article
- Export citation