Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Utochkin, Vladimir Yu.
Siraev, Ramil R.
and
Bratsun, Dmitry A.
2021.
Pattern Formation in Miscible Rotating Hele-Shaw Flows Induced by a Neutralization Reaction.
Microgravity Science and Technology,
Vol. 33,
Issue. 6,
Mizev, A.I.
Mosheva, E.A.
and
Bratsun, D.A.
2021.
Extended classification of the buoyancy-driven flows induced by a neutralization reaction in miscible fluids. Part 1. Experimental study.
Journal of Fluid Mechanics,
Vol. 916,
Issue. ,
Utochkin, Vladimir Yu.
and
Bratsun, Dmitry A.
2022.
SPONTANEOUS ORDERING OF CHEMOCONVECTIVE FINGERING NEAR AN INTERFACE IN A REACTING SOLUTION
.
Interfacial Phenomena and Heat Transfer,
Vol. 10,
Issue. 4,
p.
1.
Bratsun, D. A.
Oschepkov, V. O.
Mosheva, E. A.
and
Siraev, R. R.
2022.
The effect of concentration-dependent diffusion on double-diffusive instability.
Physics of Fluids,
Vol. 34,
Issue. 3,
Zakharova, O.S.
Bratsun, D.A.
and
Ryzhkov, I.I.
2022.
Convective instability in multicomponent mixtures with Soret effect.
Computational Continuum Mechanics,
Vol. 15,
Issue. 1,
p.
67.
Zhang, Bo
Usta, Mustafa
Khan, Irfan
Ranjan, Devesh
and
Aidun, Cyrus K.
2022.
Chemically Reacting Turbulent Mixing in Coaxial Miscible Liquid Jets Under Variable Viscosities and Reaction Rates.
SSRN Electronic Journal ,
Denisova, Maria
and
Kostarev, Konstantin
2022.
Peculiarities of Mass Transfer Between the Droplet With a Chemical Reaction On Its Surface and the Surrounding Medium.
Microgravity Science and Technology,
Vol. 34,
Issue. 5,
Bratsun, Dmitry A.
Siraev, Ramil R.
Pismen, Len M.
Mosheva, Elena A.
Shmyrov, Andrey V.
and
Mizev, Alexey I.
2022.
Mixing Enhancement By Gravity-dependent Convection in a Y-shaped Continuous-flow Microreactor.
Microgravity Science and Technology,
Vol. 34,
Issue. 5,
Bratsun, Dmitry
Mizev, Alexey
Utochkin, Vladimir
Nekrasov, Svyatoslav
and
Shmyrova, Anastasia
2023.
Nonlinear development of convective patterns driven by a neutralization reaction in immiscible two-layer systems.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 381,
Issue. 2245,
Bratsun, D. A.
Siraev, R. R.
Mosheva, E. A.
Shmyrov, A. V.
and
Mizev, A. I.
2023.
Chemoconvective mixing in a Y-shaped continuous-flow microchannel.
Thermophysics and Aeromechanics,
Vol. 29,
Issue. 6,
p.
951.
Chen, Jin
Wang, Geng
Yang, Junyu
Lei, Timan
and
Luo, Kai H.
2023.
Pore-scale study of miscible density instability with viscosity contrast in porous media.
Physics of Fluids,
Vol. 35,
Issue. 9,
Maharana, Surya Narayan
Sahu, Kirti Chandra
and
Mishra, Manoranjan
2023.
Stability of a layered reactive channel flow.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 479,
Issue. 2271,
Mosheva, E.A.
Siraev, R.R.
and
Bratsun, D.A.
2023.
Chemoconvection of miscible solutions in an inclined layer.
Computational Continuum Mechanics,
Vol. 16,
Issue. 1,
p.
5.
Zhang, Bo
Usta, Mustafa
Khan, Irfan
Ranjan, Devesh
and
Aidun, Cyrus K.
2023.
Chemically reacting mixing in coaxial miscible liquid jets under variable viscosities and reaction rates.
Chemical Engineering Science,
Vol. 268,
Issue. ,
p.
118412.
Mosheva, Elena
Siraev, Ramil
and
Bratsun, Dmitry
2023.
Control of Chemoconvection in a Rectangular Slot by Changing Its Spatial Orientation.
Fluids,
Vol. 8,
Issue. 3,
p.
98.
Huang, Po-Wei
Flemisch, Bernd
Qin, Chao-Zhong
Saar, Martin O.
and
Ebigbo, Anozie
2023.
Validating the Nernst–Planck transport model under reaction-driven flow conditions using RetroPy v1.0.
Geoscientific Model Development,
Vol. 16,
Issue. 16,
p.
4767.
Kozlov, Nikolai
and
Mosheva, Elena
2023.
Investigation of chemoconvection in vibration fields.
Physical Chemistry Chemical Physics,
Vol. 25,
Issue. 12,
p.
8921.
Bratsun, D. A.
and
Utochkin, V. Yu.
2024.
Convection induced by centrifugal and Coriolis buoyancy in a rotating Hele-Shaw reactor.
Physics of Fluids,
Vol. 36,
Issue. 9,
Utochkin, Vladimir Yu.
Bratsun, Dmitry A.
and
Siraev, Ramil R.
2024.
ALIGNMENT OF FINGERING PATTERN INDUCED BY AN INTERFACIAL NEUTRALIZATION REACTION
.
Interfacial Phenomena and Heat Transfer,
Vol. 12,
Issue. 2,
p.
1.
Vailati, A.
Šeta, B.
Bou-Ali, M.M.
and
Shevtsova, V.
2024.
Perspective of research on diffusion: From microgravity to space exploration.
International Journal of Heat and Mass Transfer,
Vol. 229,
Issue. ,
p.
125705.
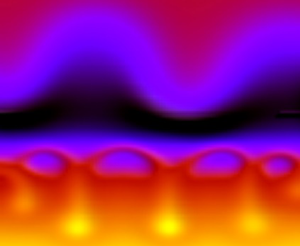
 $K_{\rho }$, the reaction-induced buoyancy number defined in Part 1. In this paper, the theoretical analysis complementing the experimental observations provides the conceptual insights required for a full understanding of the mechanisms of the observed phenomena. The mathematical model we develop consists of a system of reaction–diffusion–advection equations governing the evolution of concentrations coupled to the Navier–Stokes equation. The system dynamics is examined through transient linear stability analysis and numerical simulation. If
$K_{\rho }$, the reaction-induced buoyancy number defined in Part 1. In this paper, the theoretical analysis complementing the experimental observations provides the conceptual insights required for a full understanding of the mechanisms of the observed phenomena. The mathematical model we develop consists of a system of reaction–diffusion–advection equations governing the evolution of concentrations coupled to the Navier–Stokes equation. The system dynamics is examined through transient linear stability analysis and numerical simulation. If  $K_{\rho }>1$, then a statically stable potential well appears adjacent to the reaction front. As a result, a Rayleigh–Bénard-like cellular pattern can arise in this depleted density region. If
$K_{\rho }>1$, then a statically stable potential well appears adjacent to the reaction front. As a result, a Rayleigh–Bénard-like cellular pattern can arise in this depleted density region. If  $K_{\rho }\leqslant 1$, then a potential well collapses, and a shock-wave-like structure with an almost planar front occurs. This wave propagates fast compared with the diffusion time and acts as a turbulent bore separating immobile fluid and an area of intense convective mixing. Finally, we determine the place of the above instabilities in an extended classification of known instability types.
$K_{\rho }\leqslant 1$, then a potential well collapses, and a shock-wave-like structure with an almost planar front occurs. This wave propagates fast compared with the diffusion time and acts as a turbulent bore separating immobile fluid and an area of intense convective mixing. Finally, we determine the place of the above instabilities in an extended classification of known instability types.

