 $\boldsymbol{\geq 3}$
$\boldsymbol{\geq 3}$
 $K3^{[n]}$ type are algebraic
$K3^{[n]}$ type are algebraic
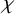 $\chi $-dependence of ring structure for the moduli of one-dimensional sheaves on
$\chi $-dependence of ring structure for the moduli of one-dimensional sheaves on 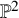 $\mathbb {P}^2$
$\mathbb {P}^2$
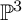 $\mathbb {P}^3$
$\mathbb {P}^3$
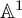 ${\mathbb A}^1$-CONNECTEDNESS OF MODULI OF VECTOR BUNDLES ON A CURVE
${\mathbb A}^1$-CONNECTEDNESS OF MODULI OF VECTOR BUNDLES ON A CURVE
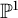 $\mathbb {P}^1$
$\mathbb {P}^1$