The present work studies numerically the quasi-steady propagation of a hydrogen/oxygen detonation in a supersonic model combustor consisting of a cavity and an expanding wall. The two-dimensional reactive compressible Navier–Stokes equations with a one-step and two-species reaction model are solved using a hybrid sixth-order weighted essentially non-oscillatory-centred difference scheme combined with a structured adaptive mesh refinement technique. The results show that, after the shutdown of the hot jet, the detonation wave is successfully stabilized quasi-steadily in the supersonic model combustor together with periodic fluctuations of the detonation front. The formation of the quasi-steady propagation of detonation in the model combustor is mainly due to the combined effects of (i) pressure oscillations generated in the cavity, which facilitate the detonation propagation, and (ii) lateral mass divergence brought by the expanding wall, which can lead to detonation attenuation, and an unburned jet associated with large-scale vortices resulting from a Prandtl–Meyer expansion fan. This expansion fan is generated because of the expanding wall, which can contribute to the detonation stabilization. It is found that, for an incoming velocity lower than the Chapman–Jouguet value, a quasi-steady propagation of the detonation wave cannot be achieved. However, for incoming velocity higher than the Chapman–Jouguet value, a stabilization can be realized. This is effectively due to the formation of a periodic process, including four stages of forward propagation, detonation attenuation, backward propagation and detonation bifurcation, indicating the influence of the supersonic model combustor on the overall process.

The flow in a Hele-Shaw cell with a time-increasing gap poses a unique shrinking interface problem. When the upper plate of the cell is lifted perpendicularly at a prescribed speed, the exterior less viscous fluid penetrates the interior more viscous fluid, which generates complex, time-dependent interfacial patterns through the Saffman–Taylor instability. The pattern formation process sensitively depends on the lifting speed and is still not fully understood. For some lifting speeds, such as linear or exponential speed, the instability is transient and the interface eventually shrinks as a circle. However, linear stability analysis suggests there exist shape invariant shrinking patterns if the gap $b(t)$ is increased more rapidly: $b(t)=\left (1-({7}/{2})\tau \mathcal {C} t\right )^{-{2}/{7}}$, where $\tau$ is the surface tension and $\mathcal {C}$ is a function of the interface perturbation mode $k$. Here, we use a spectrally accurate boundary integral method together with an efficient time adaptive rescaling scheme, which for the first time makes it possible to explore the nonlinear limiting dynamical behaviour of a vanishing interface. When the gap is increased at a constant rate, our numerical results quantitatively agree with experimental observations (Nase et al., Phys. Fluids, vol. 23, 2011, 123101). When we use the shape invariant gap $b(t)$, our nonlinear results reveal the existence of $k$-fold dominant, one-dimensional, web-like networks, where the fractal dimension is reduced to almost unity at late times. We conclude by constructing a morphology diagram for pattern selection that relates the dominant mode $k$ of the vanishing interface and the control parameter $\mathcal {C}$.

Continuously forced, stratified shear flows occur in many geophysical systems, including flow over sills, through fjords and at the mouths of rivers and estuaries. These continuously forced shear flows can be unstable and drive turbulence, which can enhance the rate of mixing. In this study, we analyse three-dimensional direct numerical simulations of an idealized stratified shear flow that is continuously forced by weakly relaxing both the buoyancy and streamwise velocity towards prescribed mean profiles. We explore a range of large and small Richardson numbers, for constant Reynolds and Prandtl numbers (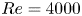${Re}=4000$ and ${Pr}=1$). After a turbulent steady state develops, three regimes are observed: (i) a weakly stratified, overturning regime, (ii) a strongly stratified, scouring regime and (iii) an intermediately stratified, intermittent regime. The overturning regime exhibits partially formed overturning billows that break down into turbulence and broaden the velocity and buoyancy interfaces. Conversely, the scouring regime exhibits internal gravity waves propagating along the strongly stratified buoyancy interface, while turbulence on either side of the buoyancy interface reinforces the stratification. The intermediate regime quasi-periodically alternates between behaviours associated with the overturning and scouring regimes. For each case, we quantify an appropriate measure of the efficiency of mixing and examine its dependence on relevant parameters including appropriate definitions of the buoyancy Reynolds number, gradient Richardson number and horizontal Froude numbers. Using a framework involving sorted buoyancy coordinates as introduced by Nakamura (J. Atmos. Sci., vol. 53, 1996, pp. 1524–1537) and Winters & D'Asaro (J. Fluid Mech., vol. 317, 1996, pp. 179–193), we examine the underlying physical mechanisms leading to broadening and thinning of the buoyancy interface.

The propulsive performance of a flexible foil with prescribed pitching and heaving motions about any pivot point location and passive chordwise flexural deflection is analysed within the framework of the linear potential flow theory and the Euler–Bernoulli beam equation using a quartic approximation for the deflection. The amplitude of the flexural component of the deflection and its phase, the thrust force, input power and propulsive efficiency are computed analytically in terms of the stiffness and mass ratio of the plate, frequency, pivot point location and remaining kinematic parameters. It is found that the maximum flexural deflection amplitude, thrust and input power are related to the first fluid–structure natural frequency of the system, corresponding to the deflection approximation considered. The same relation is observed for the propulsive efficiency when an offset drag is included in the analytical expressions. These results, which are valid for small amplitude and sufficiently large stiffness of the foil, are compared favourably with previous related results when the foil pivots about the leading edge. The configurations generating maximum thrust and efficiency enhancement by flexibility are analysed in relation to those of an otherwise identical rigid foil.

The post-injection migration of a plume of CO$_{2}$ through an inclined, confined porous layer across which the permeability varies is studied theoretically. We derive a sharp-interface lubrication model which accounts for the capillary trapping of CO$_{2}$ at the receding edge of the plume. Eventually the CO$_{2}$ becomes entirely trapped in the pore throats, and the final run-out distance is a key quantity for determining storage security and efficiency. We deploy asymptotic approximations to show that when the CO$_{2}$ plume migrates a long distance relative to its initial length, the run-out distance is approximately proportional to the permeability at the top of the layer. The permeability structure away from the top boundary is important at early and intermediate times. Dissolution of the CO$_{2}$ and three-dimensional effects are incorporated, which demonstrate that the influence of heterogeneity is quite general. The initial distribution of the CO$_{2}$ at the end of the injection phase also influences the post-injection spreading and trapping. At low injection rates, the CO$_{2}$ remains near the top of the layer so that the end-of-injection plume shape has a small aspect ratio leading to a relatively large run-out length. At very high fluxes, the end-of-injection shape and hence the final run-out distance becomes nearly independent of the injection flux because the role of buoyancy becomes negligible during injection. Our results illustrate the strong control of reservoir heterogeneity on the sweep efficiency of a CO$_{2}$ plume and hence the storage capacity. In many situations, it may be possible to increase the storage volume by injecting at a higher rate.

One of the key observations in the Princeton Superpipe was the late start of the logarithmic mean velocity overlap layer at a wall distance of the order of $10^3$ inner units. Between $y^+\approx 150$, the start of the overlap layer in zero pressure gradient turbulent boundary layers, and $y^+\approx 500$, the Superpipe profile is modelled equally well by a power law or a log law with a larger slope than in the overlap layer. This paper demonstrates, that the asymptotic mean velocity profile in turbulent plane channel flow exhibits analogous characteristics, namely a rather sudden decrease of logarithmic slope (increase of $\kappa$) at a $y^+$ of approximately $600$, which marks the start of the actual overlap layer. The demonstration results from the first construction of the complete mean velocity inner and outer asymptotic expansions up to order ${O}({Re}^{-1}_{\tau })$ from direct numerical simulations (DNS) at moderate Reynolds numbers. The ${O}({Re}^{-1}_{\tau })$ contribution to the indicator function $\varXi ^+ = y^+(\textrm {d} U^+/ \textrm {d} y^+)$ is found to be important and to prevent the direct determination of $\kappa$ from currently available channel DNS. A preliminary, leading-order analysis of a Couette flow DNS, on the other hand, yields an increase of logarithmic slope (decrease of $\kappa$) at a $y^+_{{break}}\approx 400$. The correlation between the sign of the slope change and the flow symmetry motivates the hypothesis that the breakpoint between the possibly universal short inner logarithmic region and the actual overlap log-law corresponds to the penetration depth of large-scale turbulent structures originating from the opposite wall.

Numerous experiments and theoretical calculations have shown that cylindrical vesicles can undergo a pearling instability similar to the Rayleigh–Plateau instability of a liquid jet when they are subjected to external tension. In a living cell, a Rayleigh–Plateau-like instability could be triggered by internal tension generated in the cell cortex. This mechanism has been suggested to play an essential role in biological processes such as cell morphogenesis. In contrast to the simple, passive and isotropic membrane of vesicles, the cortical tensions generated by biological cells are often strongly anisotropic. Here, we theoretically investigate how this anisotropy affects the Rayleigh–Plateau instability mechanism. We do so in the limit of both low and high Reynolds numbers and accordingly cover cell behaviour under anisotropic cortical tension as well as fast liquid jets with anisotropic surface tension. Combining analytical linear stability analysis with numerical simulations we report a strong influence of the anisotropy on the dominant wavelength of the instability: increasing azimuthal with respect to axial tension leads to destabilisation and to a shorter break-up wavelength. In addition, compared to the classical isotropic Rayleigh–Plateau situation, the range of unstable modes grows or shrinks when the azimuthal tension is higher or lower than the axial tension, respectively. We explore nonlinear effects like an altered break-up time and formation of satellite droplets under anisotropic tension. In Part 2 (Bächer et al. J. Fluid Mech., vol. xxx, 2021, Ax) of this series we continue our analysis by analytically investigating the influence of bending and shear elasticity, usually present in vesicles and cells, on this anisotropic Rayleigh–Plateau instability.

Cylindrical vesicle and cell membranes under tension can undergo a Rayleigh–Plateau instability leading to break-up. In Part 1 (Graessel et al., J. Fluid Mech., vol. xxx, 2021, Ax) we showed that anisotropic tension, created by active biological processes underneath the cell membrane, can significantly influence this process for a liquid–liquid interface. Here, we study the combined influence of anisotropic tension and membrane elasticity on the Rayleigh–Plateau instability. We analytically derive the dispersion relation for an interface endowed with bending and/or shear elasticity considering explicitly the dynamics of the suspending fluid. We find that the combination of bending elasticity and tension anisotropy leads to three qualitatively different regimes for the Rayleigh–Plateau scenario: (i) the classical regime in which short wavelengths are stable and long wavelengths are unstable, (ii) the suppressed regime in which the system is stable against all perturbation wavelengths and (iii) the restricted regime, in which a stable region at short and another one at long wavelengths are separated by a range of unstable modes centred around the dimensionless wavenumber $kR_0=1$. The width of this unstable range as well as the dominant wavelength of the instability depend on the bending modulus and tension anisotropy. For shear elasticity and area dilatation, on the other hand, only the classical and the suppressed regimes are observed, with the transition between them being independent of the tension anisotropy.

We experimentally investigate the surface drag characteristics of a staggered-distributed cube array and its interaction with the turbulent structure of the overlying flow. Instantaneous maps of the pressure field, inferred from in-plane velocity data are used to estimate the forces acting on a target roughness element. Coupled statistics of the force in combination with conditional flow analysis and extended proper orthogonal decomposition (POD) of the pressure field, based on the velocity POD modes, elucidate the relevant mechanisms responsible for surface drag generation. The results show that turbulent motions, at different scales, leave an imprint on the pressure field. Specifically, positive and negative fluctuations are generally associated with flow regions experiencing a local deceleration and acceleration, respectively. Although large-scale motions were found to be the single greatest contributor to the fluctuating pressure field, their direct influence on the surface drag fluctuations appears to be mitigated by the relative size of the considerably smaller roughness obstacles. We hypothesise that a pressure wave induced by the passage of alternating high- and low-momentum regions evenly affects the flow field over a broad region, coupling the forces on the windward and leeward sides of the cube, which, in turn, partially cancel each other out. Uncorrelated, intermediate and small-scale pressure events are thus more important to the overall drag fluctuations. While the direct influence of the large-scale structures on the surface drag may be smaller than expected, the results suggest that they are still significant for the role they play in modulating the small-scale pressure events in the canopy region.

We theoretically and experimentally investigate the mechanism underlying the generation of upstream-propagating waves induced by a steady current over a horizontal bottom with a patch of sinusoidal ripples. By considering the triad resonant wave–ripple interactions involving two unsteady wave components (which have the same frequency but different wavenumbers) and one bottom ripple component in the presence of a steady uniform current, we derive the general condition under which unsteady upstream- and/or downstream-propagating waves can be induced. The frequency and wavenumbers of the induced propagating waves are given by the triad resonance condition in terms of current speed, water depth and bottom ripple wavenumber. By means of a multiple-scale perturbation analysis, we obtain the nonlinear amplitude evolution equations governing the spatio-temporal evolution of resonance-generated waves. Based on these equations, we find that the amplitude of the generated upstream-propagating waves is dramatically amplified when the associated triad resonance occurs in the neighbourhood of the critical current speed/frequency (corresponding to zero group velocity of unsteady waves in the presence of a current). A series of laboratory experiments in a long wave flume with wide ranges of current speeds and water depths are conducted to verify the theory. The experiments confirm the observation of the phenomenon of upstream-propagating wave generation in a steady flow over a rippled bottom. In particular, the experimental measurements of the kinematics of upstream-propagating waves as well as the critical flow condition for the observation of such wave generation compare well with the theoretical prediction.

The Hasselmann kinetic equation (HKE) forms the cornerstone of present-day spectral wave models. It describes the redistribution of energy over the wave spectrum as a result of resonant four-wave interactions, and theoretically prescribes wave evolution on a slow $O(1/\varepsilon ^4\omega _0)$ time scale, where $\varepsilon$ and $\omega _0$ are typical wave steepness and frequency. Alternatives to the HKE (e.g. the generalized kinetic equation (GKE)), including the effects of non-resonant four-wave interactions, are believed capable of evolving wave fields on a fast $O(1/\varepsilon ^2\omega _0)$ time scale. It is beyond doubt that these alternatives could reasonably predict changes of unidirectional waves whereas the HKE cannot. For angular spread wave fields, however, it is still ambiguous whether the GKE behaves remarkably differently from the HKE because previous research in this direction was not fully consistent. In this study, we revised the GKE algorithm implemented in the spectral wave model WAVEWATCH III (WW3) by correcting two numerical aspects related to the discretization of the GKE and to the source term integration. It is proved that once updated, the GKE in WW3 does not give rise to significant deviation from the HKE-based results, provided that the wave spectra are fairly smooth and the directionality is sufficiently broad. These results, although unexpected, are in good agreement with findings reported by Annenkov & Shrira. More strikingly, the HKE and GKE are observed to operate at the same fast $O(1/\varepsilon ^2\omega _0)$ time scale for the spectral peak downshift and angular broadening, indicating that the HKE, solved by the well-established Webb–Resio–Tracy algorithm, seems more robust than usually expected.

The finite-amplitude space–time mean flows that are precessionally forced in rotating finite circular cylinders are examined. The findings show that, in addition to conventional Reynolds-stress-type source terms for streaming in oscillatory forced flows, a set of Coriolis-type source terms due the background rotation also contribute. These terms result from the interaction between the equatorial component of the total rotation vector and the overturning flow that is forced by the precession, both of which have azimuthal wavenumbers $m={\pm }1$. The interaction is particular to precessing flows and does not exist in rotating flows driven by libration ($m=0$ forcing) or tides ($m={\pm }2$ forcing). By examining typical example flows in the quasi-linear weakly forced streaming regime, we are able to consider the contributions from the Reynolds-stress terms and the equatorial-Coriolis terms separately, and find that they are of similar magnitude. In the cases examined, the azimuthal component of streaming flow driven by the equatorial-Coriolis terms is everywhere retrograde, whereas that driven by Reynolds stresses may have both retrograde and prograde regions, but the total streaming flows are everywhere retrograde. Even when the forcing frequency is larger than twice the background rotation rate, we find that there is a streaming flow driven by both the Reynolds-stress and the equatorial-Coriolis terms. For cases forced at precession frequencies in near resonance with the eigenfrequencies of the intrinsic inertial modes of the linear inviscid unforced rotating cylinder flow, we quantify theoretically how the amplitude of streaming flow scales with respect to variations in Reynolds number, cylinder tilt angle and aspect ratio, and compare these with numerical simulations.

We present an Euler–Lagrange approach for simulating magneto-Archimedes separation of almost neutrally buoyant spherical particles in the flow of a paramagnetic liquid, which is of direct relevance for separating different types of plastic by magnetic density separation. A four-way coupled point-particle method is employed where all relevant interactions between an external magnetic field, a magnetic fluid and discrete immersed particles are taken into account. Particle–particle interaction is modelled by a hard-sphere collision model which takes the interstitial fluid effects into account. First, the motion of rigid spherical particles in a paramagnetic liquid is studied in single- and two-particle systems. We find good agreements between our numerical results and experiments performed in a paramagnetic liquid exposed to a non-homogeneous magnetic field, also in the case of two colliding particles. Next, we investigate the magneto-Archimedes separation of particles with different mass densities in many-particle systems interacting with the fluid. Our results reveal that history effects and interparticle interactions significantly influence the levitation dynamics of particles and have a detrimental impact on the separation performance. We also investigate the effect of particle size and initial distribution on the separation performance. Results show that a reduction in the particle size from 4 to 2 mm leads to a $40\,\%$ increase in the separation time. Moreover, preseparation of particles into two groups of light and heavy particles decreases the separation time by $33\,\%$. The presented method is shown to be a robust and efficient computational framework for the investigation of particle-laden flows of magnetically responsive fluids.

We study the role of hydrodynamic instabilities in the morphogenesis of some typical karst draperies structures encountered in limestone caves. The problem is tackled using the long wave approximation for the fluid film that flows under an inclined substrate, in the presence of substrate variations that grow according to a deposition law. We numerically study the linear and nonlinear evolution of a localized initial perturbation both in the fluid film and on the substrate, i.e. the Green function. A novel approach for the spatio-temporal analysis of two-dimensional signals resulting from linear simulations is introduced, based on the concepts of the Riesz transform and the monogenic signal, the multidimensional complex continuation of a real signal. This method allows for a deeper understanding of the pattern formation. The linear evolution of an initial localized perturbation in the presence of deposition is studied. The deposition linearly selects substrate structures aligned along the streamwise direction, as the spatio-temporal response is advected away. Furthermore, the growth of the initial defect produces a quasi-steady region also characterized by streamwise structures both on the substrate and the fluid film, which is in good agreement with the Green function for a steady defect on the substrate, in the absence of deposition.