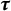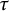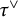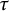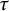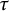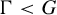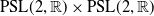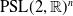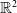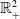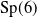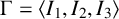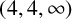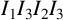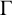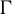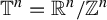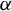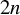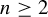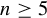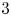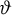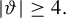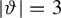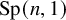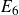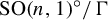62 results
Rich representations and superrigidity
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems , First View
- Published online by Cambridge University Press:
- 27 January 2025, pp. 1-24
-
- Article
- Export citation
On infinitesimal
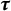 $\boldsymbol { \tau }$-isospectrality of locally symmetric spaces
$\boldsymbol { \tau }$-isospectrality of locally symmetric spaces
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 68 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 09 January 2025, pp. 246-261
- Print publication:
- March 2025
-
- Article
- Export citation
Hyperbolic actions of higher rank lattices come from rank-one factors
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 45 / Issue 4 / April 2025
- Published online by Cambridge University Press:
- 30 September 2024, pp. 961-988
- Print publication:
- April 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Weyl chamber length compactification of the
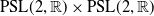 $\textrm{PSL}(2,{\mathbb{R}})\times \textrm{PSL}(2,{\mathbb{R}})$ maximal character variety
$\textrm{PSL}(2,{\mathbb{R}})\times \textrm{PSL}(2,{\mathbb{R}})$ maximal character variety
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 67 / Issue 1 / January 2025
- Published online by Cambridge University Press:
- 27 September 2024, pp. 11-33
- Print publication:
- January 2025
-
- Article
-
- You have access
- HTML
- Export citation
Non-concentration property of Patterson–Sullivan measures for Anosov subgroups
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 45 / Issue 3 / March 2025
- Published online by Cambridge University Press:
- 18 September 2024, pp. 884-893
- Print publication:
- March 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Thinness of some hypergeometric groups in
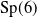 $\mathrm{Sp}(6)$
$\mathrm{Sp}(6)$
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 66 / Issue 3 / September 2024
- Published online by Cambridge University Press:
- 02 May 2024, pp. 571-581
- Print publication:
- September 2024
-
- Article
-
- You have access
- HTML
- Export citation
DIOPHANTINE TRANSFERENCE PRINCIPLE OVER FUNCTION FIELDS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 110 / Issue 2 / October 2024
- Published online by Cambridge University Press:
- 28 February 2024, pp. 216-233
- Print publication:
- October 2024
-
- Article
-
- You have access
- HTML
- Export citation
On denseness of horospheres in higher rank homogeneous spaces
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 44 / Issue 11 / November 2024
- Published online by Cambridge University Press:
- 19 February 2024, pp. 3272-3289
- Print publication:
- November 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
ON THE LIMIT SET OF A COMPLEX HYPERBOLIC TRIANGLE GROUP
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 110 / Issue 2 / October 2024
- Published online by Cambridge University Press:
- 02 February 2024, pp. 338-345
- Print publication:
- October 2024
-
- Article
-
- You have access
- HTML
- Export citation
Bounded cohomology is not a profinite invariant
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 67 / Issue 2 / June 2024
- Published online by Cambridge University Press:
- 20 October 2023, pp. 379-390
- Print publication:
- June 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Cusps, Kleinian groups, and Eisenstein series
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 11 / 2023
- Published online by Cambridge University Press:
- 31 August 2023, e75
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A presentation for the Eisenstein-Picard modular group in three complex dimensions
- Part of
-
- Journal:
- Glasgow Mathematical Journal / Volume 65 / Issue 3 / September 2023
- Published online by Cambridge University Press:
- 25 July 2023, pp. 625-654
- Print publication:
- September 2023
-
- Article
- Export citation
ARITHMETICITY OF
 $\mathbb {C}$-FUCHSIAN SUBGROUPS OF SOME NONARITHMETIC LATTICES
$\mathbb {C}$-FUCHSIAN SUBGROUPS OF SOME NONARITHMETIC LATTICES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 109 / Issue 1 / February 2024
- Published online by Cambridge University Press:
- 28 April 2023, pp. 77-88
- Print publication:
- February 2024
-
- Article
-
- You have access
- HTML
- Export citation
Global rigidity of higher rank lattice actions with dominated splitting
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 44 / Issue 3 / March 2024
- Published online by Cambridge University Press:
- 27 April 2023, pp. 799-828
- Print publication:
- March 2024
-
- Article
-
- You have access
- HTML
- Export citation
Isolations of geodesic planes in the frame bundle of a hyperbolic 3-manifold
- Part of
-
- Journal:
- Compositio Mathematica / Volume 159 / Issue 3 / March 2023
- Published online by Cambridge University Press:
- 28 February 2023, pp. 488-529
- Print publication:
- March 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A report on an ergodic dichotomy
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 44 / Issue 1 / January 2024
- Published online by Cambridge University Press:
- 27 February 2023, pp. 236-289
- Print publication:
- January 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
STRUCTURAL STABILITY OF MEANDERING-HYPERBOLIC GROUP ACTIONS
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 23 / Issue 2 / March 2024
- Published online by Cambridge University Press:
- 27 December 2022, pp. 753-810
- Print publication:
- March 2024
-
- Article
-
- You have access
- HTML
- Export citation
Quaternionic hyperbolic lattices of minimal covolume
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 10 / 2022
- Published online by Cambridge University Press:
- 25 August 2022, e68
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the profinite rigidity of lattices in higher rank Lie groups
- Part of
-
- Journal:
- Mathematical Proceedings of the Cambridge Philosophical Society / Volume 174 / Issue 2 / March 2023
- Published online by Cambridge University Press:
- 22 August 2022, pp. 369-384
- Print publication:
- March 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Effective equidistribution of horospherical flows in infinite volume rank-one homogeneous spaces
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 43 / Issue 8 / August 2023
- Published online by Cambridge University Press:
- 09 August 2022, pp. 2780-2840
- Print publication:
- August 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation