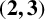 $\boldsymbol {(2,3)}$-GENERATION OF THE FINITE SIMPLE ODD-DIMENSIONAL ORTHOGONAL GROUPS
$\boldsymbol {(2,3)}$-GENERATION OF THE FINITE SIMPLE ODD-DIMENSIONAL ORTHOGONAL GROUPS
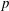 $p$-subgroups of finite Chevalley groups of rank six and seven
$p$-subgroups of finite Chevalley groups of rank six and seven
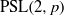 $\mathrm{PSL} (2, p)$ of size four
$\mathrm{PSL} (2, p)$ of size four