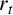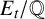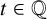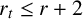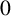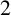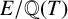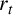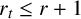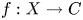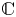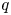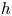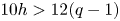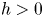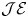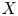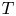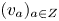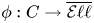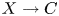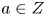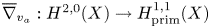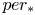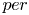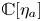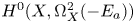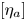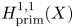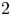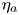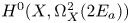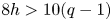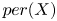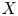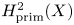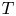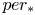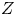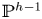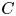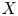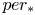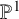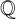Let E/\mathbb {Q}(T)$E/\mathbb {Q}(T)$ be a nonisotrivial elliptic curve of rank r. A theorem due to Silverman [‘Heights and the specialization map for families of abelian varieties’, J. reine angew. Math. 342 (1983), 197–211] implies that the rank r_t$r_t$ of the specialisation E_t/\mathbb {Q}$E_t/\mathbb {Q}$ is at least r for all but finitely many t \in \mathbb {Q}$t \in \mathbb {Q}$. Moreover, it is conjectured that r_t \leq r+2$r_t \leq r+2$, except for a set of density 0$0$. When E/\mathbb {Q}(T)$E/\mathbb {Q}(T)$ has a torsion point of order 2$2$, under an assumption on the discriminant of a Weierstrass equation for E/\mathbb {Q}(T)$E/\mathbb {Q}(T)$, we produce an upper bound for r_t$r_t$ that is valid for infinitely many t. We also present two examples of nonisotrivial elliptic curves E/\mathbb {Q}(T)$E/\mathbb {Q}(T)$ such that r_t \leq r+1$r_t \leq r+1$ for infinitely many t.

Suppose that 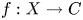f:X\to C$f:X\to C$ is a general Jacobian elliptic surface over 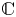{\mathbb {C}}${\mathbb {C}}$ of irregularity 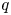q$q$ and positive geometric genus 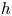h$h$. Assume that 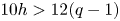10 h>12(q-1)$10 h>12(q-1)$, that 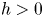h>0$h>0$ and let \overline {\mathcal {E}\ell \ell }$\overline {\mathcal {E}\ell \ell }$ denote the stack of generalized elliptic curves. (1) The moduli stack 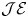\mathcal {JE}$\mathcal {JE}$ of such surfaces is smooth at the point 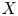X$X$ and its tangent space 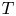T$T$ there is naturally a direct sum of lines 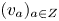(v_a)_{a\in Z}$(v_a)_{a\in Z}$, where Z\subset C$Z\subset C$ is the ramification locus of the classifying morphism 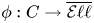\phi :C\to \overline {\mathcal {E}\ell \ell }$\phi :C\to \overline {\mathcal {E}\ell \ell }$ that corresponds to 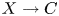X\to C$X\to C$. (2) For each 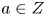a\in Z$a\in Z$ the map 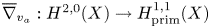\overline {\nabla }_{v_a}:H^{2,0}(X)\to H^{1,1}_{\rm prim}(X)$\overline {\nabla }_{v_a}:H^{2,0}(X)\to H^{1,1}_{\rm prim}(X)$ defined by the derivative 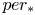per_*$per_*$ of the period map 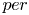per$per$ is of rank one. Its image is a line 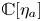{\mathbb {C}}[\eta _a]${\mathbb {C}}[\eta _a]$ and its kernel is 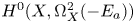H^0(X,\Omega ^2_X(-E_a))$H^0(X,\Omega ^2_X(-E_a))$, where E_a=f^{-1}(a)$E_a=f^{-1}(a)$. (3) The classes 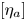[\eta _a]$[\eta _a]$ form an orthogonal basis of 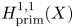H^{1,1}_{\rm prim}(X)$H^{1,1}_{\rm prim}(X)$ and [\eta _a]$[\eta _a]$ is represented by a meromorphic 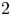2$2$-form 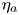\eta _a$\eta _a$ in 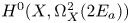H^0(X,\Omega ^2_X(2E_a))$H^0(X,\Omega ^2_X(2E_a))$ of the second kind. (4) We prove a local Schottky theorem; that is, we give a description of per_*$per_*$ in terms of a certain additional structure on the vector bundles that are involved. Assume further that 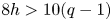8h>10(q-1)$8h>10(q-1)$ and that h\ge q+3$h\ge q+3$. (5) Given the period point 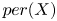per(X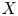)$per(X)$ of X$X$ that classifies the Hodge structure on the primitive cohomology 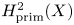H^2_{\rm prim}(X)$H^2_{\rm prim}(X)$ and the image of 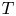T$T$ under 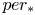per_*$per_*$ we recover 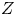Z$Z$ as a subset of 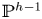{\mathbb {P}}^{h-1}${\mathbb {P}}^{h-1}$ and then, by quadratic interpolation, the curve 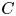C$C$. (6) We prove a generic Torelli theorem for these surfaces. Everything relies on the construction, via certain kinds of Schiffer variations of curves, of certain variations of 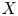X$X$ for which 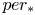per_*$per_*$ can be calculated. (In an earlier version of this paper we used variations constructed by Fay. However, Schiffer variations are slightly more powerful.)

For a nonconstant elliptic surface over \mathbb {P}^1$\mathbb {P}^1$ defined over \mathbb {Q}$\mathbb {Q}$, it is a result of Silverman [‘Heights and the specialization map for families of abelian varieties’, J. reine angew. Math. 342 (1983), 197–211] that the Mordell–Weil rank of the fibres is at least the rank of the group of sections, up to finitely many fibres. If the elliptic surface is nonisotrivial, one expects that this bound is an equality for infinitely many fibres, although no example is known unconditionally. Under the Bunyakovsky conjecture, such an example has been constructed by Neumann [‘Elliptische Kurven mit vorgeschriebenem Reduktionsverhalten. I’, Math. Nachr. 49 (1971), 107–123] and Setzer [‘Elliptic curves of prime conductor’, J. Lond. Math. Soc. (2) 10 (1975), 367–378]. In this note, we show that the Legendre elliptic surface has the desired property, conditional on the existence of infinitely many Mersenne primes.

We describe a method to show that certain elliptic surfaces do not admit purely inseparable multisections (equivalently, that genus 1 curves over function fields admit no points over the perfect closure of the base field) and use it to show that any non-Jacobian elliptic structure on a very general supersingular K3 surface has no purely inseparable multisections. We also describe specific examples of genus 1 fibrations on supersingular K3 surfaces without purely inseparable multisections.

Monodromy groups, i.e. the groups of isometries of the intersection lattice LX [colone] H2/torsion generated by the monodromy action of all deformation families of a given surface, have been computed by the author for any minimal elliptic surface with pg > q = 0. New and refined methods are now employed to address the cases of minimal elliptic surfaces with pg [ges ] q > 0. Thereby we get explicit families such that any isometry is in the group generated by their monodromies or does not respect the invariance of the canonical class or the spinor norm. The monodromy is also shown to act by the full symplectic group on the first homology modulo torsion.