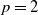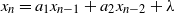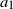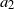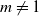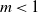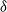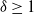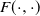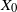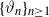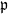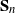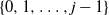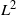273 results
Hitting times on the lollipop graph
-
- Journal:
- Probability in the Engineering and Informational Sciences , First View
- Published online by Cambridge University Press:
- 07 March 2025, pp. 1-34
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
A non-convex optimization approach of searching algebraic degree phase-type representations for general phase-type distributions
- Part of
-
- Journal:
- Advances in Applied Probability , First View
- Published online by Cambridge University Press:
- 03 March 2025, pp. 1-37
-
- Article
- Export citation
An Asymptotic Likelihood Ratio Test for a Markov Chain with Bernoullian or Contingent Input
-
- Journal:
- Psychometrika / Volume 33 / Issue 4 / December 1968
- Published online by Cambridge University Press:
- 01 January 2025, pp. 405-422
-
- Article
- Export citation
The Markov chain embedding problem in a one-jump setting
- Part of
-
- Journal:
- Journal of Applied Probability , First View
- Published online by Cambridge University Press:
- 09 December 2024, pp. 1-23
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
QUASI-ERGODICITY OF COMPACT STRONG FELLER SEMIGROUPS ON L^2
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 24 / Issue 2 / March 2025
- Published online by Cambridge University Press:
- 14 November 2024, pp. 541-584
- Print publication:
- March 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Efficiency of reversible MCMC methods: elementary derivations and applications to composite methods
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 62 / Issue 1 / March 2025
- Published online by Cambridge University Press:
- 18 September 2024, pp. 188-208
- Print publication:
- March 2025
-
- Article
-
- You have access
- HTML
- Export citation
1 - Topics in representation theory of finite groups
-
-
- Book:
- Groups and Graphs, Designs and Dynamics
- Published online:
- 11 May 2024
- Print publication:
- 30 May 2024, pp 1-86
-
- Chapter
- Export citation
29 - Coupled Systems and Diagonalisation
-
- Book:
- Mathematics for Economics and Finance
- Published online:
- 24 May 2024
- Print publication:
- 30 May 2024, pp 334-346
-
- Chapter
- Export citation
(Almost) complete characterization of the stability of a discrete-time Hawkes process with inhibition and memory of length two
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 4 / December 2024
- Published online by Cambridge University Press:
- 24 May 2024, pp. 1463-1484
- Print publication:
- December 2024
-
- Article
-
- You have access
- HTML
- Export citation
3 - Markov Chains
- from Part I - Tools and Theory
-
- Book:
- Harmonic Functions and Random Walks on Groups
- Published online:
- 16 May 2024
- Print publication:
- 23 May 2024, pp 75-116
-
- Chapter
- Export citation
Extreme heat wave sampling and prediction with analog Markov chain and comparisons with deep learning
-
- Journal:
- Environmental Data Science / Volume 3 / 2024
- Published online by Cambridge University Press:
- 27 March 2024, e9
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
On the Kolmogorov constant explicit form in the theory of discrete-time stochastic branching systems
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 61 / Issue 3 / September 2024
- Published online by Cambridge University Press:
- 04 January 2024, pp. 927-941
- Print publication:
- September 2024
-
- Article
-
- You have access
- HTML
- Export citation
Government spending multipliers with the Real Cost channel
-
- Journal:
- Macroeconomic Dynamics / Volume 28 / Issue 4 / June 2024
- Published online by Cambridge University Press:
- 06 July 2023, pp. 791-812
-
- Article
-
- You have access
- HTML
- Export citation
Distributions of random variables involved in discrete censored δ-shock models
- Part of
-
- Journal:
- Advances in Applied Probability / Volume 55 / Issue 4 / December 2023
- Published online by Cambridge University Press:
- 19 May 2023, pp. 1144-1170
- Print publication:
- December 2023
-
- Article
- Export citation
Synchronization of coupled map lattices
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 66 / Issue 1 / February 2023
- Published online by Cambridge University Press:
- 30 March 2023, pp. 143-163
-
- Article
- Export citation
A Markov Chain Model for the Evolution of Sex Ratio
-
- Journal:
- Twin Research and Human Genetics / Volume 26 / Issue 1 / February 2023
- Published online by Cambridge University Press:
- 21 March 2023, pp. 21-25
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Robustness of iterated function systems of Lipschitz maps
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 60 / Issue 3 / September 2023
- Published online by Cambridge University Press:
- 28 February 2023, pp. 921-944
- Print publication:
- September 2023
-
- Article
- Export citation
Precarious employment among South Korean women: Is inequality changing with time?
-
- Journal:
- The Economic and Labour Relations Review / Volume 28 / Issue 1 / March 2017
- Published online by Cambridge University Press:
- 01 January 2023, pp. 20-40
-
- Article
- Export citation
11 - Monte Carlo Methods
- from Part II - Statistical Molecular Dynamics
-
- Book:
- Introduction to Computational Nanomechanics
- Published online:
- 12 October 2022
- Print publication:
- 08 December 2022, pp 313-344
-
- Chapter
- Export citation
The hit-and-run version of top-to-random
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 59 / Issue 3 / September 2022
- Published online by Cambridge University Press:
- 12 July 2022, pp. 860-879
- Print publication:
- September 2022
-
- Article
- Export citation





















 . We discuss several applications and illustrate our results with examples. This includes a complete description of quasi-ergodicity for a large class of semigroups corresponding to non-local Schrödinger operators with confining potentials.
. We discuss several applications and illustrate our results with examples. This includes a complete description of quasi-ergodicity for a large class of semigroups corresponding to non-local Schrödinger operators with confining potentials.