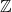 $\mathbb{Z}$
-graded identities of the Lie algebras
$\mathbb{Z}$
-graded identities of the Lie algebras
 $U_1$
in characteristic 2
$U_1$
in characteristic 2