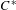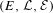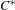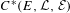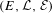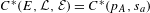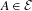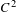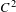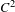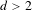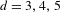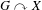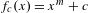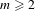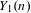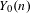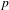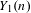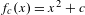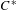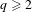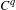Contents
Original Article
Purely infinite labeled graph
 $C^{\ast }$-algebras
$C^{\ast }$-algebras
-
- Published online by Cambridge University Press:
- 04 December 2017, pp. 2128-2158
-
- Article
- Export citation
Physical measures for certain partially hyperbolic attractors on 3-manifolds
-
- Published online by Cambridge University Press:
- 08 May 2017, pp. 74-104
-
- Article
- Export citation
Topological classification of Morse–Smale diffeomorphisms without heteroclinic curves on 3-manifolds
-
- Published online by Cambridge University Press:
- 12 December 2017, pp. 2403-2432
-
- Article
- Export citation
On the finiteness of attractors for piecewise
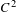 $C^{2}$ maps of the interval
$C^{2}$ maps of the interval
-
- Published online by Cambridge University Press:
- 04 December 2017, pp. 1784-1804
-
- Article
- Export citation
On the accumulation sets of exponential rays
-
- Published online by Cambridge University Press:
- 20 June 2017, pp. 370-391
-
- Article
- Export citation
Minimality for actions of abelian semigroups on compact spaces with a free interval
-
- Published online by Cambridge University Press:
- 06 March 2018, pp. 2968-2982
-
- Article
- Export citation
Distribution of shapes of orthogonal lattices
-
- Published online by Cambridge University Press:
- 02 November 2017, pp. 1531-1607
-
- Article
- Export citation
Hyperbolic geometry and pointwise ergodic theorems
-
- Published online by Cambridge University Press:
- 12 December 2017, pp. 2689-2716
-
- Article
- Export citation
A Conley-type decomposition of the strong chain recurrent set
-
- Published online by Cambridge University Press:
- 07 September 2017, pp. 1261-1274
-
- Article
- Export citation
Effective high-temperature estimates for intermittent maps
-
- Published online by Cambridge University Press:
- 12 December 2017, pp. 2159-2175
-
- Article
- Export citation
Two special subgroups of the universal sofic group
-
- Published online by Cambridge University Press:
- 10 April 2018, pp. 3250-3261
-
- Article
- Export citation
Equilibrium states for Mañé diffeomorphisms
-
- Published online by Cambridge University Press:
- 18 January 2018, pp. 2433-2455
-
- Article
- Export citation
Local and doubly empirical convergence and the entropy of algebraic actions of sofic groups
-
- Published online by Cambridge University Press:
- 07 September 2017, pp. 930-953
-
- Article
- Export citation
Hyperbolicity versus non-hyperbolic ergodic measures inside homoclinic classes
-
- Published online by Cambridge University Press:
- 07 November 2017, pp. 1805-1823
-
- Article
- Export citation
Reduction of dynatomic curves
-
- Published online by Cambridge University Press:
- 25 January 2018, pp. 2717-2768
-
- Article
- Export citation
A combinatorial classification of postcritically fixed Newton maps
-
- Published online by Cambridge University Press:
- 13 March 2018, pp. 2983-3014
-
- Article
- Export citation
The Toeplitz noncommutative solenoid and its Kubo–Martin–Schwinger states
-
- Published online by Cambridge University Press:
- 03 April 2017, pp. 105-131
-
- Article
- Export citation
Non-stationary smooth geometric structures for contracting measurable cocycles
-
- Published online by Cambridge University Press:
- 28 June 2017, pp. 392-424
-
- Article
- Export citation
When is a dynamical system mean sensitive?
-
- Published online by Cambridge University Press:
- 06 November 2017, pp. 1608-1636
-
- Article
- Export citation
Norm variation of ergodic averages with respect to two commuting transformations
-
- Published online by Cambridge University Press:
- 17 August 2017, pp. 658-688
-
- Article
- Export citation