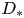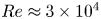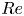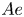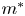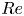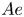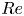80 results
Stokes flow of an evolving fluid film with arbitrary shape and topology
-
- Journal:
- Journal of Fluid Mechanics / Volume 1003 / 25 January 2025
- Published online by Cambridge University Press:
- 20 January 2025, R1
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Stability of cylindrical, multicomponent vesicles
-
- Journal:
- Journal of Fluid Mechanics / Volume 1003 / 25 January 2025
- Published online by Cambridge University Press:
- 16 January 2025, A18
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Viroporins: discovery, methods of study, and mechanisms of host-membrane permeabilization
-
- Journal:
- Quarterly Reviews of Biophysics / Volume 58 / 2025
- Published online by Cambridge University Press:
- 14 January 2025, e1
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Quasi-linear homogenization for large-inertia laminar transport across permeable membranes
-
- Journal:
- Journal of Fluid Mechanics / Volume 1000 / 10 December 2024
- Published online by Cambridge University Press:
- 27 November 2024, A46
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Optimal metabolite transport in hollow fibre membrane bioreactors
-
- Journal:
- Flow: Applications of Fluid Mechanics / Volume 4 / 2024
- Published online by Cambridge University Press:
- 15 October 2024, E18
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Long-range two-dimensional hydrodynamic interaction between a pair of mutually repellent disks
-
- Journal:
- Journal of Fluid Mechanics / Volume 988 / 10 June 2024
- Published online by Cambridge University Press:
- 26 June 2024, A39
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Stability of a dispersion of elongated particles embedded in a viscous membrane
-
- Journal:
- Journal of Fluid Mechanics / Volume 987 / 25 May 2024
- Published online by Cambridge University Press:
- 16 May 2024, R4
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
The drag of a filament moving in a supported spherical bilayer
-
- Journal:
- Journal of Fluid Mechanics / Volume 979 / 25 January 2024
- Published online by Cambridge University Press:
- 10 January 2024, A6
-
- Article
-
- You have access
- HTML
- Export citation
Homogenization theory captures macroscopic flow discontinuities across Janus membranes
-
- Journal:
- Journal of Fluid Mechanics / Volume 970 / 10 September 2023
- Published online by Cambridge University Press:
- 07 September 2023, A39
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Active nematic fluid films
-
- Journal:
- Journal of Fluid Mechanics / Volume 960 / 10 April 2023
- Published online by Cambridge University Press:
- 31 March 2023, F1
-
- Article
-
- You have access
- HTML
- Export citation
Morphodynamics of active nematic fluid surfaces
-
- Journal:
- Journal of Fluid Mechanics / Volume 957 / 25 February 2023
- Published online by Cambridge University Press:
- 10 February 2023, A4
-
- Article
- Export citation
Modelling moving contact lines on inextensible elastic sheets in two dimensions
-
- Journal:
- Journal of Fluid Mechanics / Volume 955 / 25 January 2023
- Published online by Cambridge University Press:
- 16 January 2023, A25
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Aerodynamics and fluid–structure interaction of an airfoil with actively controlled flexible leeward surface
-
- Journal:
- Journal of Fluid Mechanics / Volume 954 / 10 January 2023
- Published online by Cambridge University Press:
- 06 January 2023, A34
-
- Article
- Export citation
1 - Phases and Mesophases
-
- Book:
- Liquid Crystals and their Computer Simulations
- Published online:
- 21 July 2022
- Print publication:
- 28 July 2022, pp 1-60
-
- Chapter
- Export citation
12 - Atomistic Simulations
-
- Book:
- Liquid Crystals and their Computer Simulations
- Published online:
- 21 July 2022
- Print publication:
- 28 July 2022, pp 487-536
-
- Chapter
- Export citation
A model for the electric field-driven flow and deformation of a drop or vesicle in strong electrolyte solutions
-
- Journal:
- Journal of Fluid Mechanics / Volume 943 / 25 July 2022
- Published online by Cambridge University Press:
- 20 June 2022, A47
-
- Article
- Export citation
Fluid–structure interactions of energy-harvesting membrane hydrofoils
-
- Journal:
- Journal of Fluid Mechanics / Volume 942 / 10 July 2022
- Published online by Cambridge University Press:
- 19 May 2022, R4
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Two-dimensional hydrodynamics of a Janus particle vesicle
-
- Journal:
- Journal of Fluid Mechanics / Volume 941 / 25 June 2022
- Published online by Cambridge University Press:
- 04 May 2022, A41
-
- Article
- Export citation
Swinging and tumbling of multicomponent vesicles in flow
-
- Journal:
- Journal of Fluid Mechanics / Volume 935 / 25 March 2022
- Published online by Cambridge University Press:
- 03 February 2022, A39
-
- Article
- Export citation
Flow-excited membrane instability at moderate Reynolds numbers
-
- Journal:
- Journal of Fluid Mechanics / Volume 929 / 25 December 2021
- Published online by Cambridge University Press:
- 02 November 2021, A40
-
- Article
- Export citation