 $\boldsymbol {L^p}$
-HARDY AND
$\boldsymbol {L^p}$
-HARDY AND
 $\boldsymbol {L^p}$
-RELLICH TYPE INEQUALITIES WITH REMAINDER TERMS
$\boldsymbol {L^p}$
-RELLICH TYPE INEQUALITIES WITH REMAINDER TERMS
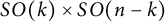 $SO(k)\times SO(n-k)$
-symmetry
$SO(k)\times SO(n-k)$
-symmetry