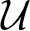 $\mathcal{U}$-frequent hypercyclicity and hypercyclicity
$\mathcal{U}$-frequent hypercyclicity and hypercyclicity
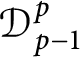 $\mathcal {D}^{p}_{p-1 }$
into tent spaces and Volterra operators
$\mathcal {D}^{p}_{p-1 }$
into tent spaces and Volterra operators
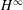 $H^{\infty }$
$H^{\infty }$