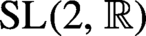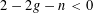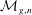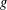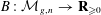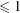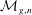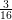Refine search
Actions for selected content:
25 results
Random walks and contracting elements I: deviation inequality and limit laws
- Part of
-
- Journal:
- Compositio Mathematica / Volume 161 / Issue 7 / July 2025
- Published online by Cambridge University Press:
- 17 September 2025, pp. 1512-1575
- Print publication:
- July 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
CHARACTER VARIETIES, EARTHQUAKES AND ESSENTIAL SURFACES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 112 / Issue 2 / October 2025
- Published online by Cambridge University Press:
- 27 June 2025, pp. 387-388
- Print publication:
- October 2025
-
- Article
-
- You have access
- HTML
- Export citation
Uniform models and short curves for random 3-manifolds
- Part of
-
- Journal:
- Compositio Mathematica / Volume 161 / Issue 3 / March 2025
- Published online by Cambridge University Press:
- 27 June 2025, pp. 447-502
- Print publication:
- March 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Counting problems from the viewpoint of ergodic theory: from primitive integer points to simple closed curves
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 45 / Issue 5 / May 2025
- Published online by Cambridge University Press:
- 24 February 2025, pp. 1281-1328
- Print publication:
- May 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Effective count of square-tiled surfaces with prescribed real and imaginary foliations in connected components of strata
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 45 / Issue 3 / March 2025
- Published online by Cambridge University Press:
- 06 November 2024, pp. 649-662
- Print publication:
- March 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
LINEAR GROWTH OF TRANSLATION LENGTHS OF RANDOM ISOMETRIES ON GROMOV HYPERBOLIC SPACES AND TEICHMÜLLER SPACES
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 23 / Issue 4 / July 2024
- Published online by Cambridge University Press:
- 06 November 2023, pp. 1751-1795
- Print publication:
- July 2024
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
SINGULAR DIRECTIONS IN VEECH SURFACES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 107 / Issue 3 / June 2023
- Published online by Cambridge University Press:
- 16 September 2022, pp. 390-397
- Print publication:
- June 2023
-
- Article
- Export citation
Distribution in the unit tangent bundle of the geodesics of given type
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 43 / Issue 3 / March 2023
- Published online by Cambridge University Press:
- 24 January 2022, pp. 887-903
- Print publication:
- March 2023
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
Typical properties of periodic Teichmüller geodesics: Lyapunov exponents
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 43 / Issue 2 / February 2023
- Published online by Cambridge University Press:
- 17 November 2021, pp. 556-584
- Print publication:
- February 2023
-
- Article
- Export citation
On Ruelle’s property
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 42 / Issue 4 / April 2022
- Published online by Cambridge University Press:
- 02 February 2021, pp. 1474-1486
- Print publication:
- April 2022
-
- Article
- Export citation
Limits of geodesic push-forwards of horocycle invariant measures
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 41 / Issue 9 / September 2021
- Published online by Cambridge University Press:
- 23 October 2020, pp. 2782-2804
- Print publication:
- September 2021
-
- Article
- Export citation
COARSE AND FINE GEOMETRY OF THE THURSTON METRIC
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 8 / 2020
- Published online by Cambridge University Press:
- 26 May 2020, e28
-
- Article
-
- You have access
- Open access
- Export citation
SQUARE-INTEGRABILITY OF THE MIRZAKHANI FUNCTION AND STATISTICS OF SIMPLE CLOSED GEODESICS ON HYPERBOLIC SURFACES
- Part of
-
- Journal:
- Forum of Mathematics, Sigma / Volume 8 / 2020
- Published online by Cambridge University Press:
- 04 February 2020, e9
-
- Article
-
- You have access
- Open access
- Export citation
On Selberg’s eigenvalue conjecture for moduli spaces of abelian differentials
- Part of
-
- Journal:
- Compositio Mathematica / Volume 155 / Issue 12 / December 2019
- Published online by Cambridge University Press:
- 30 October 2019, pp. 2354-2398
- Print publication:
- December 2019
-
- Article
- Export citation
Manhattan curves for hyperbolic surfaces with cusps
- Part of
-
- Journal:
- Ergodic Theory and Dynamical Systems / Volume 40 / Issue 7 / July 2020
- Published online by Cambridge University Press:
- 04 December 2018, pp. 1843-1874
- Print publication:
- July 2020
-
- Article
- Export citation
POLYGONAL QUASICONFORMAL MAPPINGS AND CHORD-ARC CURVES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 95 / Issue 1 / February 2017
- Published online by Cambridge University Press:
- 02 November 2016, pp. 66-72
- Print publication:
- February 2017
-
- Article
-
- You have access
- Export citation
MODULI SPACES OF SURFACES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 92 / Issue 1 / August 2015
- Published online by Cambridge University Press:
- 10 April 2015, pp. 168-170
- Print publication:
- August 2015
-
- Article
-
- You have access
- Export citation
ON STABILIZERS OF SOME TEICHMÜLLER DISKS IN POINTED MAPPING CLASS GROUPS
-
- Journal:
- Glasgow Mathematical Journal / Volume 52 / Issue 3 / September 2010
- Published online by Cambridge University Press:
- 25 August 2010, pp. 593-604
- Print publication:
- September 2010
-
- Article
-
- You have access
- Export citation
SINGULARITIES OF QUADRATIC DIFFERENTIALS AND EXTREMAL TEICHMÜLLER MAPPINGS DEFINED BY DEHN TWISTS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 87 / Issue 2 / October 2009
- Published online by Cambridge University Press:
- 09 October 2009, pp. 275-288
- Print publication:
- October 2009
-
- Article
-
- You have access
- Export citation
A MODEL OF UNIVERSAL TEICHMÜLLER SPACE AND ITS APPLICATION
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 77 / Issue 1 / February 2008
- Published online by Cambridge University Press:
- 01 February 2008, pp. 21-30
- Print publication:
- February 2008
-
- Article
-
- You have access
- Export citation