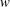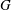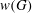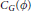Refine search
Actions for selected content:
13 results
OMEGA-CATEGORICAL PSEUDOFINITE GROUPS
- Part of
-
- Journal:
- The Journal of Symbolic Logic , First View
- Published online by Cambridge University Press:
- 15 January 2025, pp. 1-14
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
HALL CLASSES OF GROUPS WITH A LOCALLY FINITE OBSTRUCTION
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 117 / Issue 1 / August 2024
- Published online by Cambridge University Press:
- 24 July 2023, pp. 16-43
- Print publication:
- August 2024
-
- Article
- Export citation
Invariants for metabelian groups of prime power exponent, colorings, and stairs
- Part of
-
- Journal:
- Canadian Journal of Mathematics / Volume 75 / Issue 1 / February 2023
- Published online by Cambridge University Press:
- 10 December 2021, pp. 267-297
- Print publication:
- February 2023
-
- Article
- Export citation
Finite Groups and Lie Rings with an Automorphism of Order 2n
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 60 / Issue 2 / May 2017
- Published online by Cambridge University Press:
- 15 June 2016, pp. 391-412
-
- Article
- Export citation
WORDS AND PRONILPOTENT SUBGROUPS IN PROFINITE GROUPS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 97 / Issue 3 / December 2014
- Published online by Cambridge University Press:
- 29 September 2014, pp. 343-364
- Print publication:
- December 2014
-
- Article
-
- You have access
- Export citation
On Zero-divisors in Group Rings of Groups with Torsion
-
- Journal:
- Canadian Mathematical Bulletin / Volume 57 / Issue 2 / 14 June 2014
- Published online by Cambridge University Press:
- 20 November 2018, pp. 326-334
- Print publication:
- 14 June 2014
-
- Article
-
- You have access
- Export citation
ON VERBAL SUBGROUPS IN RESIDUALLY FINITE GROUPS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 84 / Issue 1 / August 2011
- Published online by Cambridge University Press:
- 10 June 2011, pp. 159-170
- Print publication:
- August 2011
-
- Article
-
- You have access
- Export citation
GROUPS WITH FINITELY MANY CONJUGACY CLASSES OF SUBGROUPS THAT ARE NOT NILPOTENT-BY-CHERNIKOV
-
- Journal:
- Glasgow Mathematical Journal / Volume 48 / Issue 2 / May 2006
- Published online by Cambridge University Press:
- 23 August 2006, pp. 247-249
- Print publication:
- May 2006
-
- Article
-
- You have access
- Export citation
POSITIVE DEFINITE FUNCTIONS OF DIAGONAL LIMITS OF FINITE ALTERNATING GROUPS
-
- Journal:
- Journal of the London Mathematical Society / Volume 70 / Issue 3 / December 2004
- Published online by Cambridge University Press:
- 03 December 2004, pp. 678-690
- Print publication:
- December 2004
-
- Article
- Export citation
Fixed Point Free Actions of Groups of Exponent 5
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 77 / Issue 3 / December 2004
- Published online by Cambridge University Press:
- 09 April 2009, pp. 297-304
- Print publication:
- December 2004
-
- Article
-
- You have access
- Export citation
Nilpotency of Some Lie Algebras Associated with p-Groups
-
- Journal:
- Canadian Journal of Mathematics / Volume 51 / Issue 3 / 01 June 1999
- Published online by Cambridge University Press:
- 20 November 2018, pp. 658-672
- Print publication:
- 01 June 1999
-
- Article
-
- You have access
- Export citation
Locally graded groups with all subgroups normal-by-finite
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics / Volume 60 / Issue 2 / April 1996
- Published online by Cambridge University Press:
- 09 April 2009, pp. 222-227
- Print publication:
- April 1996
-
- Article
-
- You have access
- Export citation
On Infinite Locally Finite Groups
-
- Journal:
- Canadian Mathematical Bulletin / Volume 37 / Issue 4 / 01 December 1994
- Published online by Cambridge University Press:
- 20 November 2018, pp. 537-544
- Print publication:
- 01 December 1994
-
- Article
-
- You have access
- Export citation
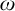
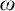
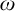





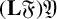
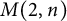
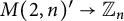
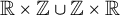
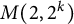
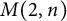
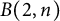
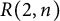
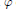 of order 2
of order 2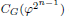 of the involution
of the involution 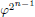 is nilpotent of class
is nilpotent of class 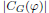 ) be the number of fixed points of
) be the number of fixed points of