We study smoothing of pencils of curves on surfaces with normal crossings. As a consequence we show that the canonical divisor of  $\overline {\mathcal {M}}_{g,n}$ is not pseudoeffective in some range, implying that
$\overline {\mathcal {M}}_{g,n}$ is not pseudoeffective in some range, implying that 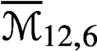 $\overline {\mathcal {M}}_{12,6}$,
$\overline {\mathcal {M}}_{12,6}$, 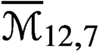 $\overline {\mathcal {M}}_{12,7}$,
$\overline {\mathcal {M}}_{12,7}$, 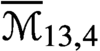 $\overline {\mathcal {M}}_{13,4}$ and
$\overline {\mathcal {M}}_{13,4}$ and  $\overline {\mathcal {M}}_{14,3}$ are uniruled. We provide upper bounds for the Kodaira dimension of
$\overline {\mathcal {M}}_{14,3}$ are uniruled. We provide upper bounds for the Kodaira dimension of 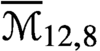 $\overline {\mathcal {M}}_{12,8}$ and
$\overline {\mathcal {M}}_{12,8}$ and  $\overline {\mathcal {M}}_{16}$. We also show that the moduli space of
$\overline {\mathcal {M}}_{16}$. We also show that the moduli space of  $(4g+5)$-pointed hyperelliptic curves
$(4g+5)$-pointed hyperelliptic curves 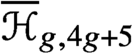 $\overline {\mathcal {H}}_{g,4g+5}$ is uniruled. Together with a recent result of Schwarz, this concludes the classification of moduli of pointed hyperelliptic curves with negative Kodaira dimension.
$\overline {\mathcal {H}}_{g,4g+5}$ is uniruled. Together with a recent result of Schwarz, this concludes the classification of moduli of pointed hyperelliptic curves with negative Kodaira dimension.
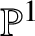 $\mathbb {P}^1$-invariance
$\mathbb {P}^1$-invariance
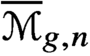 $\overline {\mathcal {M}}_{g,n}$
$\overline {\mathcal {M}}_{g,n}$