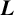 $\boldsymbol {L}$
-FUNCTIONS
$\boldsymbol {L}$
-FUNCTIONS
 $\boldsymbol {L}$
-FUNCTIONS
$\boldsymbol {L}$
-FUNCTIONS
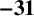 $\boldsymbol {-31}$
TO MODULAR FORMS
$\boldsymbol {-31}$
TO MODULAR FORMS
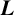 $\boldsymbol {L}$
-VALUE
$\boldsymbol {L}$
-VALUE
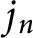 $j_n$
$j_n$