Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ganesh, Jadav
Ramesh, Golla
and
Sukumar, Daniel
2015.
On the structure of absolutely minimum attaining operators.
Journal of Mathematical Analysis and Applications,
Vol. 428,
Issue. 1,
p.
457.
PANDEY, SATISH K.
and
PAULSEN, VERN I.
2017.
A SPECTRAL CHARACTERIZATION OF OPERATORS.
Journal of the Australian Mathematical Society,
Vol. 102,
Issue. 3,
p.
369.
Ramesh, G.
2018.
Absolutely norm attaining paranormal operators.
Journal of Mathematical Analysis and Applications,
Vol. 465,
Issue. 1,
p.
547.
J., Ganesh
G., Ramesh
and
D., Sukumar
2018.
A characterization of absolutely minimum attaining operators.
Journal of Mathematical Analysis and Applications,
Vol. 468,
Issue. 1,
p.
567.
Venku Naidu, D
and
Ramesh, G
2019.
On absolutely norm attaining operators.
Proceedings - Mathematical Sciences,
Vol. 129,
Issue. 4,
Bala, Neeru
and
Golla, Ramesh
2020.
Spectral properties of absolutely minimum attaining operators.
Banach Journal of Mathematical Analysis,
Vol. 14,
Issue. 3,
p.
630.
Kulkarni, S. H.
and
Ramesh, G.
2021.
Absolutely minimum attaining closed operators.
The Journal of Analysis,
Vol. 29,
Issue. 2,
p.
473.
Bala, Neeru
Dhara, Kousik
Sarkar, Jaydeb
and
Sensarma, Aryaman
2021.
Idempotent, model, and Toeplitz operators attaining their norms.
Linear Algebra and its Applications,
Vol. 622,
Issue. ,
p.
150.
Ramesh, G.
and
Sequeira, Shanola S.
2023.
On the closure of absolutely norm attaining operators.
Linear and Multilinear Algebra,
Vol. 71,
Issue. 18,
p.
2894.
Golla, Ramesh
Osaka, Hiroyuki
Udagawa, Yoichi
and
Yamazaki, Takeaki
2023.
Stability of AN-property for the induced Aluthge transformations.
Linear Algebra and its Applications,
Vol. 678,
Issue. ,
p.
206.
Kulkarni, S. H.
and
Ramesh, G.
2025.
Inverse Problems, Regularization Methods and Related Topics.
p.
273.
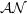 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathcal{AN}$-OPERATORS
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathcal{AN}$-OPERATORS $\mathcal{AN}$-operators between separable, complex Hilbert spaces which is similar to that of the singular value decomposition of a compact operator. Apart from this, we show that a bounded operator is
$\mathcal{AN}$-operators between separable, complex Hilbert spaces which is similar to that of the singular value decomposition of a compact operator. Apart from this, we show that a bounded operator is 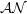 $\mathcal{AN}$ if and only if it is either compact or a sum of a compact operator and scalar multiple of an isometry satisfying some condition. We obtain characterizations of these operators as a consequence of this structure theorem and deduce several properties which are similar to those of compact operators.
$\mathcal{AN}$ if and only if it is either compact or a sum of a compact operator and scalar multiple of an isometry satisfying some condition. We obtain characterizations of these operators as a consequence of this structure theorem and deduce several properties which are similar to those of compact operators.