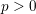Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Biswas, Indranil
Hai, Phùng Hô
and
Dos Santos, João Pedro
2021.
On the fundamental group schemes of certain quotient varieties.
Tohoku Mathematical Journal,
Vol. 73,
Issue. 4,
Shuddhodan, K.V.
2022.
The (non-uniform) Hrushovski-Lang-Weil estimates.
Advances in Mathematics,
Vol. 410,
Issue. ,
p.
108753.