Published online by Cambridge University Press: 13 September 2017
Let 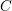 $C$ be a Petri general curve of genus
$C$ be a Petri general curve of genus  $g$ and
$g$ and  $E$ a general stable vector bundle of rank
$E$ a general stable vector bundle of rank  $r$ and slope
$r$ and slope 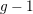 $g-1$ over
$g-1$ over 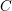 $C$ with
$C$ with 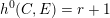 $h^{0}(C,E)=r+1$. For
$h^{0}(C,E)=r+1$. For 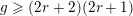 $g\geqslant (2r+2)(2r+1)$, we show how the bundle
$g\geqslant (2r+2)(2r+1)$, we show how the bundle 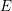 $E$ can be recovered from the tangent cone to the generalised theta divisor
$E$ can be recovered from the tangent cone to the generalised theta divisor 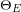 $\unicode[STIX]{x1D6E9}_{E}$ at
$\unicode[STIX]{x1D6E9}_{E}$ at 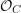 ${\mathcal{O}}_{C}$. We use this to give a constructive proof and a sharpening of Brivio and Verra’s theorem that the theta map
${\mathcal{O}}_{C}$. We use this to give a constructive proof and a sharpening of Brivio and Verra’s theorem that the theta map  $\mathit{SU}_{C}(r){\dashrightarrow}|r\unicode[STIX]{x1D6E9}|$ is generically injective for large values of
$\mathit{SU}_{C}(r){\dashrightarrow}|r\unicode[STIX]{x1D6E9}|$ is generically injective for large values of 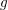 $g$.
$g$.