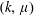Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Dey, Chiranjib
and
De, Uday Chand
2020.
A note on quasi-Yamabe solitons on contact metric manifolds.
Journal of Geometry,
Vol. 111,
Issue. 1,
De, Uday Chand
Chaubey, Sudhakar K.
and
Suh, Young Jin
2020.
A note on almost co-Kähler manifolds.
International Journal of Geometric Methods in Modern Physics,
Vol. 17,
Issue. 10,
p.
2050153.
Chaubey, Sudhakar K.
and
De, Uday Chand
2020.
Characterization of three-dimensional Riemannian manifolds with a type of semi-symmetric metric connection admitting Yamabe soliton.
Journal of Geometry and Physics,
Vol. 157,
Issue. ,
p.
103846.
Suh, Young Jin
and
De, Uday Chand
2020.
Yamabe solitons and gradient Yamabe solitons on three-dimensional N(k)-contact manifolds.
International Journal of Geometric Methods in Modern Physics,
Vol. 17,
Issue. 12,
p.
2050177.
Mallesha Naik, Devaraja
Venkatesha, V.
and
Aruna Kumara, H.
2020.
Ricci Solitons and Certain Related Metrics on Almost Co-Kaehler Manifolds.
Zurnal matematiceskoj fiziki, analiza, geometrii,
Vol. 16,
Issue. 4,
p.
402.
Chen, Xiaomin
2020.
Almost quasi-Yamabe solitons on almost cosymplectic manifolds.
International Journal of Geometric Methods in Modern Physics,
Vol. 17,
Issue. 05,
p.
2050070.
Chen, Xiaomin
2021.
Cotton solitons on almost cokähler 3-manifolds.
Quaestiones Mathematicae,
Vol. 44,
Issue. 8,
p.
1055.
De, Uday Chand
Suh, Young Jin
and
Chaubey, Sudhakar K.
2021.
Conformal vector fields on almost co-Kähler manifolds.
Mathematica Slovaca,
Vol. 71,
Issue. 6,
p.
1545.
Chen, Xiaomin
and
De, Uday Chand
2021.
The k-almost Yamabe solitons and almost coKähler manifolds.
International Journal of Geometric Methods in Modern Physics,
Vol. 18,
Issue. 11,
p.
2150179.
De, Uday Chand
Chaubey, Sudhakar K.
and
Suh, Young Jin
2021.
Gradient Yamabe and Gradient m-Quasi Einstein Metrics on Three-dimensional Cosymplectic Manifolds.
Mediterranean Journal of Mathematics,
Vol. 18,
Issue. 3,
Sardar, Arpan
Khan, Mohammad Nazrul Islam
and
De, Uday Chand
2021.
η-∗-Ricci Solitons and Almost co-Kähler Manifolds.
Mathematics,
Vol. 9,
Issue. 24,
p.
3200.
Alkhaldi, Ali H.
Laurian-Ioan, Pişcoran
Abolarinwa, Abimbola
and
Ali, Akram
2021.
Characterization of Almost Yamabe Solitons and Gradient Almost Yamabe Solitons with Conformal Vector Fields.
Symmetry,
Vol. 13,
Issue. 12,
p.
2362.
Wang, Yaning
2021.
Almost Kenmotsu $$(k,\mu )'$$-manifolds with Yamabe solitons.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 115,
Issue. 1,
De, Uday Chand
and
Suh, Young Jin
2021.
Yamabe and quasi-Yamabe solitons in paracontact metric manifolds.
International Journal of Geometric Methods in Modern Physics,
Vol. 18,
Issue. 12,
De, U. C.
Chaubey, S. K.
and
Shenawy, S.
2021.
Perfect fluid spacetimes and Yamabe solitons.
Journal of Mathematical Physics,
Vol. 62,
Issue. 3,
De, Krishnendu
and
De, Uday Chand
2021.
$$\delta $$-Almost Yamabe Solitons in Paracontact Metric Manifolds.
Mediterranean Journal of Mathematics,
Vol. 18,
Issue. 5,
De, Krishnendu
and
De, Uday Chand
2021.
Almost quasi-Yamabe solitons and gradient almost quasi-Yamabe solitons in paracontact geometry.
Quaestiones Mathematicae,
Vol. 44,
Issue. 11,
p.
1429.
De, Krishnendu
and
De, Uday Chand
2021.
A note on gradient solitons on para-Kenmotsu manifolds.
International Journal of Geometric Methods in Modern Physics,
Vol. 18,
Issue. 01,
p.
2150007.
Wang, Wenjie
2022.
K-cosymplectic metric as an η-Ricci soliton.
International Journal of Geometric Methods in Modern Physics,
Vol. 19,
Issue. 05,
Chaubey, Sudhakar K.
and
Vîlcu, Gabriel-Eduard
2022.
Gradient Ricci solitons and Fischer–Marsden equation on cosymplectic manifolds.
Revista de la Real Academia de Ciencias Exactas, Físicas y Naturales. Serie A. Matemáticas,
Vol. 116,
Issue. 4,