Integral equations of Oseen type are first kind Fredholm equations whose kernels have a logarithmic singularity. They arise in exterior boundary value problems in fluid flow and heat transfer. Subject to the assumption of uniqueness of solutions of the parent exterior boundary value problem, solutions of the Oseen type integral equations are shown to exist.

We develop some comparison theorems that connect the solutions of a differential system and its perturbation in a manner useful in the practical stability.

Some time ago Barry [Proc. London Math. Soc. 12 (1962), 445–495] established the right connection between the smallest and largest values of small subharmonic functions on certain circles about the origin. The behaviour of functions extremal for this connection is investigated.

Call a relation R⊆A2 (A some non-empty set) monadically representable when there exist F, G⊆A such that R = {(x, y) | x ∈ F ∘ y ∈ G} for some truth-functional connective ∘. This note finds a first-order condition on R which is necessary and sufficient for R to be monadically representable.

Let be analytic in the unit disc U(|z| <1) and let F(z) = (l-λ)f (Z) + λ(f(z)*h(z)) where with cn 's are known and are nonnegative, λ ≥ 0 In the present paper, using convolution methods we investigate the mapping properties of F(z) when f(z) belongs respectively to several subclasses of analytic functions with negative coefficients.

Sufficient conditions are derived for all bounded solutions of a class of linear systems of differential difference equations to be oscillatory.

It is shown that if a McLain group is perfect, then it is super-perfect. The proof involves demonstrating that any dense linearly ordered set has the apparently stronger property of being superdense.

A norm |·| of a Banach space x is called locally uniformly rotund if lim|xn−x| = 0 whenever xn, x ∈ X, and 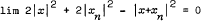. It is shown that such an equivalent norm exists on every Banach space x which possesses a projectional resolution {pα} of the identify operator, for which all (pα+1−pα)X admit such norms. This applies, for example, for the dual space of a space with Fréchet differentiable norm.

The Liapunov-Razumikhin technique has been employed to study Lp stability properties of solutions of functional differential equations of delay type where the delay becomes unbounded as t ↠ +∞. These results have been applied to investigate sufficient conditions for L2-stability of Volterra integro-differentlal equations.

An eulerian chain in a graph is a continuous route which traces every edge exactly once. It may be finite or infinite, and may have 0,1 or 2 end vertices. For each kind of eulerian chain, there is a characterization of the graphs which admit such a tracing. This paper derives a uniform characterization of graphs with an eulerian chain, regardless of the kind of chain. Relationships between the edge complements of various kinds of finite subgraphs are also investigated, and hence a sharpened version of the eulerian chain characterization is derived.

In this lecture we describe, discuss and compare the two classes of methods most commonly used for the numerical solution of boundary value problems for partial differential equations, namely, the finite difference method and the finite element method. For both of these methods an extensive development of mathematical error analysis has taken place but individual numerical analysts often express strong prejudices in favor of one of them. Our purpose is to try to convey our conviction that this attitude is both historically unjustified and inhibiting, and that familiarity with both methods provides a wider range of techniques for constructing and analyzing discretization schemes.

The method of proof of Magnus introduced in 1930 is adapted to prove the following theorem of Howie. If A and B are groups for which every finitely generated subgroup has an infinite cyclic image, and if one adds an additional relation (with obvious exceptions), then in the resultant group both A and B appear isomorphically.

In this paper we show that an R-module graph over a semisimple ring R can be written as a direct sum of graphic submodules that are uniquely determined up to isomorphism type. Moreover, this decomposition enables us to describe the R-module graph in graphic terms as a disjoint union of connected components, each of which consists of a complete directed graph on its vertices together with a set of loops at each vertex, determined by the loops at 0. We also give a graphic version of Maschke's Theorem.