Refine search
Actions for selected content:
8 results
AN EFFECTIVE VERSION OF THE PRIMITIVE ELEMENT THEOREM
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society , First View
- Published online by Cambridge University Press:
- 13 October 2025, pp. 1-8
-
- Article
- Export citation
THE DENSITY OF
 $j$-WISE RELATIVELY
$j$-WISE RELATIVELY  $r$-PRIME ALGEBRAIC INTEGERS
$r$-PRIME ALGEBRAIC INTEGERS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 98 / Issue 2 / October 2018
- Published online by Cambridge University Press:
- 06 July 2018, pp. 221-229
- Print publication:
- October 2018
-
- Article
-
- You have access
- Export citation
The equidistribution of lattice shapes of rings of integers in cubic, quartic, and quintic number fields
- Part of
-
- Journal:
- Compositio Mathematica / Volume 152 / Issue 6 / June 2016
- Published online by Cambridge University Press:
- 15 April 2016, pp. 1111-1120
- Print publication:
- June 2016
-
- Article
-
- You have access
- Export citation
ON THE MERTENS–CESÀRO THEOREM FOR NUMBER FIELDS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 93 / Issue 2 / April 2016
- Published online by Cambridge University Press:
- 11 November 2015, pp. 199-210
- Print publication:
- April 2016
-
- Article
-
- You have access
- Export citation
ON THE DISTRIBUTION OF TORSION POINTS MODULO PRIMES
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 86 / Issue 2 / October 2012
- Published online by Cambridge University Press:
- 16 February 2012, pp. 339-347
- Print publication:
- October 2012
-
- Article
-
- You have access
- Export citation
Diamonds in Torsion of Abelian Varieties
- Part of
-
- Journal:
- Journal of the Institute of Mathematics of Jussieu / Volume 9 / Issue 3 / July 2010
- Published online by Cambridge University Press:
- 10 February 2010, pp. 477-480
- Print publication:
- July 2010
-
- Article
- Export citation
Abelian varieties over $\mathbb{Q}$ with bad reduction in one prime only
-
- Journal:
- Compositio Mathematica / Volume 141 / Issue 4 / July 2005
- Published online by Cambridge University Press:
- 21 June 2005, pp. 847-868
- Print publication:
- July 2005
-
- Article
-
- You have access
- Export citation
Convolution Structures and Arithmetic Cohomology
-
- Journal:
- Compositio Mathematica / Volume 136 / Issue 3 / May 2003
- Published online by Cambridge University Press:
- 04 December 2007, pp. 237-254
- Print publication:
- May 2003
-
- Article
-
- You have access
- Export citation












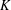
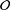
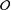
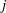

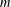
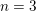
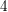
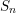

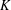
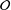


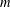
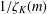
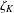
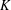
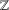
 be a commutative algebraic group defined over a number field
be a commutative algebraic group defined over a number field 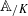 , we determine the average values of
, we determine the average values of 