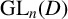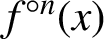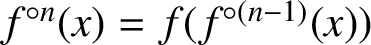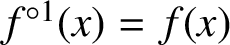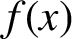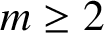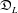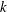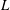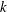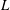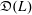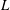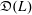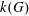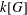Refine search
Actions for selected content:
7 results
Products of commutators in matrix rings
- Part of
-
- Journal:
- Canadian Mathematical Bulletin / Volume 68 / Issue 2 / June 2025
- Published online by Cambridge University Press:
- 14 January 2025, pp. 512-529
- Print publication:
- June 2025
-
- Article
-
- You have access
- Open access
- HTML
- Export citation
LOCALLY NILPOTENT SUBGROUPS OF
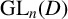 $\mathrm {GL}_n(D)$
$\mathrm {GL}_n(D)$
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 112 / Issue 1 / August 2025
- Published online by Cambridge University Press:
- 06 November 2024, pp. 128-138
- Print publication:
- August 2025
-
- Article
- Export citation
A NOTE ON SUBNORMAL SUBGROUPS IN DIVISION RINGS CONTAINING SOLVABLE SUBGROUPS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 108 / Issue 3 / December 2023
- Published online by Cambridge University Press:
- 11 January 2023, pp. 422-427
- Print publication:
- December 2023
-
- Article
- Export citation
FIXED POINTS OF POLYNOMIALS OVER DIVISION RINGS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 104 / Issue 2 / October 2021
- Published online by Cambridge University Press:
- 01 March 2021, pp. 256-262
- Print publication:
- October 2021
-
- Article
- Export citation
Free Group Algebras in Division Rings with Valuation II
- Part of
-
- Journal:
- Canadian Journal of Mathematics / Volume 72 / Issue 6 / December 2020
- Published online by Cambridge University Press:
- 05 July 2019, pp. 1463-1504
- Print publication:
- December 2020
-
- Article
- Export citation
Stable division rings
-
- Journal:
- The Journal of Symbolic Logic / Volume 76 / Issue 1 / March 2011
- Published online by Cambridge University Press:
- 12 March 2014, pp. 348-352
- Print publication:
- March 2011
-
- Article
- Export citation
A NOTE ON GROUP IDENTITIES IN DIVISION RINGS
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 47 / Issue 3 / October 2004
- Published online by Cambridge University Press:
- 09 November 2004, pp. 557-560
-
- Article
-
- You have access
- Export citation