7 results
A SCHWARZ LEMMA FOR
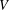 $V$-HARMONIC MAPS AND THEIR APPLICATIONS
$V$-HARMONIC MAPS AND THEIR APPLICATIONS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 96 / Issue 3 / December 2017
- Published online by Cambridge University Press:
- 17 August 2017, pp. 504-512
- Print publication:
- December 2017
-
- Article
-
- You have access
- Export citation
BIHARMONIC LAGRANGIAN SUBMANIFOLDS IN KÄHLER MANIFOLDS
-
- Journal:
- Glasgow Mathematical Journal / Volume 55 / Issue 2 / May 2013
- Published online by Cambridge University Press:
- 25 February 2013, pp. 465-480
- Print publication:
- May 2013
-
- Article
-
- You have access
- Export citation
Harmonic maps between rotationally symmetric manifolds
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 55 / Issue 3 / October 2012
- Published online by Cambridge University Press:
- 26 July 2012, pp. 685-695
-
- Article
-
- You have access
- Export citation
ON LEGENDRE CURVES IN CONTACT PSEUDO-HERMITIAN 3-MANIFOLDS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 81 / Issue 1 / February 2010
- Published online by Cambridge University Press:
- 05 November 2009, pp. 156-164
- Print publication:
- February 2010
-
- Article
-
- You have access
- Export citation
On the classification of rank-two representations of quasiprojective fundamental groups
- Part of
-
- Journal:
- Compositio Mathematica / Volume 144 / Issue 5 / September 2008
- Published online by Cambridge University Press:
- 01 September 2008, pp. 1271-1331
- Print publication:
- September 2008
-
- Article
-
- You have access
- Export citation
BIMINIMAL IMMERSIONS
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 51 / Issue 2 / June 2008
- Published online by Cambridge University Press:
- 28 July 2008, pp. 421-437
-
- Article
-
- You have access
- Export citation
Stability and constant boundary-value problems of harmonic maps with potential
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics / Volume 68 / Issue 2 / April 2000
- Published online by Cambridge University Press:
- 09 April 2009, pp. 145-154
- Print publication:
- April 2000
-
- Article
-
- You have access
- Export citation