We present some properties of orthogonality and relate them with support disjoint and norm inequalities in 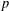$p$-Schatten ideals. In addition, we investigate the problem of characterization of norm-parallelism for bounded linear operators. We consider the characterization of the norm-parallelism problem in $p$-Schatten ideals and locally uniformly convex spaces. Later on, we study the case when an operator is norm-parallel to the identity operator. Finally, we give some equivalence assertions about the norm-parallelism of compact operators. Some applications and generalizations are discussed for certain operators.

Let $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}H$ be a linear unbounded operator in a Hilbert space. It is assumed that the resolvent of $H$ is a compact operator and $H-H^*$ is a Hilbert–Schmidt operator. Various integro-differential operators satisfy these conditions. It is shown that $H$ is similar to a normal operator and a sharp bound for the condition number is suggested. We also discuss applications of that bound to spectrum perturbations and operator functions.

The notion of a completely hyperexpansive operator has been introduced in [1] by Athavale. In this paper hyperexpansive operator valued unilateral weighted shifts are investigated. A unique semispectral measure is associated with a bounded completely hyperexpansive operator valued unilateral weighted shift with invertible weights. Examples of bounded and unbounded hyperexpansive operator valued unilateral weighted shifts with invariant domains are given.