Refine search
Actions for selected content:
5 results
NORMAL BASES FOR MODULAR FUNCTION FIELDS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 95 / Issue 3 / June 2017
- Published online by Cambridge University Press:
- 02 March 2017, pp. 384-392
- Print publication:
- June 2017
-
- Article
-
- You have access
- Export citation
PARAMETRIZING ELLIPTIC CURVES BY MODULAR UNITS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 100 / Issue 1 / February 2016
- Published online by Cambridge University Press:
- 19 August 2015, pp. 33-41
- Print publication:
- February 2016
-
- Article
-
- You have access
- Export citation
ON POWER MOMENTS OF THE HECKE MULTIPLICATIVE FUNCTIONS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 99 / Issue 3 / December 2015
- Published online by Cambridge University Press:
- 25 March 2015, pp. 334-340
- Print publication:
- December 2015
-
- Article
-
- You have access
- Export citation
SIGN CHANGES OF FOURIER COEFFICIENTS OF ENTIRE MODULAR INTEGRALS
-
- Journal:
- Glasgow Mathematical Journal / Volume 54 / Issue 2 / May 2012
- Published online by Cambridge University Press:
- 29 March 2012, pp. 355-358
- Print publication:
- May 2012
-
- Article
-
- You have access
- Export citation
On the AF-algebra of a Hecke eigenform
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 55 / Issue 1 / February 2012
- Published online by Cambridge University Press:
- 08 November 2011, pp. 207-213
-
- Article
-
- You have access
- Export citation
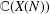
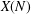

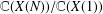
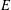
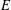
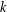
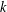
 provided that the associated period functions are polynomials.
provided that the associated period functions are polynomials.