Published online by Cambridge University Press: 19 August 2015
It is well known that every elliptic curve over the rationals admits a parametrization by means of modular functions. In this short note, we show that only finitely many elliptic curves over 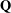 $\mathbf{Q}$ can be parametrized by modular units. This answers a question raised by W. Zudilin in a recent work on Mahler measures. Further, we give the list of all elliptic curves
$\mathbf{Q}$ can be parametrized by modular units. This answers a question raised by W. Zudilin in a recent work on Mahler measures. Further, we give the list of all elliptic curves 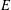 $E$ of conductor up to 1000 parametrized by modular units supported in the rational torsion subgroup of
$E$ of conductor up to 1000 parametrized by modular units supported in the rational torsion subgroup of 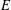 $E$. Finally, we raise several open questions.
$E$. Finally, we raise several open questions.