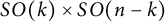 $SO(k)\times SO(n-k)$
-symmetry
$SO(k)\times SO(n-k)$
-symmetry
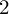 $2$
-SPHERES OF CONSTANT CURVATURE
$2$
-SPHERES OF CONSTANT CURVATURE
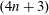 $(4n+3)$-dimensional Spheres
$(4n+3)$-dimensional Spheres