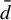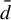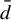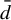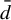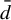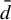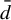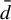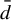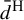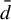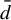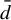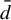1. Introduction
Given a finite set(an alphabet)
![]() $\mathscr {A}$
we let
$\mathscr {A}$
we let
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
stand for the full shift over
${\mathscr {A}^{\hspace{2pt}\infty} }$
stand for the full shift over
![]() $\mathscr {A}$
, that is, a set of all
$\mathscr {A}$
, that is, a set of all
![]() $\mathscr {A}$
-valued infinite sequences. To avoid trivialities, we assume that
$\mathscr {A}$
-valued infinite sequences. To avoid trivialities, we assume that
![]() $\mathscr {A}$
has at least two elements. We endow
$\mathscr {A}$
has at least two elements. We endow
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
with the product topology induced by the discrete topology on
${\mathscr {A}^{\hspace{2pt}\infty} }$
with the product topology induced by the discrete topology on
![]() $\mathscr {A}$
, which turns
$\mathscr {A}$
, which turns
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
into a compact metrizable space. Let
${\mathscr {A}^{\hspace{2pt}\infty} }$
into a compact metrizable space. Let
![]() $\rho $
be a metric compatible with the topology on
$\rho $
be a metric compatible with the topology on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. The shift operator
${\mathscr {A}^{\hspace{2pt}\infty} }$
. The shift operator
![]() $\sigma \colon {\mathscr {A}^{\hspace{2pt}\infty} }\to {\mathscr {A}^{\hspace{2pt}\infty} }$
turns
$\sigma \colon {\mathscr {A}^{\hspace{2pt}\infty} }\to {\mathscr {A}^{\hspace{2pt}\infty} }$
turns
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
into a non-invertible dynamical system. From the dynamical point of view, the most interesting objects are closed non-empty
${\mathscr {A}^{\hspace{2pt}\infty} }$
into a non-invertible dynamical system. From the dynamical point of view, the most interesting objects are closed non-empty
![]() $\sigma $
-invariant subsets of
$\sigma $
-invariant subsets of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
(one-sided shift spaces or subshifts). We also consider the space
${\mathscr {A}^{\hspace{2pt}\infty} }$
(one-sided shift spaces or subshifts). We also consider the space
![]() $\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
of all Borel probability measures on
$\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
of all Borel probability measures on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
with the weak
${\mathscr {A}^{\hspace{2pt}\infty} }$
with the weak
![]() $^*$
topology. The set of
$^*$
topology. The set of
![]() $\sigma $
-invariant measures in
$\sigma $
-invariant measures in
![]() $\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
concentrated on a shift space
$\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
concentrated on a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is denoted by
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is denoted by
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
. Each of these objects (invariant measures and subshifts) has a canonically defined sequence of Markov approximations converging to it in a natural topology. This fact, however, is of little practical use, because the convergence is too weak to allow for a transfer of dynamical properties from an approximating sequence to the properties of its limit.
${\mathcal {M}_{\mathit \sigma }}(X)$
. Each of these objects (invariant measures and subshifts) has a canonically defined sequence of Markov approximations converging to it in a natural topology. This fact, however, is of little practical use, because the convergence is too weak to allow for a transfer of dynamical properties from an approximating sequence to the properties of its limit.
Recall that the natural topology on the space of all subshifts of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
is the hyperspace (Vietoris) topology of non-empty closed subsets of a compact metric space. In other words, a sequence of shift spaces
${\mathscr {A}^{\hspace{2pt}\infty} }$
is the hyperspace (Vietoris) topology of non-empty closed subsets of a compact metric space. In other words, a sequence of shift spaces
![]() $(X_n)_{n=1}^{\infty} \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
converges to a shift space
$(X_n)_{n=1}^{\infty} \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
converges to a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
in the hyperspace topology if
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
in the hyperspace topology if
![]() $\rho ^{\mathrm {H}}(X_n,X)\to 0$
as
$\rho ^{\mathrm {H}}(X_n,X)\to 0$
as
![]() $n\to \infty $
(here,
$n\to \infty $
(here,
![]() $\rho ^{\mathrm {H}}$
is the Hausdorff metric corresponding to
$\rho ^{\mathrm {H}}$
is the Hausdorff metric corresponding to
![]() $\rho $
). Similarly, we say that simplices of invariant measures of shift spaces
$\rho $
). Similarly, we say that simplices of invariant measures of shift spaces
![]() $(X_n)_{n=1}^{\infty} \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
approximate the simplex of invariant measures of a shift space
$(X_n)_{n=1}^{\infty} \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
approximate the simplex of invariant measures of a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
if
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
if
![]() ${\mathcal {M}_{\mathit \sigma }}(X_n)$
converges to
${\mathcal {M}_{\mathit \sigma }}(X_n)$
converges to
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
as
${\mathcal {M}_{\mathit \sigma }}(X)$
as
![]() $n\to \infty $
in the natural hyperspace topology of
$n\to \infty $
in the natural hyperspace topology of
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
, that is, if
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
, that is, if
![]() $D^{\mathrm {H}}({\mathcal {M}_{\mathit \sigma }}(X_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
$D^{\mathrm {H}}({\mathcal {M}_{\mathit \sigma }}(X_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
![]() $n\to \infty $
, where
$n\to \infty $
, where
![]() $D^{\mathrm {H}}$
is the Hausdorff metric corresponding to a metric D compatible with the weak
$D^{\mathrm {H}}$
is the Hausdorff metric corresponding to a metric D compatible with the weak
![]() $^*$
topology on
$^*$
topology on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
.
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
.
Fortunately, for both measures and subshifts, stronger metrics than
![]() $\rho $
and D are also available. A useful metric for
$\rho $
and D are also available. A useful metric for
![]() $\sigma $
-invariant measures is Ornstein’s metric
$\sigma $
-invariant measures is Ornstein’s metric
![]() $\bar {d}_{\mathcal {M}}$
. In [Reference Konieczny, Kupsa and Kwietniak23] we studied a topology on the powerset of
$\bar {d}_{\mathcal {M}}$
. In [Reference Konieczny, Kupsa and Kwietniak23] we studied a topology on the powerset of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
induced by the Hausdorff pseudometric
${\mathscr {A}^{\hspace{2pt}\infty} }$
induced by the Hausdorff pseudometric
![]() ${\bar d}^{\mathrm {H}}$
derived from
${\bar d}^{\mathrm {H}}$
derived from
![]() $\bar {d}$
-pseudometric on
$\bar {d}$
-pseudometric on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. A very similar idea of using
${\mathscr {A}^{\hspace{2pt}\infty} }$
. A very similar idea of using
![]() $\bar {d}$
-approximation was independently considered by Thompson [Reference Thompson42], who used it in the settings of [Reference Climenhaga and Thompson4].
$\bar {d}$
-approximation was independently considered by Thompson [Reference Thompson42], who used it in the settings of [Reference Climenhaga and Thompson4].
Recall that the pseudometric
![]() $\bar {d}$
is given for
$\bar {d}$
is given for
![]() $x=(x_j)_{j=0}^{\infty} ,\ y=(y_j)_{j=0}^{\infty} \in {\mathscr {A}^{\hspace{2pt}\infty} }$
by
$x=(x_j)_{j=0}^{\infty} ,\ y=(y_j)_{j=0}^{\infty} \in {\mathscr {A}^{\hspace{2pt}\infty} }$
by
Since
![]() $\bar {d}(x,y)$
can be zero for distinct x and y,
$\bar {d}(x,y)$
can be zero for distinct x and y,
![]() $\bar {d}$
is not a metric. Nevertheless, after factorizing by the equivalence relation
$\bar {d}$
is not a metric. Nevertheless, after factorizing by the equivalence relation
![]() $\sim $
on
$\sim $
on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
, where
${\mathscr {A}^{\hspace{2pt}\infty} }$
, where
![]() $x\sim y$
if and only if
$x\sim y$
if and only if
![]() $\bar {d}(x,y)=0$
, we obtain the factor space
$\bar {d}(x,y)=0$
, we obtain the factor space
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }/{\sim }$
on which
${\mathscr {A}^{\hspace{2pt}\infty} }/{\sim }$
on which
![]() $\bar {d}$
becomes a complete, non-separable metric. Since
$\bar {d}$
becomes a complete, non-separable metric. Since
![]() $\bar {d}$
is bounded by
$\bar {d}$
is bounded by
![]() $1$
on
$1$
on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
, it induces a Hausdorff pseudometric
${\mathscr {A}^{\hspace{2pt}\infty} }$
, it induces a Hausdorff pseudometric
![]() ${\bar d}^{\mathrm {H}}$
on the space
${\bar d}^{\mathrm {H}}$
on the space
![]() $\operatorname {\mathrm {CL}}({\mathscr {A}^{\hspace{2pt}\infty} },\bar {d})$
of all non-empty
$\operatorname {\mathrm {CL}}({\mathscr {A}^{\hspace{2pt}\infty} },\bar {d})$
of all non-empty
![]() $\bar {d}$
-closed subsets of
$\bar {d}$
-closed subsets of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. Similarly,
${\mathscr {A}^{\hspace{2pt}\infty} }$
. Similarly,
![]() $\bar {d}_{\mathcal {M}}$
is a complete bounded non-separable metric on
$\bar {d}_{\mathcal {M}}$
is a complete bounded non-separable metric on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
inducing a Hausdorff metric
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
inducing a Hausdorff metric
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
on the space
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
on the space
![]() $\operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
of all non-empty
$\operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
of all non-empty
![]() $\bar {d}_{\mathcal {M}}$
-closed subsets of
$\bar {d}_{\mathcal {M}}$
-closed subsets of
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
. Note that
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
. Note that
![]() $\bar {d}_{\mathcal {M}}$
-convergence implies weak
$\bar {d}_{\mathcal {M}}$
-convergence implies weak
![]() $^*$
convergence, so for each shift space
$^*$
convergence, so for each shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
the set
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
the set
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
is
${\mathcal {M}_{\mathit \sigma }}(X)$
is
![]() $\bar {d}_{\mathcal {M}}$
-closed. It is also known that the set of ergodic measures on X, denoted by
$\bar {d}_{\mathcal {M}}$
-closed. It is also known that the set of ergodic measures on X, denoted by
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)$
, is
${\mathcal {M}_{\mathit \sigma }}e(X)$
, is
![]() $\bar {d}_{\mathcal {M}}$
-closed.
$\bar {d}_{\mathcal {M}}$
-closed.
Hence, we obtain two more ways to say that shift spaces
![]() $(X_n)_{n=1}^{\infty} \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
approximate
$(X_n)_{n=1}^{\infty} \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
approximate
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
:
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
:
Note that we do not assume that the approximation in (2) and (3) is monotone (meaning
![]() $X_1\supseteq X_2 \supseteq \cdots $
and
$X_1\supseteq X_2 \supseteq \cdots $
and
![]() $X=\bigcap X_n$
), but in practice it is often the case.
$X=\bigcap X_n$
), but in practice it is often the case.
In [Reference Konieczny, Kupsa and Kwietniak23], we studied the consequences of the existence of an approximating sequence as in (2) and (3). We were especially interested in the case when the approximating sequence is the sequence of Markov approximations. We introduced
![]() $\bar {d}$
-approachable shift spaces (subshifts that are approached by their topological Markov approximations not only in the ‘usual’ Hausdorff metric topology, but also in the
$\bar {d}$
-approachable shift spaces (subshifts that are approached by their topological Markov approximations not only in the ‘usual’ Hausdorff metric topology, but also in the
![]() ${\bar d}^{\mathrm {H}}$
sense). We also considered a condition that is ostensibly a relaxation of (3):
${\bar d}^{\mathrm {H}}$
sense). We also considered a condition that is ostensibly a relaxation of (3):
We proved in [Reference Konieczny, Kupsa and Kwietniak23] that for every shift space X and Y over
![]() $\mathscr {A}$
we have
$\mathscr {A}$
we have
hence
In other words,
![]() ${\bar d}^{\mathrm {H}}$
approximation (2) implies convergence of simplices of invariant measures in the Hausdorff metric
${\bar d}^{\mathrm {H}}$
approximation (2) implies convergence of simplices of invariant measures in the Hausdorff metric
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
induced by Ornstein’s
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
induced by Ornstein’s
![]() $\bar {d}_{\mathcal {M}}$
metric on the space
$\bar {d}_{\mathcal {M}}$
metric on the space
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
as in (3). As a consequence, certain features of simplices of invariant measures of shift spaces in the approximating sequence are inherited by the simplex of the limit.
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
as in (3). As a consequence, certain features of simplices of invariant measures of shift spaces in the approximating sequence are inherited by the simplex of the limit.
In analogy with Friedman and Ornstein’s result characterizing Bernoulli measures among all totally ergodic shift-invariant measures as
![]() $\bar {d}_{\mathcal {M}}$
-limits of their own Markov approximations (see [Reference Friedman and Ornstein14]), we also characterized in [Reference Konieczny, Kupsa and Kwietniak23] chain-mixing
$\bar {d}_{\mathcal {M}}$
-limits of their own Markov approximations (see [Reference Friedman and Ornstein14]), we also characterized in [Reference Konieczny, Kupsa and Kwietniak23] chain-mixing
![]() $\bar {d}$
-approachable shift spaces using the newly introduced
$\bar {d}$
-approachable shift spaces using the newly introduced
![]() $\bar {d}$
-shadowing property. (Note that Ornstein’s theory characterizing Bernoullicity works best for invertible measure-preserving systems. In particular, Markov shifts carrying Markov approximations mentioned here are two-sided, while we consider the one-sided setting.) In this way we obtained a large family of shift spaces that contains all
$\bar {d}$
-shadowing property. (Note that Ornstein’s theory characterizing Bernoullicity works best for invertible measure-preserving systems. In particular, Markov shifts carrying Markov approximations mentioned here are two-sided, while we consider the one-sided setting.) In this way we obtained a large family of shift spaces that contains all
![]() $\beta $
-shifts and all mixing sofic shifts, in particular all mixing shifts of finite type. This is because many specification properties imply chain mixing and
$\beta $
-shifts and all mixing sofic shifts, in particular all mixing shifts of finite type. This is because many specification properties imply chain mixing and
![]() $\bar {d}$
-approachability (this is the case, for example, for all shift spaces with the almost specification property). We refer to [Reference Konieczny, Kupsa and Kwietniak23] for more details.
$\bar {d}$
-approachability (this is the case, for example, for all shift spaces with the almost specification property). We refer to [Reference Konieczny, Kupsa and Kwietniak23] for more details.
We also showed in [Reference Konieczny, Kupsa and Kwietniak23] that if every
![]() $X_n$
has an entropy-dense set of ergodic measures and the sequence
$X_n$
has an entropy-dense set of ergodic measures and the sequence
![]() $(X_n)_{n=1}^{\infty} $
converges to X in the sense defined by any of (2)–(4), then ergodic measures of X are also entropy dense. This established a new method of proving entropy density. As a consequence, we obtained entropy density of ergodic measures for all surjective shift spaces with the
$(X_n)_{n=1}^{\infty} $
converges to X in the sense defined by any of (2)–(4), then ergodic measures of X are also entropy dense. This established a new method of proving entropy density. As a consequence, we obtained entropy density of ergodic measures for all surjective shift spaces with the
![]() $\bar {d}$
-shadowing property. Entropy density of ergodic measures is a property introduced by Orey in 1986 [Reference Orey34] and Föllmer and Orey in 1988 [Reference Föllmer and Orey13]. Recall that ergodic measures of a shift space X are entropy dense if every invariant measure can be approximated with an ergodic one with respect to the weak
$\bar {d}$
-shadowing property. Entropy density of ergodic measures is a property introduced by Orey in 1986 [Reference Orey34] and Föllmer and Orey in 1988 [Reference Föllmer and Orey13]. Recall that ergodic measures of a shift space X are entropy dense if every invariant measure can be approximated with an ergodic one with respect to the weak
![]() $^*$
topology and entropy at the same time. In particular,
$^*$
topology and entropy at the same time. In particular,
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)$
is a dense subset of
${\mathcal {M}_{\mathit \sigma }}e(X)$
is a dense subset of
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
. Note that there are shift spaces with dense, but not entropy-dense, sets of ergodic measures (see [Reference Gelfert and Kwietniak16]). Density of ergodic measures and entropy density are strongly related to the theory of large deviations and multifractal analysis [Reference Comman5, Reference Eizenberg, Kifer and Weiss12, Reference Pfister and Sullivan38, Reference Pfister and Sullivan39]; see Comman’s article [Reference Comman6] and references therein for more information about that connection.
${\mathcal {M}_{\mathit \sigma }}(X)$
. Note that there are shift spaces with dense, but not entropy-dense, sets of ergodic measures (see [Reference Gelfert and Kwietniak16]). Density of ergodic measures and entropy density are strongly related to the theory of large deviations and multifractal analysis [Reference Comman5, Reference Eizenberg, Kifer and Weiss12, Reference Pfister and Sullivan38, Reference Pfister and Sullivan39]; see Comman’s article [Reference Comman6] and references therein for more information about that connection.
The results of [Reference Konieczny, Kupsa and Kwietniak23] are also applicable to the study of the dynamics of the so-called
![]() $\mathscr {B}$
-free shifts (or systems), a subject that has recently attracted considerable interest (see [Reference Dymek, Kasjan, Kułaga-Przymus and Lemańczyk10, Reference Dymek, Kułaga-Przymus and Sell11, Reference Kasjan, Keller and Lemańczyk19–Reference Konieczny, Kupsa and Kwietniak22, Reference Kułaga-Przymus, Lemańczyk and Weiss24–Reference Kułaga-Przymus and Lemańczyk26].
$\mathscr {B}$
-free shifts (or systems), a subject that has recently attracted considerable interest (see [Reference Dymek, Kasjan, Kułaga-Przymus and Lemańczyk10, Reference Dymek, Kułaga-Przymus and Sell11, Reference Kasjan, Keller and Lemańczyk19–Reference Konieczny, Kupsa and Kwietniak22, Reference Kułaga-Przymus, Lemańczyk and Weiss24–Reference Kułaga-Przymus and Lemańczyk26].
In the present paper, we study further properties of
![]() $\bar {d}$
-approachable shift spaces. In particular, in §5 we construct minimal and proximal examples of chain-mixing
$\bar {d}$
-approachable shift spaces. In particular, in §5 we construct minimal and proximal examples of chain-mixing
![]() $\bar {d}$
-approachable shift spaces. These examples demonstrate that our technique yields entropy density for shift spaces that are beyond the reach of methods based on specification, as specification excludes both proximality and minimality. So far only specification-like conditions have been invoked to prove entropy density explicitly (see [Reference Eizenberg, Kifer and Weiss12, Reference Pfister and Sullivan38]). We note that there exists a general theorem due to Downarowicz and Serafin [Reference Downarowicz and Serafin8] that guarantees existence of minimal shifts with entropy-dense ergodic measures, but due to its generality it is hard to see concrete examples. We also prove (see §3) that
$\bar {d}$
-approachable shift spaces. These examples demonstrate that our technique yields entropy density for shift spaces that are beyond the reach of methods based on specification, as specification excludes both proximality and minimality. So far only specification-like conditions have been invoked to prove entropy density explicitly (see [Reference Eizenberg, Kifer and Weiss12, Reference Pfister and Sullivan38]). We note that there exists a general theorem due to Downarowicz and Serafin [Reference Downarowicz and Serafin8] that guarantees existence of minimal shifts with entropy-dense ergodic measures, but due to its generality it is hard to see concrete examples. We also prove (see §3) that
![]() $\bar {d}$
-approachability implies
$\bar {d}$
-approachability implies
![]() $\bar {d}$
-stability, where
$\bar {d}$
-stability, where
![]() $\bar {d}$
-stability is a property recently introduced by Austin [Reference Austin1]. Austin combined one of Ornstein’s conditions equivalent to Bernoullicity with equivariant analogs of some basic results in measure concentration to characterize Bernoullicity of the equilibrium measure of a continuous potential
$\bar {d}$
-stability is a property recently introduced by Austin [Reference Austin1]. Austin combined one of Ornstein’s conditions equivalent to Bernoullicity with equivariant analogs of some basic results in measure concentration to characterize Bernoullicity of the equilibrium measure of a continuous potential
![]() $\varphi $
under the assumption that the equilibrium is unique. Austin formulated his main condition in terms of a stronger kind of differentiability of the pressure functional at
$\varphi $
under the assumption that the equilibrium is unique. Austin formulated his main condition in terms of a stronger kind of differentiability of the pressure functional at
![]() $\varphi $
. He proved that the condition is always necessary and he showed that it is sufficient if the shift space is ‘
$\varphi $
. He proved that the condition is always necessary and he showed that it is sufficient if the shift space is ‘
![]() $\bar {d}$
-stable’. Austin remarked that the class of ‘
$\bar {d}$
-stable’. Austin remarked that the class of ‘
![]() $\bar {d}$
-stable’ subshifts includes the full shift and several other examples with the specification property. He also suspected that
$\bar {d}$
-stable’ subshifts includes the full shift and several other examples with the specification property. He also suspected that
![]() $\bar {d}$
-stability holds also for examples without any specification properties. Hence, our minimal and proximal examples of
$\bar {d}$
-stability holds also for examples without any specification properties. Hence, our minimal and proximal examples of
![]() $\bar {d}$
-approachable shifts confirm this suspicion. In §4 we also show that the implication (3)
$\bar {d}$
-approachable shifts confirm this suspicion. In §4 we also show that the implication (3)
![]() $\implies $
(2) holds true if all shift spaces involved have the
$\implies $
(2) holds true if all shift spaces involved have the
![]() $\bar {d}$
-shadowing property, hence chain mixing and
$\bar {d}$
-shadowing property, hence chain mixing and
![]() $\bar {d}$
-approachability suffice for this implication; see Theorem 4.1. We note that the implication (3)
$\bar {d}$
-approachability suffice for this implication; see Theorem 4.1. We note that the implication (3)
![]() $\implies $
(2) does not hold in general by producing a sequence of shift spaces
$\implies $
(2) does not hold in general by producing a sequence of shift spaces
![]() $(X_n)_{n=1}^{\infty} $
such that for some shift space X we have
$(X_n)_{n=1}^{\infty} $
such that for some shift space X we have
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
while
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
while
![]() ${\bar d}^{\mathrm {H}}(X_n,X)\to 1$
as
${\bar d}^{\mathrm {H}}(X_n,X)\to 1$
as
![]() $n\to \infty $
; see Proposition 4.4.
$n\to \infty $
; see Proposition 4.4.
We prove (Proposition 4.2) that it is possible to find a sequence of shift spaces
![]() $(X_n)_{n=1}^{\infty} $
such that
$(X_n)_{n=1}^{\infty} $
such that
![]() ${\mathcal {M}_{\mathit \sigma }}(X_n)$
converges in
${\mathcal {M}_{\mathit \sigma }}(X_n)$
converges in
![]() $\bar {d}_{\mathcal {M}}$
to a singleton set that is not a simplex of invariant measures for any shift space. Finally, we prove that the
$\bar {d}_{\mathcal {M}}$
to a singleton set that is not a simplex of invariant measures for any shift space. Finally, we prove that the
![]() $\bar {d}$
-shadowing on the measure center of a shift space (the smallest invariant subshift of full measure for every invariant measure) implies the same for the shift. We recall our notation and basic definitions in §2.
$\bar {d}$
-shadowing on the measure center of a shift space (the smallest invariant subshift of full measure for every invariant measure) implies the same for the shift. We recall our notation and basic definitions in §2.
The results of the present paper, as well as the results of [Reference Konieczny, Kupsa and Kwietniak23], are also applicable to two-sided shift spaces (shift-invariant subsets of
![]() $\mathscr {A}^{\mathbb {Z}}$
), provided that the definition of the pseudometric
$\mathscr {A}^{\mathbb {Z}}$
), provided that the definition of the pseudometric
![]() $\bar {d}$
stays as in (1), that is, we average over the coordinates
$\bar {d}$
stays as in (1), that is, we average over the coordinates
![]() $0,1,\ldots ,n-1$
.
$0,1,\ldots ,n-1$
.
2. Definitions
2.1. Hausdorff pseudometrics
Let Z be a set. A pseudometric on Z is a real-valued, non-negative, symmetric function
![]() $\rho $
on
$\rho $
on
![]() $Z\times Z$
vanishing on the diagonal
$Z\times Z$
vanishing on the diagonal
![]() $\{(x,y)\in Z\times Z: x=y\}$
and satisfying the triangle inequality. Let
$\{(x,y)\in Z\times Z: x=y\}$
and satisfying the triangle inequality. Let
![]() $\rho $
be a bounded pseudometric on Z. For
$\rho $
be a bounded pseudometric on Z. For
![]() $z\in Z$
and non-empty
$z\in Z$
and non-empty
![]() $A,B\subseteq Z$
, we define
$A,B\subseteq Z$
, we define
We call
![]() $\rho ^{\mathrm {H}}$
the Hausdorff pseudometric induced by
$\rho ^{\mathrm {H}}$
the Hausdorff pseudometric induced by
![]() $\rho $
on the space of all non-empty subsets of Z. If
$\rho $
on the space of all non-empty subsets of Z. If
![]() $\rho $
is a bounded metric, then
$\rho $
is a bounded metric, then
![]() $\rho ^{\mathrm {H}}$
becomes a metric on the set
$\rho ^{\mathrm {H}}$
becomes a metric on the set
![]() $\operatorname {\mathrm {CL}}(Z,\rho )$
of closed non-empty subsets of
$\operatorname {\mathrm {CL}}(Z,\rho )$
of closed non-empty subsets of
![]() $(Z,\rho )$
. Note that in our settings some properties, well known in the compact case, fail because we consider
$(Z,\rho )$
. Note that in our settings some properties, well known in the compact case, fail because we consider
![]() $(Z,\rho )$
where
$(Z,\rho )$
where
![]() $\rho $
is not necessarily compact, but only a bounded pseudometric space. For example,
$\rho $
is not necessarily compact, but only a bounded pseudometric space. For example,
![]() $\rho $
and another pseudometric
$\rho $
and another pseudometric
![]() $\tilde \rho $
may induce the same topology on Z but the spaces
$\tilde \rho $
may induce the same topology on Z but the spaces
![]() $(\operatorname {\mathrm {CL}}(Z),\rho ^{\mathrm {H}})$
and
$(\operatorname {\mathrm {CL}}(Z),\rho ^{\mathrm {H}})$
and
![]() $(\operatorname {\mathrm {CL}}(Z),\tilde {\rho }^{\mathrm {H}})$
need not be homeomorphic.
$(\operatorname {\mathrm {CL}}(Z),\tilde {\rho }^{\mathrm {H}})$
need not be homeomorphic.
2.2. Shift spaces and languages
We let
![]() $\mathbb {N}$
denote the set of positive integers. We also write
$\mathbb {N}$
denote the set of positive integers. We also write
![]() $\mathbb {N}_0=\mathbb {N}\cup \{0\}$
. Unless otherwise stated, the letters
$\mathbb {N}_0=\mathbb {N}\cup \{0\}$
. Unless otherwise stated, the letters
![]() $i,j,k,l,m,n$
always denote integers. An alphabet is a finite set
$i,j,k,l,m,n$
always denote integers. An alphabet is a finite set
![]() $\mathscr {A}$
endowed with the discrete topology. We refer to elements of
$\mathscr {A}$
endowed with the discrete topology. We refer to elements of
![]() $\mathscr {A}$
as symbols or letters. The full shift
$\mathscr {A}$
as symbols or letters. The full shift
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
is the Cartesian product of infinitely many copies of
${\mathscr {A}^{\hspace{2pt}\infty} }$
is the Cartesian product of infinitely many copies of
![]() $\mathscr {A}$
indexed by
$\mathscr {A}$
indexed by
![]() $\mathbb {N}_0$
. We endow
$\mathbb {N}_0$
. We endow
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
with the product topology. A compatible metric on
${\mathscr {A}^{\hspace{2pt}\infty} }$
with the product topology. A compatible metric on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
is given for
${\mathscr {A}^{\hspace{2pt}\infty} }$
is given for
![]() $x,y\in {\mathscr {A}^{\hspace{2pt}\infty} }$
by
$x,y\in {\mathscr {A}^{\hspace{2pt}\infty} }$
by
 $$ \begin{align*} \rho(x,y)=\begin{cases} 0 & \mbox{if } x=y, \\ 2^{-\min\{j:x_j\neq y_j\}} & \mbox{otherwise}. \end{cases} \end{align*} $$
$$ \begin{align*} \rho(x,y)=\begin{cases} 0 & \mbox{if } x=y, \\ 2^{-\min\{j:x_j\neq y_j\}} & \mbox{otherwise}. \end{cases} \end{align*} $$
The shift map
![]() $\sigma \colon \mathscr {A}^{\hspace{2pt}\infty}\hspace{-0.5pt} \to\hspace{-0.5pt} \mathscr {A}^{\hspace{2pt}\infty} $
is given for
$\sigma \colon \mathscr {A}^{\hspace{2pt}\infty}\hspace{-0.5pt} \to\hspace{-0.5pt} \mathscr {A}^{\hspace{2pt}\infty} $
is given for
![]() $x=(x_i)_{i\hspace{-0.5pt}=\hspace{-0.5pt}0}^{\infty}\hspace{-0.5pt} \in\hspace{-0.5pt} {\mathscr {A}^{\hspace{2pt}\infty} }$
and
$x=(x_i)_{i\hspace{-0.5pt}=\hspace{-0.5pt}0}^{\infty}\hspace{-0.5pt} \in\hspace{-0.5pt} {\mathscr {A}^{\hspace{2pt}\infty} }$
and
![]() $j\hspace{-0.5pt}\ge\hspace{-0.5pt} 0$
by
$j\hspace{-0.5pt}\ge\hspace{-0.5pt} 0$
by
![]() $\sigma (x)_j\hspace{-0.5pt}=\hspace{-0.5pt}x_{j+1}$
. A shift space over
$\sigma (x)_j\hspace{-0.5pt}=\hspace{-0.5pt}x_{j+1}$
. A shift space over
![]() $\mathscr {A}$
is a non-empty, closed, and
$\mathscr {A}$
is a non-empty, closed, and
![]() $\sigma $
-invariant subset of
$\sigma $
-invariant subset of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. A word over
${\mathscr {A}^{\hspace{2pt}\infty} }$
. A word over
![]() $\mathscr {A}$
is a finite sequence of elements of
$\mathscr {A}$
is a finite sequence of elements of
![]() $\mathscr {A}$
. The number of entries of a word w is called the length of w and is denoted by
$\mathscr {A}$
. The number of entries of a word w is called the length of w and is denoted by
![]() $|w|$
. The empty sequence is called the empty word and is the only word of length
$|w|$
. The empty sequence is called the empty word and is the only word of length
![]() $0$
. We denote it by
$0$
. We denote it by
![]() $\unicode{x3bb} $
. The concatenation of words
$\unicode{x3bb} $
. The concatenation of words
![]() $u=u_1\cdots u_k$
and
$u=u_1\cdots u_k$
and
![]() $v=v_1\cdots v_m$
is the word
$v=v_1\cdots v_m$
is the word
![]() $u_1\cdots u_k v_1\cdots v_m$
denoted simply by
$u_1\cdots u_k v_1\cdots v_m$
denoted simply by
![]() $uv$
. Given
$uv$
. Given
![]() $x \in {\mathscr {A}^{\hspace{2pt}\infty} }$
and
$x \in {\mathscr {A}^{\hspace{2pt}\infty} }$
and
![]() $0\le i<j$
, we let
$0\le i<j$
, we let
![]() $x_{[i,j)}$
denote the word
$x_{[i,j)}$
denote the word
![]() $x_ix_{i+1}\cdots x_{j-1}$
over
$x_ix_{i+1}\cdots x_{j-1}$
over
![]() $\mathscr {A}$
of length
$\mathscr {A}$
of length
![]() $j-i$
. We say that a word w appears in
$j-i$
. We say that a word w appears in
![]() $x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
if there exist
$x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
if there exist
![]() $0\le i<j$
such that
$0\le i<j$
such that
![]() $w=x_{[i,j)}$
. A word w appears in a shift space
$w=x_{[i,j)}$
. A word w appears in a shift space
![]() $X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
if there exists
$X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
if there exists
![]() $x\in X$
such that w appears in x. The language of a shift space
$x\in X$
such that w appears in x. The language of a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is the set
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is the set
![]() $\mathcal {B}(X)$
of all finite words over
$\mathcal {B}(X)$
of all finite words over
![]() $\mathscr {A}$
appearing in X. We agree that the empty word appears in every sequence in
$\mathscr {A}$
appearing in X. We agree that the empty word appears in every sequence in
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. For
${\mathscr {A}^{\hspace{2pt}\infty} }$
. For
![]() $n\in \mathbb {N}_0$
, we let
$n\in \mathbb {N}_0$
, we let
![]() $\mathcal {B}_n(X) \subseteq \mathscr {A}^n$
be the set of all words
$\mathcal {B}_n(X) \subseteq \mathscr {A}^n$
be the set of all words
![]() $w\in \mathcal {B}(X)$
with
$w\in \mathcal {B}(X)$
with
![]() $|w|=n$
. Given a set
$|w|=n$
. Given a set
![]() $\mathscr {F}$
of finite words over
$\mathscr {F}$
of finite words over
![]() $\mathscr {A}$
, we define
$\mathscr {A}$
, we define
![]() $X_{\mathscr {F}}$
be the set of all
$X_{\mathscr {F}}$
be the set of all
![]() $x=(x_i)_{i=0}^{\infty} \in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that no word from
$x=(x_i)_{i=0}^{\infty} \in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that no word from
![]() $\mathscr {F}$
appears in x. The resulting set
$\mathscr {F}$
appears in x. The resulting set
![]() $X_{\mathscr {F}}$
is either empty or a shift space. Furthermore, for every shift space X over
$X_{\mathscr {F}}$
is either empty or a shift space. Furthermore, for every shift space X over
![]() $\mathscr {A}$
one can find a collection
$\mathscr {A}$
one can find a collection
![]() $\mathscr {F}$
of finite words such that
$\mathscr {F}$
of finite words such that
![]() $X=X_{\mathscr {F}}$
. A shift space X is a shift of finite type if there exists a finite set
$X=X_{\mathscr {F}}$
. A shift space X is a shift of finite type if there exists a finite set
![]() $\mathscr {F}$
such that
$\mathscr {F}$
such that
![]() $X=X_{\mathscr {F}}$
. Every shift space
$X=X_{\mathscr {F}}$
. Every shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is the intersection of a sequence
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is the intersection of a sequence
![]() $(X^M_n)_{n\ge 0}$
of shifts of finite type. To construct that sequence, we define
$(X^M_n)_{n\ge 0}$
of shifts of finite type. To construct that sequence, we define
![]() $\mathscr {F}[n]$
to consist of all words w over
$\mathscr {F}[n]$
to consist of all words w over
![]() $\mathscr {A}$
with
$\mathscr {A}$
with
![]() $|w|= n+1$
and
$|w|= n+1$
and
![]() $w\notin \mathcal {B}_{n+1}(X)$
. In this way, we obtain for each
$w\notin \mathcal {B}_{n+1}(X)$
. In this way, we obtain for each
![]() $n\ge 0$
a shift of finite type
$n\ge 0$
a shift of finite type
![]() $X^M_n=X_{\mathscr {F}[n]}$
such that
$X^M_n=X_{\mathscr {F}[n]}$
such that
![]() $\mathcal {B}_{j}(X)=\mathcal {B}_j(X^M_n)$
for
$\mathcal {B}_{j}(X)=\mathcal {B}_j(X^M_n)$
for
![]() $0\le j\le n+1$
. We call the shift space
$0\le j\le n+1$
. We call the shift space
![]() $X^M_n$
the nth (topological) Markov approximation of X or finite type approximation of order n to X. We note that for every shift space X, its Markov approximation
$X^M_n$
the nth (topological) Markov approximation of X or finite type approximation of order n to X. We note that for every shift space X, its Markov approximation
![]() $X^M_n$
can be conveniently described using a Rauzy graph. The nth Rauzy graph of X is a labeled graph
$X^M_n$
can be conveniently described using a Rauzy graph. The nth Rauzy graph of X is a labeled graph
![]() $G_n=(V_n,E_n,\tau _n)$
, where we set
$G_n=(V_n,E_n,\tau _n)$
, where we set
![]() $V_n=\mathcal {B}_n(X)$
and
$V_n=\mathcal {B}_n(X)$
and
![]() $E_n=\mathcal {B}_{n+1}(X)$
, and for each
$E_n=\mathcal {B}_{n+1}(X)$
, and for each
![]() $w=w_0w_1\cdots w_{n}\in E_n$
we define
$w=w_0w_1\cdots w_{n}\in E_n$
we define
![]() $i(w)=w_0\cdots w_{n-1}\in V_n$
,
$i(w)=w_0\cdots w_{n-1}\in V_n$
,
![]() $t(w)=w_1\cdots w_{n}\in V_n$
, and
$t(w)=w_1\cdots w_{n}\in V_n$
, and
![]() $\tau _n(w)=w_0\in \mathscr {A}$
. The sofic shift space
$\tau _n(w)=w_0\in \mathscr {A}$
. The sofic shift space
![]() $X_n$
presented by
$X_n$
presented by
![]() $G_n$
satisfies
$G_n$
satisfies
![]() $\mathcal {B}_{j}(X_n)=\mathcal {B}_{j}(X)$
for
$\mathcal {B}_{j}(X_n)=\mathcal {B}_{j}(X)$
for
![]() $j=1,\ldots ,n+1$
; see Proposition 3.62 in [Reference Kůrka28]. It is now easy to see that
$j=1,\ldots ,n+1$
; see Proposition 3.62 in [Reference Kůrka28]. It is now easy to see that
![]() $X_n$
is the nth topological Markov approximations for X.
$X_n$
is the nth topological Markov approximations for X.
The following definitions of (chain) mixing and (chain) transitivity are stated only for shift spaces. We will do the same for several notions: instead of presenting a general definition for continuous maps acting on compact metric spaces (for the latter, see [Reference Kůrka28]), we will state an equivalent definition adapted to symbolic dynamics. This applies to (chain) transitivity, (chain) mixing, specification, and its variants.
A shift space X is transitive if for every
![]() $u,w\in \mathcal {B}(X)$
there exists v with
$u,w\in \mathcal {B}(X)$
there exists v with
![]() $uvw\in \mathcal {B}(X)$
. A shift space X is topologically mixing if for any
$uvw\in \mathcal {B}(X)$
. A shift space X is topologically mixing if for any
![]() $u,w\in \mathcal {B}(X)$
there exists
$u,w\in \mathcal {B}(X)$
there exists
![]() $N\in \mathbb {N}_0$
such that for each
$N\in \mathbb {N}_0$
such that for each
![]() $n\ge N$
there is
$n\ge N$
there is
![]() $v=v(n)\in \mathcal {B}_n(X)$
such that
$v=v(n)\in \mathcal {B}_n(X)$
such that
![]() $uvw\in \mathcal {B}(X)$
.
$uvw\in \mathcal {B}(X)$
.
A shift space is chain transitive (respectively, chain mixing) if its topological Markov approximations
![]() $X^M_n$
are transitive (respectively, topologically mixing) for all except finitely many ns.
$X^M_n$
are transitive (respectively, topologically mixing) for all except finitely many ns.
2.3. Ergodic properties of shift spaces
Let
![]() $\mathcal {M}(X)$
be the set of all Borel probability measures supported on a shift space
$\mathcal {M}(X)$
be the set of all Borel probability measures supported on a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
. In particular,
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
. In particular,
![]() $\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
stands for the space of all Borel probability measures on
$\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
stands for the space of all Borel probability measures on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. We write
${\mathscr {A}^{\hspace{2pt}\infty} }$
. We write
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
and
${\mathcal {M}_{\mathit \sigma }}(X)$
and
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)$
to denote respectively the sets of
${\mathcal {M}_{\mathit \sigma }}e(X)$
to denote respectively the sets of
![]() $\sigma $
-invariant and ergodic
$\sigma $
-invariant and ergodic
![]() $\sigma $
-invariant measures in
$\sigma $
-invariant measures in
![]() $\mathcal {M}(X)$
. We endow
$\mathcal {M}(X)$
. We endow
![]() $\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
with the weak
$\mathcal {M}({\mathscr {A}^{\hspace{2pt}\infty} })$
with the weak
![]() $^*$
topology, hence it becomes a compact metrizable space and
$^*$
topology, hence it becomes a compact metrizable space and
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
is its closed subset for every shift space
${\mathcal {M}_{\mathit \sigma }}(X)$
is its closed subset for every shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
.
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
.
We say that
![]() $x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
generates
$x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
generates
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
along a strictly increasing sequence of integers
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
along a strictly increasing sequence of integers
![]() $(N_k)_{k=1}^{\infty} $
, if for every continuous function
$(N_k)_{k=1}^{\infty} $
, if for every continuous function
![]() $f\colon X\to \mathbb {R}$
the sequence of Cesàro averages of
$f\colon X\to \mathbb {R}$
the sequence of Cesàro averages of
![]() $(f(\sigma ^n(x)))_{n=0}^{\infty} $
along
$(f(\sigma ^n(x)))_{n=0}^{\infty} $
along
![]() $(N_k)_{k=1}^{\infty} $
converges and the limit satisfies
$(N_k)_{k=1}^{\infty} $
converges and the limit satisfies
 $$ \begin{align*} \lim_{k\to\infty}\frac{1}{N_k}\sum_{n=0}^{N_k-1}f(\sigma^n(x))=\int_{{\mathscr{A}^{\hspace{2pt}\infty}}}f\,\textit{d}\mu. \end{align*} $$
$$ \begin{align*} \lim_{k\to\infty}\frac{1}{N_k}\sum_{n=0}^{N_k-1}f(\sigma^n(x))=\int_{{\mathscr{A}^{\hspace{2pt}\infty}}}f\,\textit{d}\mu. \end{align*} $$
Compactness implies that for every strictly increasing sequence of integers
![]() $(N_k)_{k=1}^{\infty} $
and every point
$(N_k)_{k=1}^{\infty} $
and every point
![]() $x\in X$
there is a subsequence of
$x\in X$
there is a subsequence of
![]() $(N_k)_{k=1}^{\infty} $
such that x generates an invariant measure along that subsequence. In particular, every point always generates at least one measure. A point
$(N_k)_{k=1}^{\infty} $
such that x generates an invariant measure along that subsequence. In particular, every point always generates at least one measure. A point
![]() $x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
is generic for
$x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
is generic for
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
if
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
if
![]() $\mu $
is the unique measure generated by x. Every ergodic measure has a generic point. We denote by
$\mu $
is the unique measure generated by x. Every ergodic measure has a generic point. We denote by
![]() $h(\mu )$
the Kolmogorov–Sinai entropy of
$h(\mu )$
the Kolmogorov–Sinai entropy of
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
. We say that ergodic measures of a shift space X are entropy dense if for every measure
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
. We say that ergodic measures of a shift space X are entropy dense if for every measure
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
, every neighborhood U of
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
, every neighborhood U of
![]() $\mu $
in
$\mu $
in
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
, and every
${\mathcal {M}_{\mathit \sigma }}(X)$
, and every
![]() $\varepsilon>0$
there is
$\varepsilon>0$
there is
![]() $\nu \in U\cap {\mathcal {M}_{\mathit \sigma }}e(X)$
with
$\nu \in U\cap {\mathcal {M}_{\mathit \sigma }}e(X)$
with
![]() $|h(\nu )-h(\mu )|<\varepsilon $
. Note that having entropy-dense ergodic measures is preserved by conjugacy.
$|h(\nu )-h(\mu )|<\varepsilon $
. Note that having entropy-dense ergodic measures is preserved by conjugacy.
2.4. The functions
 $\bar {d}$
and
$\bar {d}$
and
 $\bar {d}_{\mathcal {M}}$
$\bar {d}_{\mathcal {M}}$
Given
![]() $x=(x_n)_{n=0}^{\infty} ,y=(y_n)_{n=0}^{\infty} \in {\mathscr {A}^{\hspace{2pt}\infty} }$
, we set
$x=(x_n)_{n=0}^{\infty} ,y=(y_n)_{n=0}^{\infty} \in {\mathscr {A}^{\hspace{2pt}\infty} }$
, we set
The function
![]() $\bar {d}$
is a pseudometric on
$\bar {d}$
is a pseudometric on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
, but
${\mathscr {A}^{\hspace{2pt}\infty} }$
, but
![]() $\bar {d}$
is not a metric if
$\bar {d}$
is not a metric if
![]() $\mathscr {A}$
has at least two elements, because the implication
$\mathscr {A}$
has at least two elements, because the implication
![]() $\bar {d}(x,y)=0\implies x=y$
fails. The function
$\bar {d}(x,y)=0\implies x=y$
fails. The function
![]() $\bar {d}$
is not continuous in general. Furthermore,
$\bar {d}$
is not continuous in general. Furthermore,
![]() $\bar {d}\colon {\mathscr {A}^{\hspace{2pt}\infty} }\times {\mathscr {A}^{\hspace{2pt}\infty} }\to [0,1]$
is shift invariant (for all
$\bar {d}\colon {\mathscr {A}^{\hspace{2pt}\infty} }\times {\mathscr {A}^{\hspace{2pt}\infty} }\to [0,1]$
is shift invariant (for all
![]() $x,y\in {\mathscr {A}^{\hspace{2pt}\infty} }$
we have
$x,y\in {\mathscr {A}^{\hspace{2pt}\infty} }$
we have
![]() $\bar {d}(x,y)=\bar {d}(\sigma (x),\sigma (y))$
).
$\bar {d}(x,y)=\bar {d}(\sigma (x),\sigma (y))$
).
Ornstein’s metric
![]() $\bar {d}_{\mathcal {M}}$
on
$\bar {d}_{\mathcal {M}}$
on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
is usually defined with the help of joinings. (Ornstein’s metric
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
is usually defined with the help of joinings. (Ornstein’s metric
![]() $\bar {d}_{\mathcal {M}}$
is usually denoted by
$\bar {d}_{\mathcal {M}}$
is usually denoted by
![]() $\bar {d}$
, but in [Reference Konieczny, Kupsa and Kwietniak23] as well as in this paper the distinction between
$\bar {d}$
, but in [Reference Konieczny, Kupsa and Kwietniak23] as well as in this paper the distinction between
![]() $\bar {d}$
and
$\bar {d}$
and
![]() $\bar {d}_{\mathcal {M}}$
is crucial. We refer to
$\bar {d}_{\mathcal {M}}$
is crucial. We refer to
![]() $\bar {d}_{\mathcal {M}}$
as the ‘d-bar distance for measures’ and we call
$\bar {d}_{\mathcal {M}}$
as the ‘d-bar distance for measures’ and we call
![]() $\bar {d}$
on
$\bar {d}$
on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
‘pointwise d-bar’ or simply ‘d-bar’.) A
${\mathscr {A}^{\hspace{2pt}\infty} }$
‘pointwise d-bar’ or simply ‘d-bar’.) A
![]() $\sigma \times \sigma $
-invariant measure
$\sigma \times \sigma $
-invariant measure
![]() $\xi $
on
$\xi $
on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }\times {\mathscr {A}^{\hspace{2pt}\infty} }$
is a joining of
${\mathscr {A}^{\hspace{2pt}\infty} }\times {\mathscr {A}^{\hspace{2pt}\infty} }$
is a joining of
![]() $\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
if
$\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
if
![]() $\mu $
and
$\mu $
and
![]() $\nu $
are the marginal measures for
$\nu $
are the marginal measures for
![]() $\xi $
under the projection to the first (respectively, the second) coordinate. We write
$\xi $
under the projection to the first (respectively, the second) coordinate. We write
![]() $J(\mu ,\nu )$
for the set of all joinings of
$J(\mu ,\nu )$
for the set of all joinings of
![]() $\mu $
and
$\mu $
and
![]() $\nu $
. Note that
$\nu $
. Note that
![]() $J(\mu ,\nu )$
is always non-empty because the product measure
$J(\mu ,\nu )$
is always non-empty because the product measure
![]() $\mu\hspace{-0.5pt} \times\hspace{-0.5pt} \nu $
belongs to
$\mu\hspace{-0.5pt} \times\hspace{-0.5pt} \nu $
belongs to
![]() $J(\mu,\hspace{-0.5pt} \nu )$
. Ornstein’s metric
$J(\mu,\hspace{-0.5pt} \nu )$
. Ornstein’s metric
![]() $\bar {d}_{\mathcal {M}}$
on
$\bar {d}_{\mathcal {M}}$
on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
is given by
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
is given by
where
![]() $d_0(x,y)=1$
if
$d_0(x,y)=1$
if
![]() $x_0\neq y_0$
and
$x_0\neq y_0$
and
![]() $d_0(x,y)=0$
otherwise. The space
$d_0(x,y)=0$
otherwise. The space
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
endowed with the
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
endowed with the
![]() $\bar {d}_{\mathcal {M}}$
-metric becomes a complete but non-separable (hence, non-compact) metric space. The space
$\bar {d}_{\mathcal {M}}$
-metric becomes a complete but non-separable (hence, non-compact) metric space. The space
![]() ${\mathcal {M}_{\mathit \sigma }}e({\mathscr {A}^{\hspace{2pt}\infty} })\subseteq {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
of ergodic measures is
${\mathcal {M}_{\mathit \sigma }}e({\mathscr {A}^{\hspace{2pt}\infty} })\subseteq {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
of ergodic measures is
![]() $\bar {d}_{\mathcal {M}}$
-closed, as are the spaces of strongly mixing and Bernoulli measures on
$\bar {d}_{\mathcal {M}}$
-closed, as are the spaces of strongly mixing and Bernoulli measures on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
. The entropy function
${\mathscr {A}^{\hspace{2pt}\infty} }$
. The entropy function
![]() $\mu \mapsto h(\mu )$
is continuous on
$\mu \mapsto h(\mu )$
is continuous on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
under
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
under
![]() $\bar {d}_{\mathcal {M}}$
. The convergence in
$\bar {d}_{\mathcal {M}}$
. The convergence in
![]() $\bar {d}_{\mathcal {M}}$
implies weak
$\bar {d}_{\mathcal {M}}$
implies weak
![]() $^*$
convergence (for more details, see [Reference Ornstein35]).
$^*$
convergence (for more details, see [Reference Ornstein35]).
Applying Hausdorff metric construction described in §2.1 to the bounded metric space
![]() $({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
, we obtain a metric denoted by
$({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
, we obtain a metric denoted by
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
defined on the space
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
defined on the space
![]() $\operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
of non-empty closed subsets of
$\operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
of non-empty closed subsets of
![]() $({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
. For every shift space X the sets
$({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
. For every shift space X the sets
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
and
${\mathcal {M}_{\mathit \sigma }}(X)$
and
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)$
are closed sets in the Hausdorff metric
${\mathcal {M}_{\mathit \sigma }}e(X)$
are closed sets in the Hausdorff metric
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
. Similarly, starting from the pseudometric space
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
. Similarly, starting from the pseudometric space
![]() $({\mathscr {A}^{\hspace{2pt}\infty} },\bar {d})$
, we get a pseudometric
$({\mathscr {A}^{\hspace{2pt}\infty} },\bar {d})$
, we get a pseudometric
![]() ${\bar d}^{\mathrm {H}}$
on the set of all non-empty subsets of
${\bar d}^{\mathrm {H}}$
on the set of all non-empty subsets of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
.
${\mathscr {A}^{\hspace{2pt}\infty} }$
.
2.5. On
 $\bar {d}$
-shadowing and
$\bar {d}$
-shadowing and
 $\bar {d}$
-approachability
$\bar {d}$
-approachability
A shift space
![]() $X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is
$X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is
![]() $\bar {d}$
-approachable if its Markov approximations
$\bar {d}$
-approachable if its Markov approximations
![]() $X^M_1,X^M_2,\ldots $
satisfy
$X^M_1,X^M_2,\ldots $
satisfy
![]() ${\bar d}^{\mathrm {H}}(X^M_n,X)\to 0$
as
${\bar d}^{\mathrm {H}}(X^M_n,X)\to 0$
as
![]() $n\to \infty $
. Every shift of finite type is
$n\to \infty $
. Every shift of finite type is
![]() $\bar {d}$
-approachable.
$\bar {d}$
-approachable.
We say that the shift space X has the
![]() $\bar {d}$
-shadowing property if for every
$\bar {d}$
-shadowing property if for every
![]() $\varepsilon>0$
there is
$\varepsilon>0$
there is
![]() $N\in \mathbb {N}$
such that every sequence
$N\in \mathbb {N}$
such that every sequence
![]() $(w^{(j)})_{j=1}^{\infty} $
in
$(w^{(j)})_{j=1}^{\infty} $
in
![]() $\mathcal {B}(X)$
with
$\mathcal {B}(X)$
with
![]() $|w^{(j)}|\ge N$
for
$|w^{(j)}|\ge N$
for
![]() $j=1,2,\ldots $
is
$j=1,2,\ldots $
is
![]() $\varepsilon $
-traced by some point
$\varepsilon $
-traced by some point
![]() $x'\in X$
, that is, there is
$x'\in X$
, that is, there is
![]() $x'\in X$
such that
$x'\in X$
such that
![]() $\bar {d}(x,x')<\varepsilon $
, where
$\bar {d}(x,x')<\varepsilon $
, where
![]() $x=w^{(1)}w^{(2)}w^{(3)}\ldots .$
The
$x=w^{(1)}w^{(2)}w^{(3)}\ldots .$
The
![]() $\bar {d}$
-shadowing property was introduced in [Reference Konieczny, Kupsa and Kwietniak23]. It is closely related to the average shadowing property introduced by Blank [Reference Blank3] and studied in [Reference Kulczycki, Kwietniak and Oprocha27]. Every mixing sofic shift space has the
$\bar {d}$
-shadowing property was introduced in [Reference Konieczny, Kupsa and Kwietniak23]. It is closely related to the average shadowing property introduced by Blank [Reference Blank3] and studied in [Reference Kulczycki, Kwietniak and Oprocha27]. Every mixing sofic shift space has the
![]() $\bar {d}$
-shadowing property, and
$\bar {d}$
-shadowing property, and
![]() $\bar {d}$
-shadowing is inherited by
$\bar {d}$
-shadowing is inherited by
![]() ${\bar d}^{\mathrm {H}}$
-limits of shift spaces with the
${\bar d}^{\mathrm {H}}$
-limits of shift spaces with the
![]() $\bar {d}$
-shadowing. Note that
$\bar {d}$
-shadowing. Note that
![]() $\bar {d}$
-shadowing implies
$\bar {d}$
-shadowing implies
![]() $\bar {d}$
-approachability, but to prove the converse we need to assume additionally that the shift space in question is chain mixing. The exact statement is Theorem 6 in [Reference Konieczny, Kupsa and Kwietniak23], which says that a shift space
$\bar {d}$
-approachability, but to prove the converse we need to assume additionally that the shift space in question is chain mixing. The exact statement is Theorem 6 in [Reference Konieczny, Kupsa and Kwietniak23], which says that a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is chain mixing and
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is chain mixing and
![]() $\bar {d}$
-approachable if and only if
$\bar {d}$
-approachable if and only if
![]() $\sigma (X)=X$
and X has the
$\sigma (X)=X$
and X has the
![]() $\bar {d}$
-shadowing property. Theorem 6 in [Reference Konieczny, Kupsa and Kwietniak23] lists a third condition, but we will not need it until §5, so we postpone the exact statement.
$\bar {d}$
-shadowing property. Theorem 6 in [Reference Konieczny, Kupsa and Kwietniak23] lists a third condition, but we will not need it until §5, so we postpone the exact statement.
As we have already mentioned in the introduction, this characterization is a topological counterpart of the result saying that a totally ergodic shift-invariant probability measure is Bernoulli if and only if it is the
![]() $\bar {d}_{\mathcal {M}}$
-limit of the sequence of its canonical Markov approximations.
$\bar {d}_{\mathcal {M}}$
-limit of the sequence of its canonical Markov approximations.
In §5.1, we also show how to apply this corollary even in the case when natural approximations of our shift are not comparable via inclusion, hence do not form a descending chain of shift spaces.
3.
 $\bar {d}$
-approachability vs.
$\bar {d}$
-approachability vs.
 $\bar {d}$
-stability
$\bar {d}$
-stability
We recall the notion of
![]() $\bar {d}_{\mathcal {M}}$
-stability that has been recently introduced by Austin [Reference Austin1]. Then we show that it follows from the
$\bar {d}_{\mathcal {M}}$
-stability that has been recently introduced by Austin [Reference Austin1]. Then we show that it follows from the
![]() $\bar {d}$
-shadowing property. Later we discuss some further properties of
$\bar {d}$
-shadowing property. Later we discuss some further properties of
![]() $\bar {d}_{\mathcal {M}}$
-stable shifts.
$\bar {d}_{\mathcal {M}}$
-stable shifts.
Definition 3.1. A shift space
![]() $X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is
$X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is
![]() $\bar {d}_{\mathcal {M}}$
-stable if for every
$\bar {d}_{\mathcal {M}}$
-stable if for every
![]() $\varepsilon>0$
there is an open neighborhood
$\varepsilon>0$
there is an open neighborhood
![]() $\mathscr {U}$
of
$\mathscr {U}$
of
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
in the weak
${\mathcal {M}_{\mathit \sigma }}(X)$
in the weak
![]() $^*$
topology on
$^*$
topology on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
such that if
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
such that if
![]() $\nu \in \mathscr {U}$
, then there is
$\nu \in \mathscr {U}$
, then there is
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
with
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
with
![]() $\bar {d}_{\mathcal {M}}(\mu ,\nu )<\varepsilon $
.
$\bar {d}_{\mathcal {M}}(\mu ,\nu )<\varepsilon $
.
Note that we use slightly different notation: Austin writes
![]() $\bar {d}$
instead of
$\bar {d}$
instead of
![]() $\bar {d}_{\mathcal {M}}$
. Equivalently, a shift space X is
$\bar {d}_{\mathcal {M}}$
. Equivalently, a shift space X is
![]() $\bar {d}_{\mathcal {M}}$
-stable if any shift-invariant measure which lives close enough to X in the weak
$\bar {d}_{\mathcal {M}}$
-stable if any shift-invariant measure which lives close enough to X in the weak
![]() $^*$
topology is actually close in Ornstein’s
$^*$
topology is actually close in Ornstein’s
![]() $\bar {d}_{\mathcal {M}}$
metric on
$\bar {d}_{\mathcal {M}}$
metric on
![]() ${\mathcal {M}_{\mathit \sigma }}(\mathscr {A}^{\hspace{2pt}\infty} )$
to a shift-invariant measure supported on X. This observation (noted already in [Reference Austin1]) is formulated as the next lemma for future reference. We state it in terms of the natural basis
${\mathcal {M}_{\mathit \sigma }}(\mathscr {A}^{\hspace{2pt}\infty} )$
to a shift-invariant measure supported on X. This observation (noted already in [Reference Austin1]) is formulated as the next lemma for future reference. We state it in terms of the natural basis
![]() $(U_n)_{n=1}^{\infty} $
of open neighborhoods of a shift space X with respect to the Hausdorff topology induced by the usual (product) topology on
$(U_n)_{n=1}^{\infty} $
of open neighborhoods of a shift space X with respect to the Hausdorff topology induced by the usual (product) topology on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
(the topology of the Hausdorff metric
${\mathscr {A}^{\hspace{2pt}\infty} }$
(the topology of the Hausdorff metric
![]() $\rho ^{\mathrm {H}}$
), where for
$\rho ^{\mathrm {H}}$
), where for
![]() $n\ge 1$
we have
$n\ge 1$
we have
Lemma 3.2. A shift space
![]() $X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is
$X \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is
![]() $\bar {d}_{\mathcal {M}}$
-stable if, and only if, for any
$\bar {d}_{\mathcal {M}}$
-stable if, and only if, for any
![]() $\varepsilon> 0$
there are
$\varepsilon> 0$
there are
![]() $\delta> 0$
and
$\delta> 0$
and
![]() $N\in \mathbb {N}$
such that
$N\in \mathbb {N}$
such that
![]() $\bar {d}_{\mathcal {M}}(\nu ,{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
whenever
$\bar {d}_{\mathcal {M}}(\nu ,{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
whenever
![]() $\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
,
$\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
,
![]() $n\ge N$
, and
$n\ge N$
, and
![]() $\nu (U_n(X))>1-\delta $
.
$\nu (U_n(X))>1-\delta $
.
Proposition 3.3. If a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
has the
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
has the
![]() $\bar {d}$
-shadowing property, then X is
$\bar {d}$
-shadowing property, then X is
![]() $\bar {d}_{\mathcal {M}}$
-stable.
$\bar {d}_{\mathcal {M}}$
-stable.
Proof. Fix
![]() $1>\varepsilon >0$
. Use the definition of the
$1>\varepsilon >0$
. Use the definition of the
![]() $\bar {d}$
-shadowing property to pick n such that every sequence
$\bar {d}$
-shadowing property to pick n such that every sequence
![]() $(w^{(j)})_{j=1}^{\infty} $
in
$(w^{(j)})_{j=1}^{\infty} $
in
![]() $\mathcal {B}(X)$
with
$\mathcal {B}(X)$
with
![]() $|w^{(j)}|\ge n$
for every
$|w^{(j)}|\ge n$
for every
![]() $j\ge 1$
is
$j\ge 1$
is
![]() $\varepsilon /2$
-traced in the
$\varepsilon /2$
-traced in the
![]() $\bar {d}$
pseudometric by some point in X. Let
$\bar {d}$
pseudometric by some point in X. Let
![]() $\nu $
be a shift-invariant measure on
$\nu $
be a shift-invariant measure on
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
such that
${\mathscr {A}^{\hspace{2pt}\infty} }$
such that
![]() $\nu (U_n(X))>1-\varepsilon /2$
. We would like to show that
$\nu (U_n(X))>1-\varepsilon /2$
. We would like to show that
![]() $\bar {d}_{\mathcal {M}}(\nu ,{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
.
$\bar {d}_{\mathcal {M}}(\nu ,{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
.
Let
![]() $y=(y_i)_{i=0}^{\infty} \in \mathscr {A}^{\hspace{2pt}\infty} $
be a generic point for
$y=(y_i)_{i=0}^{\infty} \in \mathscr {A}^{\hspace{2pt}\infty} $
be a generic point for
![]() $\nu $
(such a point always exists in
$\nu $
(such a point always exists in
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
). Hence, the frequency of visits of y to in
${\mathscr {A}^{\hspace{2pt}\infty} }$
). Hence, the frequency of visits of y to in
![]() $U_n(X)$
satisfies
$U_n(X)$
satisfies
Define
![]() $m_0\ge 0$
as the smallest
$m_0\ge 0$
as the smallest
![]() $\ell \ge 0$
such that
$\ell \ge 0$
such that
![]() $y_{[\ell ,\ell +n)}\in \mathcal {B}_n(X)$
. Inductively, given
$y_{[\ell ,\ell +n)}\in \mathcal {B}_n(X)$
. Inductively, given
![]() $m_k$
for some
$m_k$
for some
![]() $k\ge 0$
, we define
$k\ge 0$
, we define
![]() $m_{k+1}$
as the smallest integer
$m_{k+1}$
as the smallest integer
![]() $\ell \ge m_k+n$
such that
$\ell \ge m_k+n$
such that
![]() $y_{[\ell ,\ell +n)}\in \mathcal {B}_n(X)$
. Then the set
$y_{[\ell ,\ell +n)}\in \mathcal {B}_n(X)$
. Then the set
 $$ \begin{align*}M=\mathbb{N}_0\setminus\bigcup_{k=0}^{\infty} [m_k,m_{k+1})\end{align*} $$
$$ \begin{align*}M=\mathbb{N}_0\setminus\bigcup_{k=0}^{\infty} [m_k,m_{k+1})\end{align*} $$
is contained in the set
so its upper density is less than
![]() $\varepsilon /2$
. For
$\varepsilon /2$
. For
![]() $k\in \mathbb {N}_0$
, we extend the word
$k\in \mathbb {N}_0$
, we extend the word
![]() $y_{[m_k,m_{k}+n)}\in \mathcal {B}_n(X)$
to the right to form some word
$y_{[m_k,m_{k}+n)}\in \mathcal {B}_n(X)$
to the right to form some word
![]() $v^{(k)}\in \mathcal {B}(X)$
of length
$v^{(k)}\in \mathcal {B}(X)$
of length
![]() $m_{k+1}-m_k$
. Then the sequence
$m_{k+1}-m_k$
. Then the sequence
differs from y only at positions (indices) belonging to the set M. Hence,
![]() $\bar {d}(y,z)\le \varepsilon /2$
. The same is true for
$\bar {d}(y,z)\le \varepsilon /2$
. The same is true for
![]() $y'=\sigma ^{m_0}(y)$
and
$y'=\sigma ^{m_0}(y)$
and
![]() $z'=\sigma ^{m_0}(z)$
. Furthermore,
$z'=\sigma ^{m_0}(z)$
. Furthermore,
![]() $y'$
is still generic for
$y'$
is still generic for
![]() $\nu $
. By the
$\nu $
. By the
![]() $\bar {d}$
-shadowing property,
$\bar {d}$
-shadowing property,
can be approximated by
![]() $z"\in X$
such that
$z"\in X$
such that
![]() $\bar {d}(z',z")<\varepsilon /2$
. Therefore,
$\bar {d}(z',z")<\varepsilon /2$
. Therefore,
It is a standard fact (see [Reference Glasner17, Theorem 15.23]) that every measure generated by
![]() $z"$
is
$z"$
is
![]() $\varepsilon $
-close with respect to the
$\varepsilon $
-close with respect to the
![]() $\bar {d}_{\mathcal {M}}$
distance to the measure generated by
$\bar {d}_{\mathcal {M}}$
distance to the measure generated by
![]() $y'$
. Hence
$y'$
. Hence
![]() $\bar {d}_{\mathcal {M}}(\nu ,{\mathcal {M}_{\mathit \sigma }}(X))\le \varepsilon $
.
$\bar {d}_{\mathcal {M}}(\nu ,{\mathcal {M}_{\mathit \sigma }}(X))\le \varepsilon $
.
Shift spaces with the specification property are primary examples of
![]() $\bar {d}$
-stable shift spaces provided by [Reference Austin1]. Recall that a shift space
$\bar {d}$
-stable shift spaces provided by [Reference Austin1]. Recall that a shift space
![]() $X\subset {\mathscr {A}^{\hspace{2pt}\infty} }$
has the specification property if there exists
$X\subset {\mathscr {A}^{\hspace{2pt}\infty} }$
has the specification property if there exists
![]() $k\in \mathbb {N}$
such that for any
$k\in \mathbb {N}$
such that for any
![]() $u,w\in \mathcal {B}(X)$
there is v with
$u,w\in \mathcal {B}(X)$
there is v with
![]() $|v|=k$
such that
$|v|=k$
such that
![]() $uvw\in \mathcal {B}(X)$
. The specification property is a very useful property with many consequences for a shift space. For a more extensive overview on the specification property and its relatives we refer the reader to [Reference Kwietniak, Łącka and Oprocha30]. Here we note that the specification property and even either one of its two weaker, incommensurable variants known as the almost specification property or the weak specification property (see [Reference Kwietniak, Łącka and Oprocha30]) imply
$uvw\in \mathcal {B}(X)$
. The specification property is a very useful property with many consequences for a shift space. For a more extensive overview on the specification property and its relatives we refer the reader to [Reference Kwietniak, Łącka and Oprocha30]. Here we note that the specification property and even either one of its two weaker, incommensurable variants known as the almost specification property or the weak specification property (see [Reference Kwietniak, Łącka and Oprocha30]) imply
![]() $\bar {d}$
-approachability and chain mixing, hence
$\bar {d}$
-approachability and chain mixing, hence
![]() $\bar {d}$
-shadowing [Reference Konieczny, Kupsa and Kwietniak23]. Therefore, we obtain the following corollary.
$\bar {d}$
-shadowing [Reference Konieczny, Kupsa and Kwietniak23]. Therefore, we obtain the following corollary.
Corollary 3.4. Let X be a shift space satisfying one of the following conditions:
-
(1) X has the specification property;
-
(2) X has the weak specification property;
-
(3) X has the almost specification property.
Then X is
![]() $\bar {d}_{\mathcal {M}}$
-stable.
$\bar {d}_{\mathcal {M}}$
-stable.
Note that the nomenclature is not fixed; we follow [Reference Kwietniak, Łącka and Oprocha30]. Recall that a mistake function is a non-decreasing function
![]() $g\colon \mathbb {N}\to \mathbb {N}$
with
$g\colon \mathbb {N}\to \mathbb {N}$
with
![]() $g(n)/n\to 0$
as
$g(n)/n\to 0$
as
![]() $n\to \infty $
. We say that a shift space X has the almost specification property if there is a mistake function
$n\to \infty $
. We say that a shift space X has the almost specification property if there is a mistake function
![]() $g\colon \mathbb {N}\to \mathbb {N}$
such that for any
$g\colon \mathbb {N}\to \mathbb {N}$
such that for any
![]() $u,w\in \mathcal {B}(X)$
there is a word
$u,w\in \mathcal {B}(X)$
there is a word
![]() $v\in \mathcal {B}(X)$
satisfying
$v\in \mathcal {B}(X)$
satisfying
![]() $v=u'w'$
, where
$v=u'w'$
, where
![]() $|u|=|u'|$
,
$|u|=|u'|$
,
![]() $|v|=|v'|$
, and the following inequalities hold:
$|v|=|v'|$
, and the following inequalities hold:
 $$ \begin{align*} |\{1\le j\le n: u_j\neq u^{\prime}_j\}|&\le g(|u|),\\ |\{1\le j\le n: w_j\neq w^{\prime}_j\}|&\le g(|w|). \end{align*} $$
$$ \begin{align*} |\{1\le j\le n: u_j\neq u^{\prime}_j\}|&\le g(|u|),\\ |\{1\le j\le n: w_j\neq w^{\prime}_j\}|&\le g(|w|). \end{align*} $$
We say that a shift space X has the weak specification property if there is a mistake function g such that for any
![]() $u,w\in \mathcal {B}(X)$
there is v with
$u,w\in \mathcal {B}(X)$
there is v with
![]() $uvw\in \mathcal {B}(X)$
satisfying
$uvw\in \mathcal {B}(X)$
satisfying
![]() $|v|=g(|w|)$
. The weak and almost specification properties are independent of each other: neither one implies the other (see [Reference Kwietniak, Oprocha and Rams31]). Additionally, in contrast to the classical specification property, the weaker versions do not imply the uniqueness of the measure of maximal entropy (see [Reference Kwietniak, Oprocha and Rams31, Reference Pavlov37]).
$|v|=g(|w|)$
. The weak and almost specification properties are independent of each other: neither one implies the other (see [Reference Kwietniak, Oprocha and Rams31]). Additionally, in contrast to the classical specification property, the weaker versions do not imply the uniqueness of the measure of maximal entropy (see [Reference Kwietniak, Oprocha and Rams31, Reference Pavlov37]).
Remark 3.5. Using Proposition 3.3, we see that the proximal shift space constructed in Example 5.4 and the minimal shift space from §5.2 (see Theorem 5.11) are
![]() $\bar {d}_{\mathcal {M}}$
-stable. This answers Austin’s question affirmatively. We note that these examples do not have any of the specification properties mentioned in Corollary 3.4, because any of these specification properties implies that a shift having one of them and positive topological entropy has many disjoint minimal proper subsets. Hence, such a shift space is neither minimal nor proximal.
$\bar {d}_{\mathcal {M}}$
-stable. This answers Austin’s question affirmatively. We note that these examples do not have any of the specification properties mentioned in Corollary 3.4, because any of these specification properties implies that a shift having one of them and positive topological entropy has many disjoint minimal proper subsets. Hence, such a shift space is neither minimal nor proximal.
We list certain properties of
![]() $\bar {d}_{\mathcal {M}}$
-stable shift spaces. But first we recall some definitions. Let
$\bar {d}_{\mathcal {M}}$
-stable shift spaces. But first we recall some definitions. Let
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
be a shift space. The measure center of X is the smallest shift space
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
be a shift space. The measure center of X is the smallest shift space
![]() $X^+\subseteq X$
such that
$X^+\subseteq X$
such that
![]() $\mu (X^+)=1$
for every
$\mu (X^+)=1$
for every
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
. In other words,
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
. In other words,
![]() $X^+$
is the smallest subshift of X containing supports of all invariant measures on X. The measure center is determined by the language of all words in
$X^+$
is the smallest subshift of X containing supports of all invariant measures on X. The measure center is determined by the language of all words in
![]() $\mathcal {B}(X)$
whose cylinders have positive measure for at least one measure in
$\mathcal {B}(X)$
whose cylinders have positive measure for at least one measure in
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
, that is,
${\mathcal {M}_{\mathit \sigma }}(X)$
, that is,
The following observation follows directly from the definitions.
Proposition 3.6. A shift space X is
![]() $\bar {d}_{\mathcal {M}}$
-stable if and only if its measure center
$\bar {d}_{\mathcal {M}}$
-stable if and only if its measure center
![]() $X^+$
is
$X^+$
is
![]() $\bar {d}_{\mathcal {M}}$
-stable.
$\bar {d}_{\mathcal {M}}$
-stable.
It is possible that
![]() $X^+=X$
holds true for every
$X^+=X$
holds true for every
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space. In the next proposition, we note that
$\bar {d}_{\mathcal {M}}$
-stable shift space. In the next proposition, we note that
![]() $\bar {d}_{\mathcal {M}}$
-stability of X implies that the canonical Markov approximations of X form a sequence of shift spaces satisfying (3). That is,
$\bar {d}_{\mathcal {M}}$
-stability of X implies that the canonical Markov approximations of X form a sequence of shift spaces satisfying (3). That is,
![]() $\bar {d}_{\mathcal {M}}$
-stability implies that X has a property we call
$\bar {d}_{\mathcal {M}}$
-stability implies that X has a property we call
![]() $\bar {d}_{\mathcal {M}}$
-approachability.
$\bar {d}_{\mathcal {M}}$
-approachability.
Proposition 3.7. If
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space, then
$\bar {d}_{\mathcal {M}}$
-stable shift space, then
Proof. Fix
![]() $\varepsilon>0$
. Let
$\varepsilon>0$
. Let
![]() $\delta>0$
and
$\delta>0$
and
![]() $n\ge 1$
be such that if
$n\ge 1$
be such that if
![]() $\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
and
$\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
and
![]() $\nu (U_n(X))>1-\delta $
, then
$\nu (U_n(X))>1-\delta $
, then
![]() $\bar {d}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X),\nu )<\varepsilon $
. Fix
$\bar {d}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X),\nu )<\varepsilon $
. Fix
![]() $m\ge n$
and
$m\ge n$
and
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X^M_m)$
. Since
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X^M_m)$
. Since
![]() $X^M_m\subseteq U_n(X)$
we see that
$X^M_m\subseteq U_n(X)$
we see that
![]() $\bar {d}_{\mathcal {M}}(\mu ,{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
, so
$\bar {d}_{\mathcal {M}}(\mu ,{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
, so
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_m),{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
for
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_m),{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon $
for
![]() $m\ge n$
. Hence,
$m\ge n$
. Hence,
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
![]() $n\to \infty $
.
$n\to \infty $
.
The converse is not true, that is, the condition
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
![]() $n\to \infty $
does not imply
$n\to \infty $
does not imply
![]() $\bar {d}_{\mathcal {M}}$
-stability. Any shift of finite type X with non-transitive
$\bar {d}_{\mathcal {M}}$
-stability. Any shift of finite type X with non-transitive
![]() $X^+$
is a counterexample. A concrete example is the shift space X over
$X^+$
is a counterexample. A concrete example is the shift space X over
![]() $\{0,1\}$
consisting of only two fixed points
$\{0,1\}$
consisting of only two fixed points
![]() $0^{\infty} $
and
$0^{\infty} $
and
![]() $1^{\infty} $
. It is a binary shift of finite type with
$1^{\infty} $
. It is a binary shift of finite type with
![]() $01$
and
$01$
and
![]() $10$
as the forbidden words. Such a shift space (trivially) satisfies
$10$
as the forbidden words. Such a shift space (trivially) satisfies
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
![]() $n\to \infty $
, but is not
$n\to \infty $
, but is not
![]() $\bar {d}_{\mathcal {M}}$
-stable because of Proposition 3.9 below. Nevertheless, adding an assumption of chain mixing to
$\bar {d}_{\mathcal {M}}$
-stable because of Proposition 3.9 below. Nevertheless, adding an assumption of chain mixing to
![]() $\bar {d}_{\mathcal {M}}$
-approachability, we obtain
$\bar {d}_{\mathcal {M}}$
-approachability, we obtain
![]() $\bar {d}_{\mathcal {M}}$
-stability.
$\bar {d}_{\mathcal {M}}$
-stability.
Proposition 3.8. If
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a chain-mixing shift space with
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a chain-mixing shift space with
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n), {\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n), {\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
![]() $n\to \infty $
, then X is
$n\to \infty $
, then X is
![]() $\bar {d}_{\mathcal {M}}$
-stable.
$\bar {d}_{\mathcal {M}}$
-stable.
Proof. Fix
![]() $\varepsilon>0$
. Find
$\varepsilon>0$
. Find
![]() $N>0$
such that
$N>0$
such that
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_N),{\mathcal {M}_{\mathit \sigma }}(X))\le \varepsilon /2$
. Since X is chain mixing,
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_N),{\mathcal {M}_{\mathit \sigma }}(X))\le \varepsilon /2$
. Since X is chain mixing,
![]() $X^M_N$
is a topologically mixing shift of finite type so it also has the
$X^M_N$
is a topologically mixing shift of finite type so it also has the
![]() $\bar {d}$
-shadowing property (see [Reference Konieczny, Kupsa and Kwietniak23]). Hence,
$\bar {d}$
-shadowing property (see [Reference Konieczny, Kupsa and Kwietniak23]). Hence,
![]() $X^M_N$
is
$X^M_N$
is
![]() $\bar {d}_{\mathcal {M}}$
-stable by Proposition 3.3. Let
$\bar {d}_{\mathcal {M}}$
-stable by Proposition 3.3. Let
![]() $\delta>0$
and
$\delta>0$
and
![]() $m\ge 1$
be such that if
$m\ge 1$
be such that if
![]() $\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
and
$\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
and
![]() $\nu (U_m(X^M_N))>1-\delta $
, then
$\nu (U_m(X^M_N))>1-\delta $
, then
![]() $\bar {d}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_N^M),\nu )<\varepsilon /2$
. Without loss of generality we have
$\bar {d}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_N^M),\nu )<\varepsilon /2$
. Without loss of generality we have
![]() $m\ge N$
, so
$m\ge N$
, so
![]() $U_m(X)\subseteq U_m(X^M_N)$
. Let
$U_m(X)\subseteq U_m(X^M_N)$
. Let
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}e({\mathscr {A}^{\hspace{2pt}\infty} })$
be such that
$\mu \in {\mathcal {M}_{\mathit \sigma }}e({\mathscr {A}^{\hspace{2pt}\infty} })$
be such that
![]() $\mu (U_m(X))>1-\delta $
. Then
$\mu (U_m(X))>1-\delta $
. Then
![]() $\mu (U_m(X^M_N))\ge \mu (U_m(X))>1-\delta $
. Hence,
$\mu (U_m(X^M_N))\ge \mu (U_m(X))>1-\delta $
. Hence,
![]() $\bar {d}_{\mathcal {M}}(\mu ,{\mathcal {M}_{\mathit \sigma }}(X^M_N))<\varepsilon /2$
, so there exists
$\bar {d}_{\mathcal {M}}(\mu ,{\mathcal {M}_{\mathit \sigma }}(X^M_N))<\varepsilon /2$
, so there exists
![]() $\mu '\in {\mathcal {M}_{\mathit \sigma }}e(X^M_N)$
such that
$\mu '\in {\mathcal {M}_{\mathit \sigma }}e(X^M_N)$
such that
![]() $\bar {d}_{\mathcal {M}}(\mu ,\mu ')<\varepsilon /2$
. Since
$\bar {d}_{\mathcal {M}}(\mu ,\mu ')<\varepsilon /2$
. Since
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_N),{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon /2$
there exists
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_N),{\mathcal {M}_{\mathit \sigma }}(X))<\varepsilon /2$
there exists
![]() $\xi \in {\mathcal {M}_{\mathit \sigma }}e(X)$
with
$\xi \in {\mathcal {M}_{\mathit \sigma }}e(X)$
with
![]() $\bar {d}_{\mathcal {M}}(\xi ,\mu )\le \bar {d}_{\mathcal {M}}(\xi ,\mu ')+\bar {d}_{\mathcal {M}}(\mu ',\mu )<\varepsilon $
.
$\bar {d}_{\mathcal {M}}(\xi ,\mu )\le \bar {d}_{\mathcal {M}}(\xi ,\mu ')+\bar {d}_{\mathcal {M}}(\mu ',\mu )<\varepsilon $
.
We do not know if
![]() $\bar {d}_{\mathcal {M}}$
-stability implies the stronger condition called
$\bar {d}_{\mathcal {M}}$
-stability implies the stronger condition called
![]() $\bar {d}$
-approachability (the sequence of canonical Markov approximations of our shift space is a sequence of shift spaces satisfying condition (2)), even if we assume that the shift space is chain transitive or chain mixing. The examples discussed in Proposition 4.4 suggest that it might not be the case.
$\bar {d}$
-approachability (the sequence of canonical Markov approximations of our shift space is a sequence of shift spaces satisfying condition (2)), even if we assume that the shift space is chain transitive or chain mixing. The examples discussed in Proposition 4.4 suggest that it might not be the case.
Next, we note that
![]() $\bar {d}_{\mathcal {M}}$
-stability implies weak
$\bar {d}_{\mathcal {M}}$
-stability implies weak
![]() $^*$
density of ergodic measures in
$^*$
density of ergodic measures in
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
and, as a consequence, entropy density (interestingly, we need to prove weak
${\mathcal {M}_{\mathit \sigma }}(X)$
and, as a consequence, entropy density (interestingly, we need to prove weak
![]() $^*$
density first to obtain transitivity of
$^*$
density first to obtain transitivity of
![]() $X^+$
and obtain entropy density as a consequence of transitivity of
$X^+$
and obtain entropy density as a consequence of transitivity of
![]() $X^+$
). Hence, if X is a
$X^+$
). Hence, if X is a
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space then the simplex of invariant measures
$\bar {d}_{\mathcal {M}}$
-stable shift space then the simplex of invariant measures
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
is either a Poulsen simplex or a singleton. Note that the latter possibility can occur. As an example, take
${\mathcal {M}_{\mathit \sigma }}(X)$
is either a Poulsen simplex or a singleton. Note that the latter possibility can occur. As an example, take
![]() $X=\{0^{\infty} \}$
.
$X=\{0^{\infty} \}$
.
Proposition 3.9. If
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space, then
$\bar {d}_{\mathcal {M}}$
-stable shift space, then
![]() $X^+$
is transitive and ergodic measures are entropy dense in
$X^+$
is transitive and ergodic measures are entropy dense in
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
.
${\mathcal {M}_{\mathit \sigma }}(X)$
.
Proof. We first prove that
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)$
is weak
${\mathcal {M}_{\mathit \sigma }}e(X)$
is weak
![]() $^*$
dense in
$^*$
dense in
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
. To prove the density of ergodic measures it is enough to show that for every
${\mathcal {M}_{\mathit \sigma }}(X)$
. To prove the density of ergodic measures it is enough to show that for every
![]() $\mu _1,\mu _2\in {\mathcal {M}_{\mathit \sigma }}e(X)$
the measure
$\mu _1,\mu _2\in {\mathcal {M}_{\mathit \sigma }}e(X)$
the measure
![]() $\tfrac 12(\mu _1+\mu _2)$
is a limit of a sequence of ergodic measures in
$\tfrac 12(\mu _1+\mu _2)$
is a limit of a sequence of ergodic measures in
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)$
. Fix
${\mathcal {M}_{\mathit \sigma }}e(X)$
. Fix
![]() $\varepsilon>0$
. Let
$\varepsilon>0$
. Let
![]() $\delta>0$
and
$\delta>0$
and
![]() $n\ge 1$
be such that if
$n\ge 1$
be such that if
![]() $\mu (U_n(X))>1-\delta $
, then
$\mu (U_n(X))>1-\delta $
, then
![]() $\bar {d}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X),\mu )<\varepsilon $
. Since the ergodic measures of
$\bar {d}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X),\mu )<\varepsilon $
. Since the ergodic measures of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
are dense in
${\mathscr {A}^{\hspace{2pt}\infty} }$
are dense in
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
, when the latter space is endowed with the weak
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
, when the latter space is endowed with the weak
![]() $^*$
topology we can find
$^*$
topology we can find
![]() $\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
with
$\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
with
![]() $D(\tfrac 12(\mu _1+\mu _2),\nu )$
as small as necessary. Here D stands for any metric on
$D(\tfrac 12(\mu _1+\mu _2),\nu )$
as small as necessary. Here D stands for any metric on
![]() ${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
compatible with the weak
${\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
compatible with the weak
![]() $^*$
topology. In particular, we may assume that
$^*$
topology. In particular, we may assume that
![]() $D(\tfrac 12(\mu _1+\mu _2),\nu )$
is sufficiently small to guarantee
$D(\tfrac 12(\mu _1+\mu _2),\nu )$
is sufficiently small to guarantee
![]() $\nu (U_n(X))>1-\delta $
. By
$\nu (U_n(X))>1-\delta $
. By
![]() $\bar {d}_{\mathcal {M}}$
-stability, there is
$\bar {d}_{\mathcal {M}}$
-stability, there is
![]() $\xi \in {\mathcal {M}_{\mathit \sigma }}(X)$
such that
$\xi \in {\mathcal {M}_{\mathit \sigma }}(X)$
such that
![]() $\bar {d}_{\mathcal {M}}(\nu ,\xi )<\varepsilon $
. Since
$\bar {d}_{\mathcal {M}}(\nu ,\xi )<\varepsilon $
. Since
![]() $\nu $
is ergodic, we can assure that
$\nu $
is ergodic, we can assure that
![]() $\xi $
is an ergodic measure. By the triangle inequality,
$\xi $
is an ergodic measure. By the triangle inequality,
![]() $D(\tfrac 12(\mu _1+\mu _2),\xi )\le D(\tfrac 12(\mu _1+\mu _2),\nu )+D(\nu ,\xi )$
. Since
$D(\tfrac 12(\mu _1+\mu _2),\xi )\le D(\tfrac 12(\mu _1+\mu _2),\nu )+D(\nu ,\xi )$
. Since
![]() $\nu $
and
$\nu $
and
![]() $\xi $
can be arbitrarily close in
$\xi $
can be arbitrarily close in
![]() $\bar {d}_{\mathcal {M}}$
, they can also be arbitrarily close in D. Hence,
$\bar {d}_{\mathcal {M}}$
, they can also be arbitrarily close in D. Hence,
![]() $\xi $
can be arbitrarily close to
$\xi $
can be arbitrarily close to
![]() $\tfrac 12(\mu _1+\mu _2)$
in D and the ergodic measures must be weak
$\tfrac 12(\mu _1+\mu _2)$
in D and the ergodic measures must be weak
![]() $^*$
dense. Now, transitivity of
$^*$
dense. Now, transitivity of
![]() $X^+$
follows easily from weak
$X^+$
follows easily from weak
![]() $^*$
density of ergodic measures (see Proposition 6.4 in [Reference Gelfert and Kwietniak16] for details). By Proposition 3.6 the measure center
$^*$
density of ergodic measures (see Proposition 6.4 in [Reference Gelfert and Kwietniak16] for details). By Proposition 3.6 the measure center
![]() $X^+$
is a
$X^+$
is a
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space. Now, Proposition 3.7 implies
$\bar {d}_{\mathcal {M}}$
-stable shift space. Now, Proposition 3.7 implies
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}((X^+)^M_n),{\mathcal {M}_{\mathit \sigma }}(X^+))\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}((X^+)^M_n),{\mathcal {M}_{\mathit \sigma }}(X^+))\to 0$
as
![]() $n\to \infty $
. Transitivity of
$n\to \infty $
. Transitivity of
![]() $X^+$
implies that its Markov approximations are entropy-dense shift spaces. Hence, ergodic measures are entropy dense in
$X^+$
implies that its Markov approximations are entropy-dense shift spaces. Hence, ergodic measures are entropy dense in
![]() ${\mathcal {M}_{\mathit \sigma }}(X^+)$
by [Reference Konieczny, Kupsa and Kwietniak23], but clearly
${\mathcal {M}_{\mathit \sigma }}(X^+)$
by [Reference Konieczny, Kupsa and Kwietniak23], but clearly
![]() ${\mathcal {M}_{\mathit \sigma }}(X)={\mathcal {M}_{\mathit \sigma }}(X^+)$
.
${\mathcal {M}_{\mathit \sigma }}(X)={\mathcal {M}_{\mathit \sigma }}(X^+)$
.
Proposition 3.10. If X is a strictly ergodic
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space, then the unique invariant measure on X is isomorphic to an odometer.
$\bar {d}_{\mathcal {M}}$
-stable shift space, then the unique invariant measure on X is isomorphic to an odometer.
Proof. Assume that X is a strictly ergodic infinite shift space. Let
![]() $\nu $
be its unique ergodic invariant measure, that is,
$\nu $
be its unique ergodic invariant measure, that is,
![]() ${\mathcal {M}_{\mathit \sigma }}(X)=\{\nu \}$
. Hence, for every
${\mathcal {M}_{\mathit \sigma }}(X)=\{\nu \}$
. Hence, for every
![]() $n\ge 1$
the Markov approximation
$n\ge 1$
the Markov approximation
![]() $X_n^M$
of X is an uncountable shift of finite type. In particular, for every
$X_n^M$
of X is an uncountable shift of finite type. In particular, for every
![]() $n\ge 1$
the simplex
$n\ge 1$
the simplex
![]() ${\mathcal {M}_{\mathit \sigma }}(X_n^M)$
contains infinitely many periodic ergodic invariant measures (measures concentrated on periodic orbits). Now assume that X is
${\mathcal {M}_{\mathit \sigma }}(X_n^M)$
contains infinitely many periodic ergodic invariant measures (measures concentrated on periodic orbits). Now assume that X is
![]() $\bar {d}_{\mathcal {M}}$
-stable, so
$\bar {d}_{\mathcal {M}}$
-stable, so
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X^M_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
as
![]() $n\to \infty $
. It follows that
$n\to \infty $
. It follows that
![]() $\lim _{n\to \infty }\bar {d}_{\mathcal {M}}(\mu ^{\text {per}}_n,\nu )=0$
for any choice of periodic ergodic measures
$\lim _{n\to \infty }\bar {d}_{\mathcal {M}}(\mu ^{\text {per}}_n,\nu )=0$
for any choice of periodic ergodic measures
![]() $\mu _n^{\text {per}}\in {\mathcal {M}_{\mathit \sigma }}(X^M_n)$
. In particular, one may take measures on periodic points whose primary periods tend to infinity. A measure that is a
$\mu _n^{\text {per}}\in {\mathcal {M}_{\mathit \sigma }}(X^M_n)$
. In particular, one may take measures on periodic points whose primary periods tend to infinity. A measure that is a
![]() $\bar {d}_{\mathcal {M}}$
-limit of such a sequence of periodic measures must be isomorphic to a Haar measure on some odometer (this result is implicit in [Reference Rudolph and Schwarz40] and follows directly from [Reference Bergelson, Kułaga-Przymus, Lemańczyk and Richter2, Theorem 1.7]; it does not need the invertibility assumption).
$\bar {d}_{\mathcal {M}}$
-limit of such a sequence of periodic measures must be isomorphic to a Haar measure on some odometer (this result is implicit in [Reference Rudolph and Schwarz40] and follows directly from [Reference Bergelson, Kułaga-Przymus, Lemańczyk and Richter2, Theorem 1.7]; it does not need the invertibility assumption).
Remark 3.11. (Some open questions)
The results in the present section do not provide a complete picture of connections between the notions of
![]() $\bar {d}_{\mathcal {M}}$
-stability and
$\bar {d}_{\mathcal {M}}$
-stability and
![]() $\bar {d}$
-approachability. For example, we were unable to answer the following questions. First, can a non-trivial periodic orbit be
$\bar {d}$
-approachability. For example, we were unable to answer the following questions. First, can a non-trivial periodic orbit be
![]() $\bar {d}_{\mathcal {M}}$
-stable shift space? Second, can a strictly ergodic infinite shift space be
$\bar {d}_{\mathcal {M}}$
-stable shift space? Second, can a strictly ergodic infinite shift space be
![]() $\bar {d}_{\mathcal {M}}$
-stable? Third, is every
$\bar {d}_{\mathcal {M}}$
-stable? Third, is every
![]() $\bar {d}_{\mathcal {M}}$
-stable system topologically mixing on its measure center? Finally, can a shift space X such that
$\bar {d}_{\mathcal {M}}$
-stable system topologically mixing on its measure center? Finally, can a shift space X such that
![]() $X^+\neq X$
be
$X^+\neq X$
be
![]() $\bar {d}_{\mathcal {M}}$
-stable?
$\bar {d}_{\mathcal {M}}$
-stable?
4. Comparing
 $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
with
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
with
 ${\bar d}^{\mathrm {H}}$
${\bar d}^{\mathrm {H}}$
If
![]() $(Z,\rho )$
is a bounded complete metric space, then so is
$(Z,\rho )$
is a bounded complete metric space, then so is
![]() $(\operatorname {\mathrm {CL}}(Z),\rho ^{\mathrm {H}})$
(see [Reference Illanes and Nadler18, §2.15]). Hence, the Hausdorff metric
$(\operatorname {\mathrm {CL}}(Z),\rho ^{\mathrm {H}})$
(see [Reference Illanes and Nadler18, §2.15]). Hence, the Hausdorff metric
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
induced on
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
induced on
![]() $\operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }))$
by
$\operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }))$
by
![]() $\bar {d}_{\mathcal {M}}$
is complete and the Cauchy condition provides a criterion for convergence of a sequence
$\bar {d}_{\mathcal {M}}$
is complete and the Cauchy condition provides a criterion for convergence of a sequence
![]() $({\mathcal {M}_{\mathit \sigma }}(X_k))_{k=1}^{\infty} $
, where
$({\mathcal {M}_{\mathit \sigma }}(X_k))_{k=1}^{\infty} $
, where
![]() $X_k\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a shift space for every
$X_k\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is a shift space for every
![]() $k\ge 1$
. But even if we know that
$k\ge 1$
. But even if we know that
![]() $({\mathcal {M}_{\mathit \sigma }}(X_k))_{k=1}^{\infty} $
converges in
$({\mathcal {M}_{\mathit \sigma }}(X_k))_{k=1}^{\infty} $
converges in
![]() $\bar {d}_{\mathcal {M}}$
to some
$\bar {d}_{\mathcal {M}}$
to some
![]() $\mathcal {M}\in \operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
, it is not clear if there exists a shift space
$\mathcal {M}\in \operatorname {\mathrm {CL}}({\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} }),\bar {d}_{\mathcal {M}})$
, it is not clear if there exists a shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
such that
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
such that
![]() $\mathcal {M}={\mathcal {M}_{\mathit \sigma }}(X)$
. We provide an example showing that this need not be the case at the end of this section. But first we demonstrate that shift spaces X and Y with the
$\mathcal {M}={\mathcal {M}_{\mathit \sigma }}(X)$
. We provide an example showing that this need not be the case at the end of this section. But first we demonstrate that shift spaces X and Y with the
![]() $\bar {d}$
-shadowing property are
$\bar {d}$
-shadowing property are
![]() ${\bar d}^{\mathrm {H}}$
close if and only if they are
${\bar d}^{\mathrm {H}}$
close if and only if they are
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
close. On the other hand, without the
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
close. On the other hand, without the
![]() $\bar {d}$
-shadowing property the inequality in (5) can be strict. We use a variant of Oxtoby’s construction of non-uniquely ergodic minimal Toeplitz subshift to show that for every
$\bar {d}$
-shadowing property the inequality in (5) can be strict. We use a variant of Oxtoby’s construction of non-uniquely ergodic minimal Toeplitz subshift to show that for every
![]() $\delta>0$
there are shift spaces X and Y such that
$\delta>0$
there are shift spaces X and Y such that
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X), {\mathcal {M}_{\mathit \sigma }}(Y))<\delta $
but
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X), {\mathcal {M}_{\mathit \sigma }}(Y))<\delta $
but
![]() ${\bar d}^{\mathrm {H}}(X,Y)>1-\delta $
. Finally, we show that if the measure center of a shift space X has the
${\bar d}^{\mathrm {H}}(X,Y)>1-\delta $
. Finally, we show that if the measure center of a shift space X has the
![]() $\bar {d}$
-shadowing property, then so also does X.
$\bar {d}$
-shadowing property, then so also does X.
Theorem 4.1. If X and Y are shift spaces over
![]() $\mathscr {A}$
with the
$\mathscr {A}$
with the
![]() $\bar {d}$
-shadowing property such that
$\bar {d}$
-shadowing property such that
for some
![]() $\varepsilon>0$
, then
$\varepsilon>0$
, then
![]() $\bar {d}^{\mathrm {H}}(X,Y)<7\varepsilon $
.
$\bar {d}^{\mathrm {H}}(X,Y)<7\varepsilon $
.
Proof. Fix
![]() $x\in X$
. Use
$x\in X$
. Use
![]() $\bar {d}$
-shadowing of Y to find
$\bar {d}$
-shadowing of Y to find
![]() $s\in \mathbb {N}$
such that for every sequence
$s\in \mathbb {N}$
such that for every sequence
![]() $\{ w^{(j)} \}_{j=1}^{\infty} $
of words in
$\{ w^{(j)} \}_{j=1}^{\infty} $
of words in
![]() $\mathcal {B}(Y)$
with
$\mathcal {B}(Y)$
with
![]() $|w^{(j)}|\ge s$
for every
$|w^{(j)}|\ge s$
for every
![]() $j\geq 1$
, there exists
$j\geq 1$
, there exists
![]() $y\in Y$
such that
$y\in Y$
such that
Pick m such that
![]() $s<m\varepsilon $
. By [Reference Downarowicz and Więcek9, Theorem 3.4] we find
$s<m\varepsilon $
. By [Reference Downarowicz and Więcek9, Theorem 3.4] we find
![]() $l\geq m$
such that x can be decomposed into an infinite concatenation of blocks, that is, we can write
$l\geq m$
such that x can be decomposed into an infinite concatenation of blocks, that is, we can write
and the blocks
![]() $A^{(1)}, A^{(2)}, \ldots $
and
$A^{(1)}, A^{(2)}, \ldots $
and
![]() $B^{(1)},B^{(2)},\ldots $
satisfy the following properties.
$B^{(1)},B^{(2)},\ldots $
satisfy the following properties.
-
• For every
 $i\geq 1$
we have
$i\geq 1$
we have
 $m\leq |B^{(i)}| \leq l$
.
$m\leq |B^{(i)}| \leq l$
. -
• For every
 $i\geq 1$
there exists an ergodic measure
$i\geq 1$
there exists an ergodic measure
 $\mu ^{(i)}\in {\mathcal {M}_{\mathit \sigma }}e(X)$
such that (8)where
$\mu ^{(i)}\in {\mathcal {M}_{\mathit \sigma }}e(X)$
such that (8)where $$ \begin{align} d^*(B^{(i)},\mu^{(i)})=\sum_{k=1}^{\infty}2^{-k}\sum_{w\in\mathscr{A}^k} |\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])|<\frac{\varepsilon}{2^{s}}, \end{align} $$
(9)
$$ \begin{align} d^*(B^{(i)},\mu^{(i)})=\sum_{k=1}^{\infty}2^{-k}\sum_{w\in\mathscr{A}^k} |\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])|<\frac{\varepsilon}{2^{s}}, \end{align} $$
(9) $$ \begin{align} \operatorname{\mathrm{freq}}(w,B^{(i)})=\begin{cases}\frac{|\{1\le j \le |B^{(i)}|-l+1 \ : \ B^{(i)}_{[j,j+l)}=w\}|}{|B^{(i)}|} &\text{if }|w|=l\le |B^{(i)}|,\\ 0&\text{otherwise.} \end{cases} \end{align} $$
$$ \begin{align} \operatorname{\mathrm{freq}}(w,B^{(i)})=\begin{cases}\frac{|\{1\le j \le |B^{(i)}|-l+1 \ : \ B^{(i)}_{[j,j+l)}=w\}|}{|B^{(i)}|} &\text{if }|w|=l\le |B^{(i)}|,\\ 0&\text{otherwise.} \end{cases} \end{align} $$
-
• The set of coordinates of x which belong to the block
 $A^{(i)}$
in (7) for some
$A^{(i)}$
in (7) for some
 $i\ge 1$
has upper Banach density smaller than
$i\ge 1$
has upper Banach density smaller than
 $\varepsilon $
. In particular, we have (10)
$\varepsilon $
. In particular, we have (10) $$ \begin{align} \limsup_{n\to\infty}\frac{|A^{(1)}|+\cdots|A^{(n)}|}{|A^{(1)}B^{(1)}|+\cdots+|A^{(n)}B^{(n)}|}<\varepsilon. \end{align} $$
$$ \begin{align} \limsup_{n\to\infty}\frac{|A^{(1)}|+\cdots|A^{(n)}|}{|A^{(1)}B^{(1)}|+\cdots+|A^{(n)}B^{(n)}|}<\varepsilon. \end{align} $$
We now use the assumption
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X),{\mathcal {M}_{\mathit \sigma }}(Y))<\varepsilon ^2$
and for every
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X),{\mathcal {M}_{\mathit \sigma }}(Y))<\varepsilon ^2$
and for every
![]() $i\geq 1$
we find an ergodic measure
$i\geq 1$
we find an ergodic measure
![]() $\nu ^{(i)}\in {\mathcal {M}_{\mathit \sigma }}(Y)$
such that
$\nu ^{(i)}\in {\mathcal {M}_{\mathit \sigma }}(Y)$
such that
Following Shields [Reference Shields41], for every
![]() $\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
and
$\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
and
![]() $n\ge 1$
we define
$n\ge 1$
we define
![]() $J_n=J_n(\mu ,\nu )$
to be the set of measures
$J_n=J_n(\mu ,\nu )$
to be the set of measures
![]() $\unicode{x3bb} _n$
on
$\unicode{x3bb} _n$
on
![]() $\mathscr {A}^n\times \mathscr {A}^n$
endowed with the powerset
$\mathscr {A}^n\times \mathscr {A}^n$
endowed with the powerset
![]() $\sigma $
-algebra such that for every
$\sigma $
-algebra such that for every
![]() $u,w\in \mathscr {A}^n$
we have
$u,w\in \mathscr {A}^n$
we have
![]() $\mu [u]=\unicode{x3bb} _n(\{u\}\times \mathscr {A}^n)$
and
$\mu [u]=\unicode{x3bb} _n(\{u\}\times \mathscr {A}^n)$
and
![]() $\nu [w]=\unicode{x3bb} _n(\mathscr {A}^n\times \{w\})$
. For
$\nu [w]=\unicode{x3bb} _n(\mathscr {A}^n\times \{w\})$
. For
![]() $\alpha>0$
we let
$\alpha>0$
we let
![]() $\Delta _n(\alpha )=\{(u,w)\in \mathscr {A}^{\hspace{2pt}n}\times \mathscr {A}^{\hspace{2pt}n}:d_{\textrm {Ham}}(u,w)\le \alpha \}$
. Finally, for
$\Delta _n(\alpha )=\{(u,w)\in \mathscr {A}^{\hspace{2pt}n}\times \mathscr {A}^{\hspace{2pt}n}:d_{\textrm {Ham}}(u,w)\le \alpha \}$
. Finally, for
![]() $\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
we write
$\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}({\mathscr {A}^{\hspace{2pt}\infty} })$
we write
By [Reference Shields41, §I.9], in particular [Reference Shields41, Lemma I.9.12], we see that
![]() $\bar {d}_{\mathcal {M}}(\mu ^{(i)},\nu ^{(i)})<\varepsilon ^2$
implies that for every
$\bar {d}_{\mathcal {M}}(\mu ^{(i)},\nu ^{(i)})<\varepsilon ^2$
implies that for every
![]() $n\ge 1$
we have
$n\ge 1$
we have
![]() $d^*_n(\mu ^{(i)},\nu ^{(i)})<\varepsilon $
. Hence, for every
$d^*_n(\mu ^{(i)},\nu ^{(i)})<\varepsilon $
. Hence, for every
![]() $n\ge 1$
and
$n\ge 1$
and
![]() $i\ge 1$
there exists
$i\ge 1$
there exists
![]() $\unicode{x3bb} ^{(i)}_n\in J_n(\mu ^{(i)},\nu ^{(i)}))$
such that
$\unicode{x3bb} ^{(i)}_n\in J_n(\mu ^{(i)},\nu ^{(i)}))$
such that
![]() $\unicode{x3bb} ^{(i)}_n(\Delta _n(\varepsilon ))>1-\varepsilon $
. Consider the set
$\unicode{x3bb} ^{(i)}_n(\Delta _n(\varepsilon ))>1-\varepsilon $
. Consider the set
It follows that for every
![]() $u\in G_n^{(i)}$
we can pick
$u\in G_n^{(i)}$
we can pick
![]() $w_n^{(i)}(u)$
such that
$w_n^{(i)}(u)$
such that
![]() $d_{\textrm {Ham}}(u,w^{(i)}_n(u))<\varepsilon $
and
$d_{\textrm {Ham}}(u,w^{(i)}_n(u))<\varepsilon $
and
![]() $\unicode{x3bb} _n^{(i)}(\{(u,w^{(i)}_n(u))\})>0$
. In particular,
$\unicode{x3bb} _n^{(i)}(\{(u,w^{(i)}_n(u))\})>0$
. In particular,
![]() $\nu ^{(i)}[w^{(i)}_n(u)]>0$
and hence
$\nu ^{(i)}[w^{(i)}_n(u)]>0$
and hence
![]() $w^{(i)}_n(u)\in \mathcal {B}_n(Y)$
. In addition, we clearly have
$w^{(i)}_n(u)\in \mathcal {B}_n(Y)$
. In addition, we clearly have
By an abuse of notation, for
![]() $i\ge 1$
and
$i\ge 1$
and
![]() $n\ge 1$
, by
$n\ge 1$
, by
![]() $\bigcup G^{(i)}_n$
we will understand
$\bigcup G^{(i)}_n$
we will understand
![]() $\bigcup \{[u]: u\in G_n^{(i)}\}$
.
$\bigcup \{[u]: u\in G_n^{(i)}\}$
.
It follows that for every
![]() $n\ge 1$
and
$n\ge 1$
and
![]() $i\ge 1$
we have
$i\ge 1$
we have
 $$ \begin{align} \mu^{(i)}\bigg(\bigcup G_n^{(i)} \bigg)=\unicode{x3bb}^{(i)}_n(G_n^{(i)}\times\mathscr{A}^{\hspace{2pt}n})\ge \unicode{x3bb}^{(i)}_n(\Delta_n(\varepsilon)\cap G_n^{(i)}\times\mathscr{A}^{\hspace{2pt}n})>1-\varepsilon. \end{align} $$
$$ \begin{align} \mu^{(i)}\bigg(\bigcup G_n^{(i)} \bigg)=\unicode{x3bb}^{(i)}_n(G_n^{(i)}\times\mathscr{A}^{\hspace{2pt}n})\ge \unicode{x3bb}^{(i)}_n(\Delta_n(\varepsilon)\cap G_n^{(i)}\times\mathscr{A}^{\hspace{2pt}n})>1-\varepsilon. \end{align} $$
For each
![]() $i\geq 1$
we take
$i\geq 1$
we take
![]() $n=s$
and consider
$n=s$
and consider
![]() $G_s^{(i)}\subseteq \mathcal {B}_s(X)$
. Note that (12) implies that
$G_s^{(i)}\subseteq \mathcal {B}_s(X)$
. Note that (12) implies that
![]() $\mu ^{(i)}(\bigcup G_s^{(i)})>1-\varepsilon $
. In analogy with (9), we define
$\mu ^{(i)}(\bigcup G_s^{(i)})>1-\varepsilon $
. In analogy with (9), we define
![]() $\operatorname {\mathrm {freq}}(G_s^{(i)},B^{(i)})$
to be number of coordinates in
$\operatorname {\mathrm {freq}}(G_s^{(i)},B^{(i)})$
to be number of coordinates in
![]() $B^{(i)}$
where some word from
$B^{(i)}$
where some word from
![]() $G^{(i)}_s$
appears in
$G^{(i)}_s$
appears in
![]() $B^{(i)}$
divided by the length of
$B^{(i)}$
divided by the length of
![]() $B^{(i)}$
, that is,
$B^{(i)}$
, that is,
 $$ \begin{align*} \operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})=\frac{|\{1\le j\le |B^{(i)}|-s+1: B^{(i)}_{[j,j+s)}\in G_s^{(i)}\}|}{|B^{(i)}|}. \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})=\frac{|\{1\le j\le |B^{(i)}|-s+1: B^{(i)}_{[j,j+s)}\in G_s^{(i)}\}|}{|B^{(i)}|}. \end{align*} $$
We easily see that
 $$ \begin{align*} \operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})=\sum_{w\in G_s^{(i)}}\operatorname{\mathrm{freq}}(w,B^{(i)}). \end{align*} $$
$$ \begin{align*} \operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})=\sum_{w\in G_s^{(i)}}\operatorname{\mathrm{freq}}(w,B^{(i)}). \end{align*} $$
Let P be the set of coordinates in
![]() $B^{(i)}$
covered by occurrences of words from
$B^{(i)}$
covered by occurrences of words from
![]() $G^{(i)}_s$
in
$G^{(i)}_s$
in
![]() $B^{(i)}$
, that is,
$B^{(i)}$
, that is,
 $$ \begin{align*} P=\{1\le p\le |B^{(i)}|: \text{ there exists } 1\le j\le |B^{(i)}|&-s+1 \text{ with } B^{(i)}_{[j,j+s)}\in G_s^{(i)}\text{ and } \\ &\quad j\le p<j+s\}. \end{align*} $$
$$ \begin{align*} P=\{1\le p\le |B^{(i)}|: \text{ there exists } 1\le j\le |B^{(i)}|&-s+1 \text{ with } B^{(i)}_{[j,j+s)}\in G_s^{(i)}\text{ and } \\ &\quad j\le p<j+s\}. \end{align*} $$
We clearly have
Furthermore,
 $$ \begin{align} \bigg|\mu^{(i)}\bigg(\bigcup G_s^{(i)}\bigg)-\operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})\bigg|\le \sum_{w\in G^{(i)}_s} |\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])|. \end{align} $$
$$ \begin{align} \bigg|\mu^{(i)}\bigg(\bigcup G_s^{(i)}\bigg)-\operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})\bigg|\le \sum_{w\in G^{(i)}_s} |\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])|. \end{align} $$
Using
![]() $d^*(B^{(i)},\mu ^{(i)})< {\varepsilon }/{2^{s}}$
(cf. (8)), we obtain
$d^*(B^{(i)},\mu ^{(i)})< {\varepsilon }/{2^{s}}$
(cf. (8)), we obtain
 $$ \begin{align} \sum_{w\in G^{(i)}_s}|\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])| & \le \sum_{w\in\mathscr{A}^s} |\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])| \end{align} $$
$$ \begin{align} \sum_{w\in G^{(i)}_s}|\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])| & \le \sum_{w\in\mathscr{A}^s} |\operatorname{\mathrm{freq}}(w,B^{(i)})-\mu^{(i)}([w])| \end{align} $$
Combining (14) and (15), we get
 $$ \begin{align} \bigg|\mu\bigg(\bigcup G_s^{(i)}\bigg)-\operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})\bigg|\le \varepsilon. \end{align} $$
$$ \begin{align} \bigg|\mu\bigg(\bigcup G_s^{(i)}\bigg)-\operatorname{\mathrm{freq}}(G_s^{(i)},B^{(i)})\bigg|\le \varepsilon. \end{align} $$
Combining (12) and (17), we see that
It follows that there exists a decomposition of
![]() $B^{(i)}$
such that
$B^{(i)}$
such that
where
![]() $\kappa (i)$
is some (large) positive integer,
$\kappa (i)$
is some (large) positive integer,
![]() $u^{(i,j)}\in G^{(i)}_s$
, and
$u^{(i,j)}\in G^{(i)}_s$
, and
![]() $v^{(i,j)}\in \mathcal {B}(X)\setminus G^{(i)}_s$
. Furthermore,
$v^{(i,j)}\in \mathcal {B}(X)\setminus G^{(i)}_s$
. Furthermore,
 $$ \begin{align} \frac{|v^{(i,1)}|+|v^{(i,2)}|+\cdots+|v^{(i,\kappa(i))}|+|v^{(i,\kappa(i)+1)}|}{|B^{(i)}|}\le\frac{|B^{(i)}|-|P|}{|B^{(i)}|}\le 2\varepsilon. \end{align} $$
$$ \begin{align} \frac{|v^{(i,1)}|+|v^{(i,2)}|+\cdots+|v^{(i,\kappa(i))}|+|v^{(i,\kappa(i)+1)}|}{|B^{(i)}|}\le\frac{|B^{(i)}|-|P|}{|B^{(i)}|}\le 2\varepsilon. \end{align} $$
(Actually, (19) tells us that
![]() $v^{(i,j)}$
is an empty word for many js.)
$v^{(i,j)}$
is an empty word for many js.)
We claim that if for each
![]() $i\geq 1$
we find blocks
$i\geq 1$
we find blocks
![]() $\hat {w}^{(i,j)}\in \mathcal {B}(Y)$
(
$\hat {w}^{(i,j)}\in \mathcal {B}(Y)$
(
![]() $j=1,\ldots ,\kappa (i)$
) with
$j=1,\ldots ,\kappa (i)$
) with
![]() $|\hat {w}^{(i,j)}|\ge s$
for each j and these blocks satisfy
$|\hat {w}^{(i,j)}|\ge s$
for each j and these blocks satisfy
and
 $$ \begin{align} d_{\textrm{Ham}}(A^{(i)}B^{(i)},\hat{w}^{(i,1)}\cdots \hat{w}^{(i,\kappa(i))})\le \frac{|A^{(i)}|+2\varepsilon|B^{(i)}|+|v^{(i,1)}|+\cdots+|v^{(i,\kappa(i)+1)}|+s}{|A^{(i)}B^{(i)}|}, \end{align} $$
$$ \begin{align} d_{\textrm{Ham}}(A^{(i)}B^{(i)},\hat{w}^{(i,1)}\cdots \hat{w}^{(i,\kappa(i))})\le \frac{|A^{(i)}|+2\varepsilon|B^{(i)}|+|v^{(i,1)}|+\cdots+|v^{(i,\kappa(i)+1)}|+s}{|A^{(i)}B^{(i)}|}, \end{align} $$
then the proof will be complete. Indeed, assume that for each
![]() $i\ge 1$
we have found blocks
$i\ge 1$
we have found blocks
![]() $\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
satisfying (20) and (21), each of them of length at least s. We set
$\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
satisfying (20) and (21), each of them of length at least s. We set
![]() $\hat {y}$
to be infinite concatenation of
$\hat {y}$
to be infinite concatenation of
![]() $\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
, where
$\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
, where
![]() $i=1,2,\ldots ,$
that is,
$i=1,2,\ldots ,$
that is,
By (7), (10), (19), (20), and (21) such
![]() $\hat {y}$
satisfies
$\hat {y}$
satisfies
Note that for every
![]() $i\ge 1$
and for every
$i\ge 1$
and for every
![]() $1\le j\le \kappa (i)$
we have
$1\le j\le \kappa (i)$
we have
![]() $\hat {w}^{(i,j)}\in \mathcal {B}(Y)$
and
$\hat {w}^{(i,j)}\in \mathcal {B}(Y)$
and
![]() $|\hat {w}^{(i,j)}|\ge s$
, so the
$|\hat {w}^{(i,j)}|\ge s$
, so the
![]() $\bar {d}$
-shadowing property guarantees there is
$\bar {d}$
-shadowing property guarantees there is
![]() $y\in Y$
with
$y\in Y$
with
By (22) and (23) we have
![]() $\bar {d}(x,y)<7\varepsilon $
as needed.
$\bar {d}(x,y)<7\varepsilon $
as needed.
It remains to find appropriate
![]() $\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
for each
$\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
for each
![]() $i\ge 1$
. To this end we fix
$i\ge 1$
. To this end we fix
![]() $i\ge 1$
and for each
$i\ge 1$
and for each
![]() $2\le j\le \kappa (i)$
we take
$2\le j\le \kappa (i)$
we take
![]() $u^{(i,j)}$
in equation (18) to find
$u^{(i,j)}$
in equation (18) to find
![]() $w^{(i,j)} =w^{(i,j)}(u^{(i,j)})\in \mathcal {B}_s(Y)$
with
$w^{(i,j)} =w^{(i,j)}(u^{(i,j)})\in \mathcal {B}_s(Y)$
with
![]() $d_{\textrm {Ham}}(u^{(i,j)},w^{(i,j)})\leq \varepsilon $
. Now, for
$d_{\textrm {Ham}}(u^{(i,j)},w^{(i,j)})\leq \varepsilon $
. Now, for
![]() $j=1$
we set
$j=1$
we set
![]() $t(i)=|A^{(i)}|+|v^{(i,1)}|+|w^{(i,1)}|+|v^{(i,2)}|\ge s$
and we pick any
$t(i)=|A^{(i)}|+|v^{(i,1)}|+|w^{(i,1)}|+|v^{(i,2)}|\ge s$
and we pick any
![]() $\hat {w}^{(i,1)}\in \mathcal {B}_{t(i)}(Y)$
. For
$\hat {w}^{(i,1)}\in \mathcal {B}_{t(i)}(Y)$
. For
![]() $2\le j\le \kappa (i)$
we simply extend each
$2\le j\le \kappa (i)$
we simply extend each
![]() $w^{(i,j)}$
to a word
$w^{(i,j)}$
to a word
![]() $\hat {w}^{(i,j)}=w^{(i,j)}\hat {v}^{(i,j+1)}\in \mathcal {B}_{|w^{(i,j)}|+|v^{(i,j+1)}|}(Y)$
, where
$\hat {w}^{(i,j)}=w^{(i,j)}\hat {v}^{(i,j+1)}\in \mathcal {B}_{|w^{(i,j)}|+|v^{(i,j+1)}|}(Y)$
, where
![]() $|\hat {v}^{(i,j+1)}|=|v^{(i,j+1)}|$
. We clearly have
$|\hat {v}^{(i,j+1)}|=|v^{(i,j+1)}|$
. We clearly have
![]() $|\hat {w}^{(i,j)}|\ge |w^{(i,j)}|=s$
and
$|\hat {w}^{(i,j)}|\ge |w^{(i,j)}|=s$
and
 $$ \begin{align*} d_{\textrm{Ham}}(\hat{w}^{(i,j)},u^{(i,j)}v^{(i,j+1)})\le \frac{|v^{(i,j+1)}|+\varepsilon|w^{(i,j)}|}{|\hat{w}^{(i,j)}|}. \end{align*} $$
$$ \begin{align*} d_{\textrm{Ham}}(\hat{w}^{(i,j)},u^{(i,j)}v^{(i,j+1)})\le \frac{|v^{(i,j+1)}|+\varepsilon|w^{(i,j)}|}{|\hat{w}^{(i,j)}|}. \end{align*} $$
It is now straightforward to see that the blocks
![]() $\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
satisfy (20) and (21). To finish the proof, reverse the roles of X and Y.
$\hat {w}^{(i,1)},\ldots ,\hat {w}^{(i,\kappa (i))}$
satisfy (20) and (21). To finish the proof, reverse the roles of X and Y.
4.1. Examples
In this subsection, we explore what happens if we abandon the assumption of
![]() $\bar {d}$
-shadowing. First, we prove Proposition 4.2 showing that the
$\bar {d}$
-shadowing. First, we prove Proposition 4.2 showing that the
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
limit of a sequence of simplices of invariant measures need not be a simplex of all invariant measures of some subshift. In particular, the shift spaces
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
limit of a sequence of simplices of invariant measures need not be a simplex of all invariant measures of some subshift. In particular, the shift spaces
![]() $X_k$
consisting of a single periodic orbit that have been constructed in the course of the proof of Proposition 4.2 do not converge in
$X_k$
consisting of a single periodic orbit that have been constructed in the course of the proof of Proposition 4.2 do not converge in
![]() ${\bar d}^{\mathrm {H}}$
-distance to a shift space, as their convergence to a shift X would imply that the limit of the corresponding simplices would be
${\bar d}^{\mathrm {H}}$
-distance to a shift space, as their convergence to a shift X would imply that the limit of the corresponding simplices would be
![]() ${\mathcal {M}_{\mathit \sigma }}(X)$
; see [Reference Konieczny, Kupsa and Kwietniak23].
${\mathcal {M}_{\mathit \sigma }}(X)$
; see [Reference Konieczny, Kupsa and Kwietniak23].
Proposition 4.2. For every alphabet
![]() $\mathscr {A}$
there exists a sequence of transitive finite shifts
$\mathscr {A}$
there exists a sequence of transitive finite shifts
![]() $(X_k)_{k=1}^{\infty} $
such that for some ergodic fully supported measure
$(X_k)_{k=1}^{\infty} $
such that for some ergodic fully supported measure
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}e({\mathscr {A}^{\hspace{2pt}\infty} })$
we have
$\mu \in {\mathcal {M}_{\mathit \sigma }}e({\mathscr {A}^{\hspace{2pt}\infty} })$
we have
In particular, there does not exist a shift space X such that
![]() $\{\mu \}={\mathcal {M}_{\mathit \sigma }}(X)$
.
$\{\mu \}={\mathcal {M}_{\mathit \sigma }}(X)$
.
Proof. We order the non-empty words over
![]() $\mathscr {A}$
into a sequence
$\mathscr {A}$
into a sequence
![]() $(W_k)_{k=0}^{\infty} $
. Let
$(W_k)_{k=0}^{\infty} $
. Let
![]() $(\delta _k)_{k=1}^{\infty} $
be a sequence of positive reals such that
$(\delta _k)_{k=1}^{\infty} $
be a sequence of positive reals such that
 $$ \begin{align} \sum_{k=1}^{\infty} \delta_k<\frac{1}{2}. \end{align} $$
$$ \begin{align} \sum_{k=1}^{\infty} \delta_k<\frac{1}{2}. \end{align} $$
We inductively define words
![]() $(V_k)_{k=0}^{\infty} $
by
$(V_k)_{k=0}^{\infty} $
by
 $$ \begin{align*} V_0&=W_0\\ V_{k+1}&=V_k^{a_{k+1}}W_{k+1}1^{b_{k+1}}\quad\text{for } k\ge 0, \end{align*} $$
$$ \begin{align*} V_0&=W_0\\ V_{k+1}&=V_k^{a_{k+1}}W_{k+1}1^{b_{k+1}}\quad\text{for } k\ge 0, \end{align*} $$
in such a way that
![]() $b_{k+1}\ge 0$
is the smallest number such that
$b_{k+1}\ge 0$
is the smallest number such that
![]() $|W_{k+1}|1^{b_{k+1}}$
is a multiple of
$|W_{k+1}|1^{b_{k+1}}$
is a multiple of
![]() $|V_k|$
and
$|V_k|$
and
![]() $a_{k+1}\ge 1$
is chosen so that the following inequality holds true:
$a_{k+1}\ge 1$
is chosen so that the following inequality holds true:
This implies that
![]() $c_k=|V_{k+1}|/|V_k|$
is a positive integer. For
$c_k=|V_{k+1}|/|V_k|$
is a positive integer. For
![]() $k\ge 1$
, let
$k\ge 1$
, let
![]() $x^{(k)}=V_k^{\infty }\in {\mathscr {A}^{\hspace{2pt}\infty} }$
be a periodic point,
$x^{(k)}=V_k^{\infty }\in {\mathscr {A}^{\hspace{2pt}\infty} }$
be a periodic point,
![]() $X_k$
be its orbit, and
$X_k$
be its orbit, and
![]() $\mu _k$
be the unique ergodic measure of the shift space
$\mu _k$
be the unique ergodic measure of the shift space
![]() $X_k$
.
$X_k$
.
Note that
![]() $\mu _k[W_k]\ge 1/|V_{k}|>0$
, and for every
$\mu _k[W_k]\ge 1/|V_{k}|>0$
, and for every
![]() $n>k$
we have
$n>k$
we have
because the infinite product
![]() $(1-\delta _{k+1})(1-\delta _{k+2})\cdots (1-\delta _n)\cdots $
converges to a non-zero limit by (24). We constructed the words
$(1-\delta _{k+1})(1-\delta _{k+2})\cdots (1-\delta _n)\cdots $
converges to a non-zero limit by (24). We constructed the words
![]() $V_k$
in such a way that
$V_k$
in such a way that
![]() $|V_{k+1}|$
is a multiple of
$|V_{k+1}|$
is a multiple of
![]() $|V_k|$
and that
$|V_k|$
and that
![]() $V_k^{a_{k+1}}$
is a prefix of
$V_k^{a_{k+1}}$
is a prefix of
![]() $V_{k+1}$
for every
$V_{k+1}$
for every
![]() $k\ge 0$
; hence, using (25), we see that for every
$k\ge 0$
; hence, using (25), we see that for every
![]() $k\ge q$
we have
$k\ge q$
we have
It follows that
![]() $x^{(k)}$
is a Cauchy sequence in the
$x^{(k)}$
is a Cauchy sequence in the
![]() $\bar {d}$
pseudometric. Since
$\bar {d}$
pseudometric. Since
![]() $\bar {d}$
is a complete pseudometric the sequence
$\bar {d}$
is a complete pseudometric the sequence
![]() $x^{(k)}$
converges, so there is
$x^{(k)}$
converges, so there is
![]() $x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that
$x\in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that
This point x must then be generic for an ergodic shift-invariant measure
![]() $\mu $
such that
$\mu $
such that
![]() $\mu $
is the
$\mu $
is the
![]() $\bar {d}_{\mathcal {M}}$
-limit of the measures
$\bar {d}_{\mathcal {M}}$
-limit of the measures
![]() $\mu _k$
. Since
$\mu _k$
. Since
![]() $\bar {d}_{\mathcal {M}}$
-convergence implies weak
$\bar {d}_{\mathcal {M}}$
-convergence implies weak
![]() $^*$
convergence, the portmanteau theorem and (26) imply that
$^*$
convergence, the portmanteau theorem and (26) imply that
 $$ \begin{align*} \mu[W_k]=\lim_{n\to\infty} \mu_n[W_k]\ge \frac{1}{|V_{k}|}\prod_{n=1}^{\infty}(1-\delta_{n})>0. \end{align*} $$
$$ \begin{align*} \mu[W_k]=\lim_{n\to\infty} \mu_n[W_k]\ge \frac{1}{|V_{k}|}\prod_{n=1}^{\infty}(1-\delta_{n})>0. \end{align*} $$
Since
![]() $\mu [W_k]>0$
for every
$\mu [W_k]>0$
for every
![]() $k\ge 0$
, the only subshift X such that
$k\ge 0$
, the only subshift X such that
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
is the full shift. On the other hand,
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
is the full shift. On the other hand,
![]() ${\mathcal {M}_{\mathit \sigma }}(X_k)=\{\mu _k\}$
and
${\mathcal {M}_{\mathit \sigma }}(X_k)=\{\mu _k\}$
and
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_k),\{\mu \})=\bar {d}(\mu _k,\mu )\to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_k),\{\mu \})=\bar {d}(\mu _k,\mu )\to 0$
as
![]() $k\to \infty $
. We see that
$k\to \infty $
. We see that
![]() ${\mathcal {M}_{\mathit \sigma }}(X_k)=\{\mu _k\}$
converges to
${\mathcal {M}_{\mathit \sigma }}(X_k)=\{\mu _k\}$
converges to
![]() $\{\mu \}$
in
$\{\mu \}$
in
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
but there is no subshift X of
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
but there is no subshift X of
![]() ${\mathscr {A}^{\hspace{2pt}\infty} }$
such that
${\mathscr {A}^{\hspace{2pt}\infty} }$
such that
![]() ${\mathcal {M}_{\mathit \sigma }}(X)=\{\mu \}$
.
${\mathcal {M}_{\mathit \sigma }}(X)=\{\mu \}$
.
Our next goal is to show an example of a sequence
![]() $(X_k)_{k=1}^{\infty} $
of shift spaces such that the
$(X_k)_{k=1}^{\infty} $
of shift spaces such that the
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
-limit of simplices
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
-limit of simplices
![]() ${\mathcal {M}_{\mathit \sigma }}(X_k)$
exists and is a simplex of invariant measures of some shift space X, but the shift spaces
${\mathcal {M}_{\mathit \sigma }}(X_k)$
exists and is a simplex of invariant measures of some shift space X, but the shift spaces
![]() $X_k$
do not converge to X with respect to the
$X_k$
do not converge to X with respect to the
![]() ${\bar d}^{\mathrm {H}}$
pseudometric.
${\bar d}^{\mathrm {H}}$
pseudometric.
To find our examples we will adapt the construction of one-sided Oxtoby sequences. The original Oxtoby sequence generates a minimal non-uniquely ergodic Toeplitz subshift; see [Reference Downarowicz7, Reference Oxtoby36, Reference Williams43]. As parameters of this construction we need a sequence of positive integers
![]() $(p_k)_{k=0}^{\infty} $
.
$(p_k)_{k=0}^{\infty} $
.
Definition 4.3. Let
![]() $\mathbf {p}=(p_k)_{k=0}^{\infty} $
be a sequence of positive integers such that
$\mathbf {p}=(p_k)_{k=0}^{\infty} $
be a sequence of positive integers such that
![]() $p_0=1$
, and for each
$p_0=1$
, and for each
![]() $k\ge 0$
we have that
$k\ge 0$
we have that
![]() $p_k$
divides
$p_k$
divides
![]() $p_{k+1}$
and
$p_{k+1}$
and
![]() $p_{k+1}/p_k\ge 3$
. Let
$p_{k+1}/p_k\ge 3$
. Let
![]() $M_0=\emptyset $
, and for
$M_0=\emptyset $
, and for
![]() $k\ge 1$
define
$k\ge 1$
define
![]() $M_k=([-p_k,p_k)+p_{k+1}\mathbb {N})\cap \mathbb {N}$
. Note that for every
$M_k=([-p_k,p_k)+p_{k+1}\mathbb {N})\cap \mathbb {N}$
. Note that for every
![]() $i\in \mathbb {N}$
there exists a unique
$i\in \mathbb {N}$
there exists a unique
![]() $k=k(i)\ge 1$
such that
$k=k(i)\ge 1$
such that
![]() $i\in M_{k}\setminus \bigcup ^{k-1}_{\ell =0} M_\ell $
. We define the Oxtoby sequence with the scale
$i\in M_{k}\setminus \bigcup ^{k-1}_{\ell =0} M_\ell $
. We define the Oxtoby sequence with the scale
![]() $\mathbf {p}$
to be a binary sequence
$\mathbf {p}$
to be a binary sequence
![]() $x(\mathbf {p})\in \{0,1\}^{\infty} $
such that
$x(\mathbf {p})\in \{0,1\}^{\infty} $
such that
![]() $x(\mathbf {p})_i=k(i) \bmod 2$
.
$x(\mathbf {p})_i=k(i) \bmod 2$
.
By Lemma 3.2 in [Reference Williams43], if
![]() $x(\mathbf {p})\in \{0,1\}^{\infty} $
is an Oxtoby sequence with scale
$x(\mathbf {p})\in \{0,1\}^{\infty} $
is an Oxtoby sequence with scale
![]() $\mathbf {p}$
satisfying
$\mathbf {p}$
satisfying
 $$ \begin{align*} \sum^{\infty}_{k=0}\frac{p_{k}}{p_{k+1}}<\infty, \end{align*} $$
$$ \begin{align*} \sum^{\infty}_{k=0}\frac{p_{k}}{p_{k+1}}<\infty, \end{align*} $$
then the orbit closure of
![]() $x(\mathbf {p})$
in
$x(\mathbf {p})$
in
![]() $\{0,1\}^{\infty} $
is a minimal shift space
$\{0,1\}^{\infty} $
is a minimal shift space
![]() $X(\mathbf {p})$
with exactly two ergodic invariant measures.
$X(\mathbf {p})$
with exactly two ergodic invariant measures.
Proposition 4.4. If
![]() $\boldsymbol{\mathrm{p}}=(p_k)_{k\in \mathbb {N}}$
is a sequence of positive integers satisfying
$\boldsymbol{\mathrm{p}}=(p_k)_{k\in \mathbb {N}}$
is a sequence of positive integers satisfying
 $$ \begin{align}\sum^{\infty}_{k=1}\frac{2p_{k}}{p_{k+1}}<\delta,\end{align} $$
$$ \begin{align}\sum^{\infty}_{k=1}\frac{2p_{k}}{p_{k+1}}<\delta,\end{align} $$
for some
![]() $0<\delta <\frac {1}{2}$
, then the minimal shift
$0<\delta <\frac {1}{2}$
, then the minimal shift
![]() $X(\boldsymbol{\mathrm{p}})$
obtained as the orbit closure of the Oxtoby sequence with scale
$X(\boldsymbol{\mathrm{p}})$
obtained as the orbit closure of the Oxtoby sequence with scale
![]() $\boldsymbol{\mathrm{p}}$
satisfies
$\boldsymbol{\mathrm{p}}$
satisfies
Proof. Fix
![]() $0<\delta <1$
and a sequence of positive integers
$0<\delta <1$
and a sequence of positive integers
![]() $\mathbf {p}$
as above. For simplicity we write x for the Oxtoby sequence
$\mathbf {p}$
as above. For simplicity we write x for the Oxtoby sequence
![]() $x(\mathbf {p})$
defined taking
$x(\mathbf {p})$
defined taking
![]() $\mathbf {p}$
as its scale and X for the associated minimal subshift
$\mathbf {p}$
as its scale and X for the associated minimal subshift
![]() $X(\mathbf {p})$
(see Definition 4.3). By Lemma 3.2 in [Reference Williams43],
$X(\mathbf {p})$
(see Definition 4.3). By Lemma 3.2 in [Reference Williams43],
![]() ${\mathcal {M}_{\mathit \sigma }}e(X)=\{\mu ',\nu '\}$
. Let
${\mathcal {M}_{\mathit \sigma }}e(X)=\{\mu ',\nu '\}$
. Let
![]() $(M_k)_{k=1}^{\infty} $
be a sequence of sets as in Definition 4.3. Fix
$(M_k)_{k=1}^{\infty} $
be a sequence of sets as in Definition 4.3. Fix
![]() $k\ge 1$
and consider the prefix
$k\ge 1$
and consider the prefix
![]() $x_{[0,p_{k+1})}$
. Since
$x_{[0,p_{k+1})}$
. Since
![]() $p_{k+1}$
is a multiple of
$p_{k+1}$
is a multiple of
![]() $p_\ell $
for every
$p_\ell $
for every
![]() $\ell \le k$
, using the structure of the sets
$\ell \le k$
, using the structure of the sets
![]() $M_k$
for
$M_k$
for
![]() $k\ge \ell $
we get that
$k\ge \ell $
we get that
Hence,
 $$ \begin{align*} \frac{|(\bigcup^{k}_{\ell=0} M_\ell)\cap [0,p_{k+1})|}{p_{k+1}}\le \sum^{k}_{\ell=0}\frac{2p_{\ell}}{p_{\ell+1}}\le \delta. \end{align*} $$
$$ \begin{align*} \frac{|(\bigcup^{k}_{\ell=0} M_\ell)\cap [0,p_{k+1})|}{p_{k+1}}\le \sum^{k}_{\ell=0}\frac{2p_{\ell}}{p_{\ell+1}}\le \delta. \end{align*} $$
But for every
![]() $i\in [0,p_{k+1})\setminus \bigcup ^{k}_{\ell =0} M_\ell $
we have
$i\in [0,p_{k+1})\setminus \bigcup ^{k}_{\ell =0} M_\ell $
we have
![]() $x_i={k+1} \bmod 2$
. In other words, the Oxtoby sequence is constant for all indices i in
$x_i={k+1} \bmod 2$
. In other words, the Oxtoby sequence is constant for all indices i in
![]() $[0,p_{k+1})\setminus \bigcup ^{k}_{\ell =0} M_\ell $
with the constant depending only on the parity of k. Since
$[0,p_{k+1})\setminus \bigcup ^{k}_{\ell =0} M_\ell $
with the constant depending only on the parity of k. Since
 $$ \begin{align*} \frac{|([0,p_{k+1})\cap\mathbb{N} \setminus \bigcup^{k}_{\ell=0} M_\ell)|}{p_{k+1}}\ge 1-\delta, \end{align*} $$
$$ \begin{align*} \frac{|([0,p_{k+1})\cap\mathbb{N} \setminus \bigcup^{k}_{\ell=0} M_\ell)|}{p_{k+1}}\ge 1-\delta, \end{align*} $$
we see that for both
![]() $\alpha =0$
and
$\alpha =0$
and
![]() $\alpha =1$
we have
$\alpha =1$
we have
Hence, for some invariant measure
![]() $\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}(X)$
we have
$\mu ,\nu \in {\mathcal {M}_{\mathit \sigma }}(X)$
we have
![]() $\mu ([0])>1-\delta $
and
$\mu ([0])>1-\delta $
and
![]() $\nu ([1])>1-\delta $
. By ergodic decomposition, these measures are convex combinations of the ergodic measures
$\nu ([1])>1-\delta $
. By ergodic decomposition, these measures are convex combinations of the ergodic measures
![]() $\mu '$
and
$\mu '$
and
![]() $\nu '$
. This implies that for one ergodic measure, say
$\nu '$
. This implies that for one ergodic measure, say
![]() $\mu '$
, we have
$\mu '$
, we have
![]() $\mu '([0])>1-\delta $
, while for the other one we have
$\mu '([0])>1-\delta $
, while for the other one we have
![]() $\nu '([1])>1-\delta $
.
$\nu '([1])>1-\delta $
.
A generic point for
![]() $\mu '$
has density of
$\mu '$
has density of
![]() $1$
at most
$1$
at most
![]() $\delta $
, so it is at most
$\delta $
, so it is at most
![]() $\delta $
far away from
$\delta $
far away from
![]() $0^{\infty} $
. Hence,
$0^{\infty} $
. Hence,
![]() $\bar {d}_{\mathcal {M}}(\mu ',\delta _{0^{\infty} })<\delta $
. Similarly,
$\bar {d}_{\mathcal {M}}(\mu ',\delta _{0^{\infty} })<\delta $
. Similarly,
![]() $\bar {d}_{\mathcal {M}}(\nu ',\delta _{1^{\infty} })<\delta $
. Therefore, the
$\bar {d}_{\mathcal {M}}(\nu ',\delta _{1^{\infty} })<\delta $
. Therefore, the
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
-distance between sets of ergodic measures on X and
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}$
-distance between sets of ergodic measures on X and
![]() $\{0^{\infty },1^{\infty }\}$
is bounded by
$\{0^{\infty },1^{\infty }\}$
is bounded by
![]() $\delta $
. By [Reference Konieczny, Kupsa and Kwietniak23, Lemma 14] (see (5) in the introduction) we have
$\delta $
. By [Reference Konieczny, Kupsa and Kwietniak23, Lemma 14] (see (5) in the introduction) we have
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X(\mathbf {p})), {\mathcal {M}_{\mathit \sigma }}(\{0^{\infty },1^{\infty }\}))=\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}e(X(\mathbf {p})),{\mathcal {M}_{\mathit \sigma }}e(\{0^{\infty },1^{\infty }\}))<\delta $
. On the other hand, by the above calculations, we see that the Oxtoby sequence x satisfies
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X(\mathbf {p})), {\mathcal {M}_{\mathit \sigma }}(\{0^{\infty },1^{\infty }\}))=\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}e(X(\mathbf {p})),{\mathcal {M}_{\mathit \sigma }}e(\{0^{\infty },1^{\infty }\}))<\delta $
. On the other hand, by the above calculations, we see that the Oxtoby sequence x satisfies
![]() $\bar {d}(x,1^{\infty} )>1-\delta $
and
$\bar {d}(x,1^{\infty} )>1-\delta $
and
![]() $\bar {d}(x,0^{\infty })>1-\delta $
, which means that
$\bar {d}(x,0^{\infty })>1-\delta $
, which means that
![]() ${\bar d}^{\mathrm {H}}(X(\mathbf {p}),\{0^{\infty },1^{\infty }\})>1-\delta $
.
${\bar d}^{\mathrm {H}}(X(\mathbf {p}),\{0^{\infty },1^{\infty }\})>1-\delta $
.
Corollary 4.5. There exists a sequence
![]() $(X_k)_{k=1}^{\infty} $
of minimal shift spaces such that for some shift space X we have
$(X_k)_{k=1}^{\infty} $
of minimal shift spaces such that for some shift space X we have
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
while
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}(X_n),{\mathcal {M}_{\mathit \sigma }}(X))\to 0$
while
![]() ${\bar d}^{\mathrm {H}}(X_n,X)\to 1$
as
${\bar d}^{\mathrm {H}}(X_n,X)\to 1$
as
![]() $n\to \infty $
.
$n\to \infty $
.
Proof. One can take
![]() $X=\{0^{\infty },1^{\infty }\}$
and the sequence of minimal shift spaces
$X=\{0^{\infty },1^{\infty }\}$
and the sequence of minimal shift spaces
![]() $X_n$
generated by Oxtoby sequences
$X_n$
generated by Oxtoby sequences
![]() $x^{(n)}$
constructed in the previous proposition for a sequence of
$x^{(n)}$
constructed in the previous proposition for a sequence of
![]() $\delta $
s going to zero.
$\delta $
s going to zero.
4.2. On
 $\bar {d}$
-shadowing on the measure center of X
$\bar {d}$
-shadowing on the measure center of X
Let us recall that the measure center
![]() $X^+$
of a shift space X is the smallest subshift of X containing supports of all invariant measures on X, that is,
$X^+$
of a shift space X is the smallest subshift of X containing supports of all invariant measures on X, that is,
![]() $X^+$
is the smallest closed set such that
$X^+$
is the smallest closed set such that
![]() $\mu (X^+)=1$
for every
$\mu (X^+)=1$
for every
![]() $\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
.
$\mu \in {\mathcal {M}_{\mathit \sigma }}(X)$
.
Fix a word u over
![]() $\mathscr {A}$
with
$\mathscr {A}$
with
![]() $|u|\geq k$
. Given a word w over
$|u|\geq k$
. Given a word w over
![]() $\mathscr {A}$
, we define
$\mathscr {A}$
, we define
![]() $\gamma _w(u)$
to be the number of occurrences of w in u, that is,
$\gamma _w(u)$
to be the number of occurrences of w in u, that is,
Furthermore, for
![]() $n\in \mathbb {N}$
with
$n\in \mathbb {N}$
with
![]() $n\ge |w|$
we set
$n\ge |w|$
we set
![]() $\Gamma _w(n)$
to be largest number of occurrences of w among all words u of length n, that is,
$\Gamma _w(n)$
to be largest number of occurrences of w among all words u of length n, that is,
It is a straightforward consequence of the definition that
![]() $\Gamma _w(uv)\le \Gamma _w(u)+\Gamma _w(v) +|w|-1$
for every
$\Gamma _w(uv)\le \Gamma _w(u)+\Gamma _w(v) +|w|-1$
for every
![]() $u,v,w\in \mathscr {A}^*$
. Thus,
$u,v,w\in \mathscr {A}^*$
. Thus,
for all
![]() $n,m$
.
$n,m$
.
We define the maximum limiting frequency of w in X as
The existence of the limit follows from the subadditivity of the function
![]() $\Gamma ^{\prime }_w(n)=\Gamma _w(n)+|w|-1$
and the fact that the difference between ratios
$\Gamma ^{\prime }_w(n)=\Gamma _w(n)+|w|-1$
and the fact that the difference between ratios
![]() $\Gamma _w(n)/n$
and
$\Gamma _w(n)/n$
and
![]() $\Gamma ^{\prime }_w(n)/n$
goes to zero.
$\Gamma ^{\prime }_w(n)/n$
goes to zero.
It is known that
see [Reference Furstenberg15, Ch. 3]. This means that
![]() $w\in \mathcal {B}(X)\setminus \mathcal {B}(X^+)$
if and only if
$w\in \mathcal {B}(X)\setminus \mathcal {B}(X^+)$
if and only if
![]() $\Lambda _X(w)=0$
, that is, for every
$\Lambda _X(w)=0$
, that is, for every
![]() $\varepsilon> 0$
there exists
$\varepsilon> 0$
there exists
![]() $N\in \mathbb {N}$
such that for all
$N\in \mathbb {N}$
such that for all
![]() $n\geq N$
and for all
$n\geq N$
and for all
![]() $u\in \mathcal {B}_n(X)$
we have
$u\in \mathcal {B}_n(X)$
we have
![]() $\gamma _w(u)\leq n\varepsilon $
.
$\gamma _w(u)\leq n\varepsilon $
.
Theorem 4.6. If
![]() $X^+$
has the
$X^+$
has the
![]() $\bar {d}$
-shadowing property then so does X.
$\bar {d}$
-shadowing property then so does X.
Proof. Fix
![]() $\varepsilon>0$
. For
$\varepsilon>0$
. For
![]() $\varepsilon /3$
we use the
$\varepsilon /3$
we use the
![]() $\bar {d}$
-shadowing property of
$\bar {d}$
-shadowing property of
![]() $X^+$
to find
$X^+$
to find
![]() $N_1$
such that for any sequence of words
$N_1$
such that for any sequence of words
![]() $\{ a^{(j)} \}_{j=1}^{\infty} $
in
$\{ a^{(j)} \}_{j=1}^{\infty} $
in
![]() $\mathcal {B}(X^+)$
with
$\mathcal {B}(X^+)$
with
![]() $|a^{(j)}|\geq N_1$
there exists
$|a^{(j)}|\geq N_1$
there exists
![]() $x\in X^+$
such that
$x\in X^+$
such that
![]() $\bar {d}(x,a^{(1)}a^{(2)}\cdots )<\varepsilon /3$
.
$\bar {d}(x,a^{(1)}a^{(2)}\cdots )<\varepsilon /3$
.
Now fix
![]() $m\geq N_1$
. For
$m\geq N_1$
. For
![]() $w\notin \mathcal {B}_m(X^+)$
let
$w\notin \mathcal {B}_m(X^+)$
let
![]() $N_w>0$
be such that for all
$N_w>0$
be such that for all
![]() $n\geq N_w$
and for all
$n\geq N_w$
and for all
![]() $u\in \mathcal {B}_n(X)$
we have
$u\in \mathcal {B}_n(X)$
we have
Set
![]() $N_0 = \max _{w\in \mathcal {B}_m(X)}{N_w}$
. We take
$N_0 = \max _{w\in \mathcal {B}_m(X)}{N_w}$
. We take
![]() $N\in \mathbb {N}$
such that
$N\in \mathbb {N}$
such that
![]() ${m}/{N}<\varepsilon /6$
and
${m}/{N}<\varepsilon /6$
and
![]() $N\geq \max \{N_0,N_1\}$
.
$N\geq \max \{N_0,N_1\}$
.
Let us take any
![]() $j\geq 1$
and any
$j\geq 1$
and any
![]() $w^{(j)}\in \mathcal {B}(X)$
such that
$w^{(j)}\in \mathcal {B}(X)$
such that
![]() $|w^{(j)}|\geq N$
. Each
$|w^{(j)}|\geq N$
. Each
![]() $w^{(j)}$
can be written as a concatenation of finite blocks as follows:
$w^{(j)}$
can be written as a concatenation of finite blocks as follows:
where
![]() $|u_i^{(j)}|=m$
for
$|u_i^{(j)}|=m$
for
![]() $1\leq i< k(j)$
and
$1\leq i< k(j)$
and
![]() $m\leq |u_{k(j)}^{(j)}|<2m$
. Using (31), we see that for
$m\leq |u_{k(j)}^{(j)}|<2m$
. Using (31), we see that for
![]() $1\leq i \leq k(j)-1$
the number of
$1\leq i \leq k(j)-1$
the number of
![]() $u_i^{(j)}$
which are not in
$u_i^{(j)}$
which are not in
![]() $\mathcal {B}_m(X^+)$
is bounded from above by
$\mathcal {B}_m(X^+)$
is bounded from above by
![]() ${\varepsilon |w^{(j)}|}/{3m}$
.
${\varepsilon |w^{(j)}|}/{3m}$
.
For each
![]() $j\geq 1$
, we create
$j\geq 1$
, we create
![]() $\bar {w}^{(j)}$
by replacing each
$\bar {w}^{(j)}$
by replacing each
![]() $u_i^{(j)}\notin \mathcal {B}(X^+)$
by some word
$u_i^{(j)}\notin \mathcal {B}(X^+)$
by some word
![]() $\bar {v}\in \mathcal {B}_m(X^+)$
for
$\bar {v}\in \mathcal {B}_m(X^+)$
for
![]() $1 \leq i < k(j)$
and replacing
$1 \leq i < k(j)$
and replacing
![]() $u_{k(j)}^{(j)}$
by some word
$u_{k(j)}^{(j)}$
by some word
![]() $\bar {v}^{(j)}\in \mathcal {B}(X^+)$
with
$\bar {v}^{(j)}\in \mathcal {B}(X^+)$
with
![]() $|u_{k(j)}|=|\bar {v}^{(j)}|$
if
$|u_{k(j)}|=|\bar {v}^{(j)}|$
if
![]() $u_{k(j)}^{(j)}\notin \mathcal {B}(X^+)$
. Therefore, we have
$u_{k(j)}^{(j)}\notin \mathcal {B}(X^+)$
. Therefore, we have
Notice that for each
![]() $j\geq 1$
the word
$j\geq 1$
the word
![]() $\bar {w}^{(j)}$
is a concatenation of words from
$\bar {w}^{(j)}$
is a concatenation of words from
![]() $\mathcal {B}(X^+)$
whose lengths are greater than or equal to m, so the same applies to
$\mathcal {B}(X^+)$
whose lengths are greater than or equal to m, so the same applies to
![]() $\bar {w}^{(1)}\bar {w}^{(2)}\cdots $
. Now we use the
$\bar {w}^{(1)}\bar {w}^{(2)}\cdots $
. Now we use the
![]() $\bar {d}$
-shadowing property of
$\bar {d}$
-shadowing property of
![]() $X^+$
and we find
$X^+$
and we find
![]() $x\in X^+ \subseteq X$
such that
$x\in X^+ \subseteq X$
such that
It follows from (32) and (33) that
![]() $\bar {d}(x,w^{(1)}w^{(2)}\cdots )<\varepsilon $
, which concludes the proof.
$\bar {d}(x,w^{(1)}w^{(2)}\cdots )<\varepsilon $
, which concludes the proof.
5.
 $\bar {d}$
-approachable examples of proximal and minimal shift spaces
$\bar {d}$
-approachable examples of proximal and minimal shift spaces
Before presenting the details of our constructions, we first recall the necessary background.
An (oriented)
![]() $\mathscr {A}$
-labeled (multi)graph is a triple
$\mathscr {A}$
-labeled (multi)graph is a triple
![]() $G = (V,E,\tau )$
, where V is the (finite) set of vertices,
$G = (V,E,\tau )$
, where V is the (finite) set of vertices,
![]() $E\subset V\times V$
is the edge set, and
$E\subset V\times V$
is the edge set, and
![]() $\tau \colon E \to \mathscr {A}$
is the label map. For each
$\tau \colon E \to \mathscr {A}$
is the label map. For each
![]() $e \in E$
we write
$e \in E$
we write
![]() $i(e), t(e) \in V$
, to denote, respectively, the initial vertex and the terminal vertex of e. We say that a sequence (finite or infinite) consisting of
$i(e), t(e) \in V$
, to denote, respectively, the initial vertex and the terminal vertex of e. We say that a sequence (finite or infinite) consisting of
![]() $\ell \in \mathbb {N}_0\cup \{\infty \}$
edges
$\ell \in \mathbb {N}_0\cup \{\infty \}$
edges
![]() $e_1, e_2, \ldots $
in E is a path of length
$e_1, e_2, \ldots $
in E is a path of length
![]() $\ell $
in G if for every
$\ell $
in G if for every
![]() $i < \ell $
we have that
$i < \ell $
we have that
![]() $t(e_i)=i(e_{i+1})$
. A path
$t(e_i)=i(e_{i+1})$
. A path
![]() $e_1, e_2, \ldots , e_\ell $
is closed if
$e_1, e_2, \ldots , e_\ell $
is closed if
![]() $t(e_\ell )=i(e_1)$
.
$t(e_\ell )=i(e_1)$
.
Given an oriented
![]() $\mathscr {A}$
-labeled graph
$\mathscr {A}$
-labeled graph
![]() $G = (V,E,\tau )$
, we define the shift
$G = (V,E,\tau )$
, we define the shift
![]() $X_G \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
by reading off labels of all infinite paths in G. In other words,
$X_G \subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
by reading off labels of all infinite paths in G. In other words,
![]() $X_G$
is the set of all
$X_G$
is the set of all
![]() $x \in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that
$x \in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that
![]() $x_i = \tau (e_{i+1})$
for each
$x_i = \tau (e_{i+1})$
for each
![]() $i \ge 0$
for some path
$i \ge 0$
for some path
![]() $e_1,e_2, \ldots $
in G. We say that X is a sofic shift if there exists a labeled graph
$e_1,e_2, \ldots $
in G. We say that X is a sofic shift if there exists a labeled graph
![]() $G= (V,E,\tau )$
such that X is presented by G, meaning that
$G= (V,E,\tau )$
such that X is presented by G, meaning that
![]() $X=X_G$
. Every shift of finite type is sofic. A sofic shift is transitive if and only if it can be presented by a (strongly) connected graph (each pair of vertices can be connected by a path); see [Reference Lind and Marcus32, Proposition 3.3.11]. A sofic shift is topologically mixing if and only if it can be presented by a (strongly) connected aperiodic graph, that is, the graph with two closed paths of coprime lengths.
$X=X_G$
. Every shift of finite type is sofic. A sofic shift is transitive if and only if it can be presented by a (strongly) connected graph (each pair of vertices can be connected by a path); see [Reference Lind and Marcus32, Proposition 3.3.11]. A sofic shift is topologically mixing if and only if it can be presented by a (strongly) connected aperiodic graph, that is, the graph with two closed paths of coprime lengths.
To prove the properties of shift spaces resulting from our constructions we will use the following result, which is a direct corollary of a combination of Theorem 6 and Corollary 17 in [Reference Konieczny, Kupsa and Kwietniak23].
Theorem 5.1. Let
![]() $(X_n)_{n=1}^{\infty} $
be a decreasing sequence of mixing sofic shift spaces over
$(X_n)_{n=1}^{\infty} $
be a decreasing sequence of mixing sofic shift spaces over
![]() $\mathscr {A}$
such that
$\mathscr {A}$
such that
 $$ \begin{align*} \sum_{n=1}^{\infty}{\bar d}^{\mathrm{H}}(X_n,X_{n+1})<\infty. \end{align*} $$
$$ \begin{align*} \sum_{n=1}^{\infty}{\bar d}^{\mathrm{H}}(X_n,X_{n+1})<\infty. \end{align*} $$
Then
![]() $X=\bigcap _{n=1}^{\infty} X_n$
is a
$X=\bigcap _{n=1}^{\infty} X_n$
is a
![]() $\bar {d}$
-approachable and chain-mixing shift space such that
$\bar {d}$
-approachable and chain-mixing shift space such that
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}e(X_n),{\mathcal {M}_{\mathit \sigma }}e(X)) \to 0$
as
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}e(X_n),{\mathcal {M}_{\mathit \sigma }}e(X)) \to 0$
as
![]() $n \to \infty $
. In particular, X satisfies
$n \to \infty $
. In particular, X satisfies
![]() $\sigma (X)=X$
and has the
$\sigma (X)=X$
and has the
![]() $\bar {d}$
-shadowing property.
$\bar {d}$
-shadowing property.
Let us recall that [Reference Konieczny, Kupsa and Kwietniak23, Corollary 17] is stated using the lower density. We do not need such flexibility here, so we stated our result in terms of the
![]() $\bar {d}$
-pseudodistance.
$\bar {d}$
-pseudodistance.
In fact, we would like to apply Theorem 5.1 to a sequence of mixing sofic shifts that is not decreasing. A natural way to apply Theorem 5.1 is to replace the sequence of sofic shifts
![]() $(X_n)_{n=1}^{\infty} $
with the decreasing sequence of shift spaces
$(X_n)_{n=1}^{\infty} $
with the decreasing sequence of shift spaces
![]() $(Y_n)_{n=1}^{\infty} $
, where
$(Y_n)_{n=1}^{\infty} $
, where
![]() $Y_n:=X_1\cap \cdots \cap X_n$
for
$Y_n:=X_1\cap \cdots \cap X_n$
for
![]() $n\in \mathbb {N}$
. It is easy to see that the shift
$n\in \mathbb {N}$
. It is easy to see that the shift
![]() $Y_n$
thus defined is also sofic for
$Y_n$
thus defined is also sofic for
![]() $n \in \mathbb {N}$
. Indeed, if for
$n \in \mathbb {N}$
. Indeed, if for
![]() $m=1,2,\ldots ,n$
a labeled graph
$m=1,2,\ldots ,n$
a labeled graph
![]() $G_m = (V_m,E_m,\tau _m)$
presents the sofic shift space
$G_m = (V_m,E_m,\tau _m)$
presents the sofic shift space
![]() $X_m$
, then
$X_m$
, then
![]() $Y_n$
is a sofic shift presented by the graph
$Y_n$
is a sofic shift presented by the graph
![]() $G=(V,E,\tau )$
, where
$G=(V,E,\tau )$
, where
![]() $V=\prod _{1\leq k\leq n}V_k$
and there is an edge from
$V=\prod _{1\leq k\leq n}V_k$
and there is an edge from
![]() $(v_1,\ldots ,v_n)\in V$
to
$(v_1,\ldots ,v_n)\in V$
to
![]() $(v^{\prime }_1,\ldots ,v^{\prime }_n)\in V$
with label
$(v^{\prime }_1,\ldots ,v^{\prime }_n)\in V$
with label
![]() $\ell \in \mathscr {A}$
if and only if for every
$\ell \in \mathscr {A}$
if and only if for every
![]() $1\leq k\leq n$
in the graph
$1\leq k\leq n$
in the graph
![]() $G_k$
there is an edge from
$G_k$
there is an edge from
![]() $v_k$
to
$v_k$
to
![]() $v^{\prime }_k$
labeled with
$v^{\prime }_k$
labeled with
![]() $\ell $
. We say that the graph
$\ell $
. We say that the graph
![]() $G=(V,E,\tau )$
is the coupling of graphs
$G=(V,E,\tau )$
is the coupling of graphs
![]() $G_k$
,
$G_k$
,
![]() $1\leq k\leq n$
. Unfortunately,
$1\leq k\leq n$
. Unfortunately,
![]() $Y_n$
need not be mixing even if the shift spaces
$Y_n$
need not be mixing even if the shift spaces
![]() $X_1,\ldots , X_n$
are. The problem is that strong connectedness of the graphs
$X_1,\ldots , X_n$
are. The problem is that strong connectedness of the graphs
![]() $G_k$
for
$G_k$
for
![]() $1\leq k\leq n$
need not ensure strong connectedness of their coupling G. Hence, the induced sofic shift
$1\leq k\leq n$
need not ensure strong connectedness of their coupling G. Hence, the induced sofic shift
![]() $Y_n$
need not be transitive and its ergodic measures need not be dense. Indeed, Figure 1 shows two graphs whose coupling is a sofic shift that is not transitive and whose ergodic measures are not dense in the set of all invariant measures. The same situation occurs for the sofic shifts represented in Figure 2.
$Y_n$
need not be transitive and its ergodic measures need not be dense. Indeed, Figure 1 shows two graphs whose coupling is a sofic shift that is not transitive and whose ergodic measures are not dense in the set of all invariant measures. The same situation occurs for the sofic shifts represented in Figure 2.

Figure 1 Two graphs (left) whose coupling (right) is disconnected. The first graph has no safe symbol.

Figure 2 Two graphs whose periods are not coprime (above) and their coupling (below).
However, under mild additional assumptions we can ensure that the sofic shifts
![]() $Y_n$
are transitive. Let X be a sofic shift over the alphabet
$Y_n$
are transitive. Let X be a sofic shift over the alphabet
![]() $\mathscr {A}$
and pick a labeled graph
$\mathscr {A}$
and pick a labeled graph
![]() $G = (V,E,\tau )$
presenting X. We call a symbol
$G = (V,E,\tau )$
presenting X. We call a symbol
![]() $b \in \mathscr {A}$
a safe symbol for X if for every edge
$b \in \mathscr {A}$
a safe symbol for X if for every edge
![]() $e\in E$
that goes from a vertex
$e\in E$
that goes from a vertex
![]() $v\in V$
to a vertex
$v\in V$
to a vertex
![]() $v'\in V$
, there is an edge
$v'\in V$
, there is an edge
![]() $e' \in E$
from v to
$e' \in E$
from v to
![]() $v'$
with label
$v'$
with label
![]() $\tau (e')=b$
. The period of a graph
$\tau (e')=b$
. The period of a graph
![]() $G = (V,E)$
is the greatest common divisor of the lengths of all cycles. Every sofic shift also has a period, which is the greatest common divisor of periods of its presentations through labeled graphs. A graph is aperiodic if it has period
$G = (V,E)$
is the greatest common divisor of the lengths of all cycles. Every sofic shift also has a period, which is the greatest common divisor of periods of its presentations through labeled graphs. A graph is aperiodic if it has period
![]() $1$
. Every sofic shift with period
$1$
. Every sofic shift with period
![]() $1$
has an aperiodic presentation. We will use a standard fact from graph theory, stating that if
$1$
has an aperiodic presentation. We will use a standard fact from graph theory, stating that if
![]() $G = (V,E)$
is a strongly connected graph with period m then the set of lengths of paths between any pair of vertices
$G = (V,E)$
is a strongly connected graph with period m then the set of lengths of paths between any pair of vertices
![]() $u,v \in V$
is the set-theoretic difference of an infinite arithmetic progression with step m and a finite set.
$u,v \in V$
is the set-theoretic difference of an infinite arithmetic progression with step m and a finite set.
Note that the two shifts in Figure 1 are aperiodic but lack a common safe symbol, while the two shifts in Figure 2 share a safe symbol
![]() $0$
but have positive periods
$0$
but have positive periods
![]() $8$
and
$8$
and
![]() $2$
. Hence, neither assumption in the following proposition can be removed.
$2$
. Hence, neither assumption in the following proposition can be removed.
Proposition 5.2. If
![]() $G_k=(V_k,E_k,\tau _k)$
for
$G_k=(V_k,E_k,\tau _k)$
for
![]() $1\leq k\leq n$
are strongly connected labeled graphs with a common safe symbol and pairwise coprime periods, then their coupling G is strongly connected.
$1\leq k\leq n$
are strongly connected labeled graphs with a common safe symbol and pairwise coprime periods, then their coupling G is strongly connected.
Proof. Let
![]() $(v_1,v_2,\ldots ,v_k)$
and
$(v_1,v_2,\ldots ,v_k)$
and
![]() $(v^{\prime }_1,v^{\prime }_2,\ldots ,v^{\prime }_n)$
be any two vertices in G. For any
$(v^{\prime }_1,v^{\prime }_2,\ldots ,v^{\prime }_n)$
be any two vertices in G. For any
![]() $1\leq k\leq n$
, there exists a walk from
$1\leq k\leq n$
, there exists a walk from
![]() $v_k$
to
$v_k$
to
![]() $v^{\prime }_k$
in the graph
$v^{\prime }_k$
in the graph
![]() $G_k$
. Let
$G_k$
. Let
![]() $\ell _k$
denote the length of one such walk and let
$\ell _k$
denote the length of one such walk and let
![]() $m_k$
denote the period of G. For all sufficiently large
$m_k$
denote the period of G. For all sufficiently large
![]() $j \in \mathbb {N}_0$
there exists a walk of length
$j \in \mathbb {N}_0$
there exists a walk of length
![]() $jm_k+\ell _k$
from
$jm_k+\ell _k$
from
![]() $v_k$
to
$v_k$
to
![]() $v^{\prime }_k$
. Since the graphs under consideration have a common safe symbol b, we may additionally assume that the edges in the aforementioned paths are all labeled with b. Since the integers
$v^{\prime }_k$
. Since the graphs under consideration have a common safe symbol b, we may additionally assume that the edges in the aforementioned paths are all labeled with b. Since the integers
![]() $m_k$
are coprime, there exist infinitely many integers
$m_k$
are coprime, there exist infinitely many integers
![]() $\ell $
such that
$\ell $
such that
![]() $\ell \equiv \ell _k \bmod {m_k}$
for each
$\ell \equiv \ell _k \bmod {m_k}$
for each
![]() $1\leq k \leq n$
. Consequently, we can find
$1\leq k \leq n$
. Consequently, we can find
![]() $\ell _0$
such that for each
$\ell _0$
such that for each
![]() $1\leq k \leq n$
there exists in
$1\leq k \leq n$
there exists in
![]() $G_k$
a walk from
$G_k$
a walk from
![]() $v_k$
to
$v_k$
to
![]() $v_k'$
of length
$v_k'$
of length
![]() $\ell _0$
, consisting only of edges labeled with b. This walk induces a walk of length
$\ell _0$
, consisting only of edges labeled with b. This walk induces a walk of length
![]() $\ell _0$
from
$\ell _0$
from
![]() $(v_1,v_2,\ldots ,v_k)$
to
$(v_1,v_2,\ldots ,v_k)$
to
![]() $(v^{\prime }_1,v^{\prime }_2,\ldots ,v^{\prime }_n)$
in G.
$(v^{\prime }_1,v^{\prime }_2,\ldots ,v^{\prime }_n)$
in G.
Corollary 5.3. Let
![]() $n\in \mathbb {N}$
. If
$n\in \mathbb {N}$
. If
![]() $X_{k}$
, for
$X_{k}$
, for
![]() $1\leq k\leq n$
, are transitive sofic shifts with a common safe symbol and pairwise coprime periods, then
$1\leq k\leq n$
, are transitive sofic shifts with a common safe symbol and pairwise coprime periods, then
![]() $Y=X_1\cap \cdots \cap X_n$
is a non-empty transitive sofic shift with a safe symbol. Furthermore, if for each
$Y=X_1\cap \cdots \cap X_n$
is a non-empty transitive sofic shift with a safe symbol. Furthermore, if for each
![]() $1\leq k\leq n$
the shift space
$1\leq k\leq n$
the shift space
![]() $X_k$
is topologically mixing, then Y is also topologically mixing.
$X_k$
is topologically mixing, then Y is also topologically mixing.
5.1. A
 $\bar {d}$
-approachable proximal shift space
$\bar {d}$
-approachable proximal shift space
We will construct a
![]() $\bar {d}$
-approachable and topologically mixing proximal shift. Furthermore, our example is hereditary and has positive topological entropy, hence its ergodic measures are entropy dense and its simplex of invariant measures is the Poulsen simplex. Assume that
$\bar {d}$
-approachable and topologically mixing proximal shift. Furthermore, our example is hereditary and has positive topological entropy, hence its ergodic measures are entropy dense and its simplex of invariant measures is the Poulsen simplex. Assume that
![]() $\mathscr {A}\hspace{-1pt}\subset\hspace{-1pt} \mathbb {N}_0$
. A shift space
$\mathscr {A}\hspace{-1pt}\subset\hspace{-1pt} \mathbb {N}_0$
. A shift space
![]() $X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is hereditary if for every
$X\subseteq {\mathscr {A}^{\hspace{2pt}\infty} }$
is hereditary if for every
![]() $x\in X$
and
$x\in X$
and
![]() $y\in {\mathscr {A}^{\hspace{2pt}\infty} }$
with
$y\in {\mathscr {A}^{\hspace{2pt}\infty} }$
with
![]() $y_i \leq x_i$
for all
$y_i \leq x_i$
for all
![]() $i \ge 0$
we have
$i \ge 0$
we have
![]() $y \in X$
. The hereditary closure
$y \in X$
. The hereditary closure
![]() $\tilde X$
of a shift space X is the smallest hereditary shift containing X. That is,
$\tilde X$
of a shift space X is the smallest hereditary shift containing X. That is,
![]() $\tilde {X}$
consists of all
$\tilde {X}$
consists of all
![]() $y \in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that there exists
$y \in {\mathscr {A}^{\hspace{2pt}\infty} }$
such that there exists
![]() $x=(x_i)_{i\ge 0} \in X$
with
$x=(x_i)_{i\ge 0} \in X$
with
![]() $y_i \leq x_i$
for all
$y_i \leq x_i$
for all
![]() $i \ge 0$
. For more on hereditary shifts, see [Reference Kwietniak29]. For some special properties of the simplex of invariant measures of hereditary shifts, see Remark 5.6 below. Recall that a hereditary shift space X is proximal if for every
$i \ge 0$
. For more on hereditary shifts, see [Reference Kwietniak29]. For some special properties of the simplex of invariant measures of hereditary shifts, see Remark 5.6 below. Recall that a hereditary shift space X is proximal if for every
![]() $N>0$
and
$N>0$
and
![]() $x\in X$
the word
$x\in X$
the word
![]() $0^N$
appears with bounded gaps in x (see the discussion of the Theorem B and the proof of Proposition 3.3 in [Reference Dymek, Kasjan, Kułaga-Przymus and Lemańczyk10]).
$0^N$
appears with bounded gaps in x (see the discussion of the Theorem B and the proof of Proposition 3.3 in [Reference Dymek, Kasjan, Kułaga-Przymus and Lemańczyk10]).
Example 5.4. For
![]() $n\in \mathbb {N}$
we consider a sofic shift
$n\in \mathbb {N}$
we consider a sofic shift
![]() $Z_n$
presented by a labeled graph
$Z_n$
presented by a labeled graph
![]() $G_n = (V_n,E_n,\tau _n)$
where
$G_n = (V_n,E_n,\tau _n)$
where
![]() $V_n=\{v_0, v_1, \ldots ,v_{10^n-1}\}$
and edges and their labels are as follows:
$V_n=\{v_0, v_1, \ldots ,v_{10^n-1}\}$
and edges and their labels are as follows:
-
• for every
 $0 \leq k < 10^n$
, there is an edge from
$0 \leq k < 10^n$
, there is an edge from
 $v_k$
to
$v_k$
to
 $v_{k+1}$
with label
$v_{k+1}$
with label
 $0$
, where
$0$
, where
 $v_{10^n} = v_{0}$
;
$v_{10^n} = v_{0}$
; -
• for every
 $1\leq k\leq 10^n-2^n$
, there is an edge from
$1\leq k\leq 10^n-2^n$
, there is an edge from
 $v_k$
to
$v_k$
to
 $v_{k+1}$
with label
$v_{k+1}$
with label
 $1$
;
$1$
; -
• there is an edge from
 $10^n-2^n$
to
$10^n-2^n$
to
 $10^n-2^n+2$
with label
$10^n-2^n+2$
with label
 $0$
.
$0$
.
Let
![]() $Z = \bigcap _{n=1}^{\infty} Z_n$
. Then for each
$Z = \bigcap _{n=1}^{\infty} Z_n$
. Then for each
![]() $n \geq 1$
we have
$n \geq 1$
we have
![]() $Z_{n+1} \not \subset Z_{n}$
and
$Z_{n+1} \not \subset Z_{n}$
and
![]() $Z_n \not \subset Z_{n+1}$
. Figure 3 shows the graph with
$Z_n \not \subset Z_{n+1}$
. Figure 3 shows the graph with
![]() $n=1$
.
$n=1$
.

Figure 3 The graph from Example 5.4 with
![]() $n=1$
.
$n=1$
.
Proposition 5.5. The shift space Z defined in Example 5.4 is hereditary, topologically mixing, proximal, has positive topological entropy, and the ergodic measures
![]() ${\mathcal {M}_{\mathit \sigma }}e(Z)$
are entropy dense in
${\mathcal {M}_{\mathit \sigma }}e(Z)$
are entropy dense in
![]() ${\mathcal {M}_{\mathit \sigma }}(Z)$
.
${\mathcal {M}_{\mathit \sigma }}(Z)$
.
Proof. Since all
![]() $Z_n$
are hereditary,
$Z_n$
are hereditary,
![]() $0$
is their common safe symbol and the shift Z is hereditary as well. Furthermore, it contains a sequence where
$0$
is their common safe symbol and the shift Z is hereditary as well. Furthermore, it contains a sequence where
![]() $1$
s appear with positive density, hence Z has positive entropy. For every
$1$
s appear with positive density, hence Z has positive entropy. For every
![]() $k\in \mathbb {N}$
, in the graph
$k\in \mathbb {N}$
, in the graph
![]() $G_k$
presenting
$G_k$
presenting
![]() $Z_k$
in Example 5.4 there are two closed walks of coprime lengths
$Z_k$
in Example 5.4 there are two closed walks of coprime lengths
![]() $10^k$
and
$10^k$
and
![]() $10^{k}-1$
, whence
$10^{k}-1$
, whence
![]() $G_k$
is aperiodic. By Corollary 5.3, for each
$G_k$
is aperiodic. By Corollary 5.3, for each
![]() $n \in \mathbb {N}$
the intersection
$n \in \mathbb {N}$
the intersection
![]() $Y_n:=Z_1\cap \cdots \cap Z_{n}$
is a topologically mixing sofic shift. In particular, the ergodic measures of
$Y_n:=Z_1\cap \cdots \cap Z_{n}$
is a topologically mixing sofic shift. In particular, the ergodic measures of
![]() $Y_n$
are entropy dense [Reference Eizenberg, Kifer and Weiss12]. By Corollary 5.1, in order to conclude that the ergodic measures on Z are entropy dense in
$Y_n$
are entropy dense [Reference Eizenberg, Kifer and Weiss12]. By Corollary 5.1, in order to conclude that the ergodic measures on Z are entropy dense in
![]() ${\mathcal {M}_{\mathit \sigma }}(Z)$
, it suffices to check that
${\mathcal {M}_{\mathit \sigma }}(Z)$
, it suffices to check that
 $$ \begin{align*}\sum^{\infty}_{n=1}{\bar d}^{\mathrm{H}}(Y_n,Y_{n+1})< \infty.\end{align*} $$
$$ \begin{align*}\sum^{\infty}_{n=1}{\bar d}^{\mathrm{H}}(Y_n,Y_{n+1})< \infty.\end{align*} $$
Fix
![]() $n\in \mathbb {N}$
. To bound
$n\in \mathbb {N}$
. To bound
![]() ${\bar d}^{\mathrm {H}}(Y_n,Y_{n+1})$
, consider
${\bar d}^{\mathrm {H}}(Y_n,Y_{n+1})$
, consider
![]() $x\in Y_n$
. Define
$x\in Y_n$
. Define
![]() $y\in \{0,1\}^{\infty} $
by
$y\in \{0,1\}^{\infty} $
by
 $$ \begin{align*} y_j= \begin{cases} x_j& \text{if } j \bmod{10^{n+1}} \in [0,10^{n+1}-2^{n+1}),\\ 0& \text{if } j \bmod{10^{n+1}} \in [10^{n+1}-2^{n+1},10^{n+1}). \end{cases} \end{align*} $$
$$ \begin{align*} y_j= \begin{cases} x_j& \text{if } j \bmod{10^{n+1}} \in [0,10^{n+1}-2^{n+1}),\\ 0& \text{if } j \bmod{10^{n+1}} \in [10^{n+1}-2^{n+1},10^{n+1}). \end{cases} \end{align*} $$
It is clear that
![]() $y\in Z_{n+1}$
. Since
$y\in Z_{n+1}$
. Since
![]() $Y_n$
is hereditary and
$Y_n$
is hereditary and
![]() $x\in Y_n$
, we see that y belongs to
$x\in Y_n$
, we see that y belongs to
![]() $Y_n$
as well. Thus,
$Y_n$
as well. Thus,
![]() $y\in Y_n\cap Z_{n+1}=Y_{n+1}$
. Furthermore,
$y\in Y_n\cap Z_{n+1}=Y_{n+1}$
. Furthermore,
![]() $\bar {d}(x,y)\leq (\frac {1}{5})^{n+1}$
, and hence
$\bar {d}(x,y)\leq (\frac {1}{5})^{n+1}$
, and hence
![]() ${\bar d}^{\mathrm {H}}(Y_n,Y_{n+1})\leq (\frac {1}{5})^{n+1}$
. This completes the proof of entropy density of ergodic measure.
${\bar d}^{\mathrm {H}}(Y_n,Y_{n+1})\leq (\frac {1}{5})^{n+1}$
. This completes the proof of entropy density of ergodic measure.
Finally, we prove topological mixing for Z. Bearing in mind that Z and
![]() $Z_n$
(
$Z_n$
(
![]() $n \in \mathbb {N}$
) are hereditary, in order to show that Z is topologically mixing it is enough to show that for each
$n \in \mathbb {N}$
) are hereditary, in order to show that Z is topologically mixing it is enough to show that for each
![]() $u,v \in \mathcal {B}(Z_n)$
there exists
$u,v \in \mathcal {B}(Z_n)$
there exists
![]() $M \in \mathbb {N}$
such that for all
$M \in \mathbb {N}$
such that for all
![]() $m \geq M$
and all
$m \geq M$
and all
![]() $n \in \mathbb {N}$
we have
$n \in \mathbb {N}$
we have
![]() $u 0^m v \in \mathcal {B}(Z_n)$
(hence
$u 0^m v \in \mathcal {B}(Z_n)$
(hence
![]() $u 0^m v \in \mathcal {B}(Z)$
).
$u 0^m v \in \mathcal {B}(Z)$
).
Fix
![]() $u,\hspace{-0.5pt}v$
, and denote
$u,\hspace{-0.5pt}v$
, and denote
![]() $i\hspace{-0.5pt}=\hspace{-0.5pt}|u|$
and
$i\hspace{-0.5pt}=\hspace{-0.5pt}|u|$
and
![]() $j\hspace{-0.5pt}=\hspace{-0.5pt}|v|$
. Let
$j\hspace{-0.5pt}=\hspace{-0.5pt}|v|$
. Let
![]() $N\hspace{-0.5pt} \in\hspace{-0.5pt} \mathbb {N}$
be such that
$N\hspace{-0.5pt} \in\hspace{-0.5pt} \mathbb {N}$
be such that
![]() $10^n\hspace{-0.5pt}>\hspace{-0.5pt} i\hspace{-0.5pt}+\hspace{-0.5pt}j\hspace{-0.5pt}+\hspace{-0.5pt}2(2^n\hspace{-0.5pt}-\hspace{-0.5pt}2)$
, for all
$10^n\hspace{-0.5pt}>\hspace{-0.5pt} i\hspace{-0.5pt}+\hspace{-0.5pt}j\hspace{-0.5pt}+\hspace{-0.5pt}2(2^n\hspace{-0.5pt}-\hspace{-0.5pt}2)$
, for all
![]() $n\ge N$
. Fix
$n\ge N$
. Fix
![]() $n\ge N$
. By the pigeonhole principle, for each
$n\ge N$
. By the pigeonhole principle, for each
![]() $m \in \mathbb {N}$
there exists
$m \in \mathbb {N}$
there exists
![]() $t \in \mathbb {N}$
such that
$t \in \mathbb {N}$
such that
![]() $[t,t+i) \bmod 10^n \subset [0,10^n-2^n)$
and
$[t,t+i) \bmod 10^n \subset [0,10^n-2^n)$
and
![]() $[t+i+m,t+i+m+j)\bmod 10^n \subset [0,10^n-2^n)$
. By the definition of
$[t+i+m,t+i+m+j)\bmod 10^n \subset [0,10^n-2^n)$
. By the definition of
![]() $Z_n$
, starting a path in the corresponding graph from
$Z_n$
, starting a path in the corresponding graph from
![]() $v_t$
and ending in
$v_t$
and ending in
![]() $v_{t+i+m+j}$
, we can read
$v_{t+i+m+j}$
, we can read
![]() $u0^mv$
along the path, so the word belongs to
$u0^mv$
along the path, so the word belongs to
![]() $\mathcal {B}_{i+m+j}(Z_n)$
. We have just proved that for all
$\mathcal {B}_{i+m+j}(Z_n)$
. We have just proved that for all
![]() $n\ge N$
and all
$n\ge N$
and all
![]() $m\in \mathbb {N}$
,
$m\in \mathbb {N}$
,
![]() $u0^mv\in \mathcal {B}(Z_n)$
. It remains to discuss the case when
$u0^mv\in \mathcal {B}(Z_n)$
. It remains to discuss the case when
![]() $n\le N$
.
$n\le N$
.
Since for each
![]() $n \in \mathbb {N}$
the system
$n \in \mathbb {N}$
the system
![]() $Z_n$
is mixing, there exists
$Z_n$
is mixing, there exists
![]() $M_n \in \mathbb {N}$
such that
$M_n \in \mathbb {N}$
such that
![]() $u 0^m v \in \mathcal {B}(Z_n)$
for all
$u 0^m v \in \mathcal {B}(Z_n)$
for all
![]() $m \geq M_n$
. Set M as the maximum of
$m \geq M_n$
. Set M as the maximum of
![]() $M_n$
,
$M_n$
,
![]() $n\le N$
. Then for
$n\le N$
. Then for
![]() $m\ge M$
,
$m\ge M$
,
![]() $u0^mv\in \mathcal {B}(Z_n)$
for all
$u0^mv\in \mathcal {B}(Z_n)$
for all
![]() $n\le N$
. But we have already proved the same conclusion for
$n\le N$
. But we have already proved the same conclusion for
![]() $n\ge N$
too. This concludes the proof of topological mixing of Z.
$n\ge N$
too. This concludes the proof of topological mixing of Z.
Since Z is hereditary, to prove that it is proximal it is enough to show that for every
![]() $N>0$
and
$N>0$
and
![]() $x\in Z$
the word
$x\in Z$
the word
![]() $0^N$
appears with bounded gaps in z (see [Reference Dymek, Kasjan, Kułaga-Przymus and Lemańczyk10]). But every
$0^N$
appears with bounded gaps in z (see [Reference Dymek, Kasjan, Kułaga-Przymus and Lemańczyk10]). But every
![]() $z\in Z$
must belong to
$z\in Z$
must belong to
![]() $Y_n$
for every
$Y_n$
for every
![]() $n\ge 1$
, so arbitrarily long blocks of
$n\ge 1$
, so arbitrarily long blocks of
![]() $0$
s appear syndetically (i.e., with bounded gaps).
$0$
s appear syndetically (i.e., with bounded gaps).
Remark 5.6. Since Z is hereditary and proximal, some of the results from [Reference Konieczny, Kupsa and Kwietniak23, Reference Kwietniak29] apply: Z is distributionally chaotic of type 2 [Reference Kwietniak29, Theorem 23], but not of type 1 [Reference Kwietniak29, Theorem 23] (cf. also [Reference Oprocha33]). Moreover, for each
![]() $t> 0$
, the set of all ergodic invariant measures on Z with entropy not exceeding t is arcwise connected with respect to the
$t> 0$
, the set of all ergodic invariant measures on Z with entropy not exceeding t is arcwise connected with respect to the
![]() $\bar {d}$
-metric on the set of all invariant measures [Reference Konieczny, Kupsa and Kwietniak23, Theorem 6].
$\bar {d}$
-metric on the set of all invariant measures [Reference Konieczny, Kupsa and Kwietniak23, Theorem 6].
5.2. A
 $\bar {d}$
-approachable minimal shift space
$\bar {d}$
-approachable minimal shift space
We will construct a minimal shift space which is
![]() $\bar {d}$
-approximable by a descending sequence of mixing sofic shifts. The sofic shift
$\bar {d}$
-approximable by a descending sequence of mixing sofic shifts. The sofic shift
![]() $X_n$
in the sequence will be generated by a finite code
$X_n$
in the sequence will be generated by a finite code
![]() $\mathcal {B}_n\subset \{0,1\}^+$
. The parameters of the construction are an initial finite non-empty set of words
$\mathcal {B}_n\subset \{0,1\}^+$
. The parameters of the construction are an initial finite non-empty set of words
![]() $\mathcal {B}_1$
and a sequence of positive integers
$\mathcal {B}_1$
and a sequence of positive integers
![]() $(t(n))^{\infty} _{n=1}$
. We assume that
$(t(n))^{\infty} _{n=1}$
. We assume that
![]() $t(n)\geq 2$
for every n. We will impose some more conditions on the
$t(n)\geq 2$
for every n. We will impose some more conditions on the
![]() $t(n)$
and
$t(n)$
and
![]() $\mathcal {B}_1$
later.
$\mathcal {B}_1$
later.
Assume we have defined the family of words
![]() $\mathcal {B}_n$
for some
$\mathcal {B}_n$
for some
![]() $n\ge 1$
. Write
$n\ge 1$
. Write
![]() $k(n)$
for the cardinality of
$k(n)$
for the cardinality of
![]() $\mathcal {B}_n$
. Enumerate the elements of
$\mathcal {B}_n$
. Enumerate the elements of
![]() $\mathcal {B}_n$
as
$\mathcal {B}_n$
as
![]() $\beta ^{(n)}_1,\ldots ,\beta ^{(n)}_{k(n)}$
, and let
$\beta ^{(n)}_1,\ldots ,\beta ^{(n)}_{k(n)}$
, and let
![]() $\tau (n)=\beta ^{(n)}_1\cdots \beta ^{(n)}_{k(n)}$
denote their concatenation. Let
$\tau (n)=\beta ^{(n)}_1\cdots \beta ^{(n)}_{k(n)}$
denote their concatenation. Let
![]() $s(n)$
(respectively,
$s(n)$
(respectively,
![]() $\ell (n)$
) be the length of the shortest (respectively, the longest) word in
$\ell (n)$
) be the length of the shortest (respectively, the longest) word in
![]() $\mathcal {B}_n$
. Words belonging to
$\mathcal {B}_n$
. Words belonging to
![]() $\mathcal {B}_{n+1}$
are constructed as follows. First we concatenate
$\mathcal {B}_{n+1}$
are constructed as follows. First we concatenate
![]() $t(n)$
arbitrarily chosen words from
$t(n)$
arbitrarily chosen words from
![]() $\mathcal {B}_n$
. Then we add the suffix
$\mathcal {B}_n$
. Then we add the suffix
![]() $\tau (n)$
:
$\tau (n)$
:
By the construction,
![]() $\ell (n)<\tau (n)<s(n+1)$
for every
$\ell (n)<\tau (n)<s(n+1)$
for every
![]() $n \geq 1$
and so
$n \geq 1$
and so
![]() $s(n)\nearrow \infty $
as
$s(n)\nearrow \infty $
as
![]() ${n\to \infty }$
. Moreover, every word from
${n\to \infty }$
. Moreover, every word from
![]() $\mathcal {B}_n$
is a subword of every word from
$\mathcal {B}_n$
is a subword of every word from
![]() $\mathcal {B}_{n+1}$
. Recursively,
$\mathcal {B}_{n+1}$
. Recursively,
For
![]() $n\ge 1$
, let
$n\ge 1$
, let
![]() $X_n$
be the coded shift generated by the code
$X_n$
be the coded shift generated by the code
![]() $\mathcal {B}_n$
. That is,
$\mathcal {B}_n$
. That is,
![]() $X_n$
consists of all concatenations of words from
$X_n$
consists of all concatenations of words from
![]() $\mathcal {B}_n$
together with their shifts. Since
$\mathcal {B}_n$
together with their shifts. Since
![]() $\mathcal {B}_n$
is finite, the shift
$\mathcal {B}_n$
is finite, the shift
![]() $X_n$
is transitive and sofic. It follows from (34) that
$X_n$
is transitive and sofic. It follows from (34) that
![]() $X_{n+1}\subseteq X_n$
. Hence,
$X_{n+1}\subseteq X_n$
. Hence,
![]() $X=\bigcap _{n=1}^{\infty} X_n$
is a non-empty shift space.
$X=\bigcap _{n=1}^{\infty} X_n$
is a non-empty shift space.
Proposition 5.7. The shift X constructed above is minimal.
Proof. If
![]() $|\mathcal {B}_1|=1$
, then X is an orbit of a periodic point. Let us assume that
$|\mathcal {B}_1|=1$
, then X is an orbit of a periodic point. Let us assume that
![]() $|\mathcal {B}_1|>1$
, so
$|\mathcal {B}_1|>1$
, so
![]() $|\mathcal {B}_n|>1$
for every n. We need to prove that if
$|\mathcal {B}_n|>1$
for every n. We need to prove that if
![]() $u\in \mathcal {B}(X)=\bigcap _{n=1}^{\infty} \mathcal {B}(X_n)$
and
$u\in \mathcal {B}(X)=\bigcap _{n=1}^{\infty} \mathcal {B}(X_n)$
and
![]() $x\in X$
, then u appears in x. Fix
$x\in X$
, then u appears in x. Fix
![]() $x\in X$
and
$x\in X$
and
![]() $u\in \mathcal {B}(X)$
. Take n large enough to imply
$u\in \mathcal {B}(X)$
. Take n large enough to imply
![]() $|u|< s(n)$
. Since
$|u|< s(n)$
. Since
![]() $\mathcal {B}(X)\subseteq \mathcal {B}(X_n)$
, we see that u must appear in some
$\mathcal {B}(X)\subseteq \mathcal {B}(X_n)$
, we see that u must appear in some
![]() $x'\in X_n$
. As all words in
$x'\in X_n$
. As all words in
![]() $\mathcal {B}_n$
are longer than u and
$\mathcal {B}_n$
are longer than u and
![]() $x'$
is a shift (possibly trivial) of an infinite concatenation of words from
$x'$
is a shift (possibly trivial) of an infinite concatenation of words from
![]() $\mathcal {B}_n$
, we conclude that u is a subword of some
$\mathcal {B}_n$
, we conclude that u is a subword of some
![]() $\bar u\in \mathcal {B}(X_n)$
which is the concatenation of two words
$\bar u\in \mathcal {B}(X_n)$
which is the concatenation of two words
![]() $v,w$
in
$v,w$
in
![]() $\mathcal {B}_{n}$
(one of them might be empty). By the definition of the
$\mathcal {B}_{n}$
(one of them might be empty). By the definition of the
![]() $\mathcal {B}_n$
, every concatenation
$\mathcal {B}_n$
, every concatenation
![]() $vw$
for words v and w from
$vw$
for words v and w from
![]() $\mathcal {B}_n$
appears in some word from
$\mathcal {B}_n$
appears in some word from
![]() $\mathcal {B}_{n+1}$
, therefore
$\mathcal {B}_{n+1}$
, therefore
![]() $vw$
and, in particular, u is a subword of a word
$vw$
and, in particular, u is a subword of a word
![]() $w'\in \mathcal {B}_{n+1}$
. Hence, condition (34) ensures that u is a subword of all words from
$w'\in \mathcal {B}_{n+1}$
. Hence, condition (34) ensures that u is a subword of all words from
![]() $\mathcal {B}_{n+2}$
. But
$\mathcal {B}_{n+2}$
. But
![]() $x\in X_{n+2}$
, so it is a shifted infinite concatenation of words from
$x\in X_{n+2}$
, so it is a shifted infinite concatenation of words from
![]() $\mathcal {B}_{n+2}$
. In particular, some word from
$\mathcal {B}_{n+2}$
. In particular, some word from
![]() $\mathcal {B}_{n+2}$
appears in x, and so does u.
$\mathcal {B}_{n+2}$
appears in x, and so does u.
From now on, we set
![]() $\mathcal {B}_1=\{0,11\}$
. For this choice of
$\mathcal {B}_1=\{0,11\}$
. For this choice of
![]() $\mathcal {B}_1$
a simple inductive argument shows that for each
$\mathcal {B}_1$
a simple inductive argument shows that for each
![]() $n \geq 1$
, the set of lengths of all words in
$n \geq 1$
, the set of lengths of all words in
![]() $\mathcal {B}_n$
is an interval:
$\mathcal {B}_n$
is an interval:
Proposition 5.8. For every
![]() $n\ge 1$
the coded system
$n\ge 1$
the coded system
![]() $X_n$
is a mixing sofic shift.
$X_n$
is a mixing sofic shift.
Proof. Any coded system generated by a finite sequence of words is sofic. In the corresponding graphs, every word in
![]() $\mathcal {B}_n$
is represented by a cycle, and all these cycles have a common vertex. In particular, the graph presenting
$\mathcal {B}_n$
is represented by a cycle, and all these cycles have a common vertex. In particular, the graph presenting
![]() $X_n$
is strongly irreducible and
$X_n$
is strongly irreducible and
![]() $X_n$
is transitive. In addition, it follows from (35) that there are two words in
$X_n$
is transitive. In addition, it follows from (35) that there are two words in
![]() $\mathcal {B}_n$
with coprime lengths, so the graph is aperiodic, thus
$\mathcal {B}_n$
with coprime lengths, so the graph is aperiodic, thus
![]() $X_n$
is mixing and has the specification property.
$X_n$
is mixing and has the specification property.
In the rest of the section, it will be convenient to control the ratio
![]() ${s(n)}/{\ell (n)}$
. Because of the identities
${s(n)}/{\ell (n)}$
. Because of the identities
 $$ \begin{align*} s(n+1) &= t(n)s(n) + |{\tau(n)}|,\\ \ell(n+1) &= t(n)\ell(n) + |{\tau(n)}|, \end{align*} $$
$$ \begin{align*} s(n+1) &= t(n)s(n) + |{\tau(n)}|,\\ \ell(n+1) &= t(n)\ell(n) + |{\tau(n)}|, \end{align*} $$
we get that the ratio is increasing and
On the other hand, we can ensure that
by satisfying the equivalent condition (the equivalence follows from the inductive definition of
![]() $s(n)$
and
$s(n)$
and
![]() $\ell (n)$
mentioned above)
$\ell (n)$
mentioned above)
Since
![]() $|\tau (n)|$
,
$|\tau (n)|$
,
![]() $\ell (n)$
and
$\ell (n)$
and
![]() $s(n)$
are determined by
$s(n)$
are determined by
![]() $t(i)$
,
$t(i)$
,
![]() $1\le i<n$
, we have enough freedom to construct the sequence
$1\le i<n$
, we have enough freedom to construct the sequence
![]() $t(n)$
satisfying condition (37) in an inductive way.
$t(n)$
satisfying condition (37) in an inductive way.
Proposition 5.9. Let
![]() $\varepsilon>0$
and
$\varepsilon>0$
and
![]() $t(n)$
be such a sequence that satisfies condition (37) and
$t(n)$
be such a sequence that satisfies condition (37) and
Then
 $$ \begin{align} \sum^{\infty}_{n=1}{\bar d}^{\mathrm{H}}(X_n,X_{n+1})<\varepsilon. \end{align} $$
$$ \begin{align} \sum^{\infty}_{n=1}{\bar d}^{\mathrm{H}}(X_n,X_{n+1})<\varepsilon. \end{align} $$
Proof. Put
![]() $\varepsilon _n=\varepsilon 2^{-n}$
. We will show that
$\varepsilon _n=\varepsilon 2^{-n}$
. We will show that
![]() ${\bar d}^{\mathrm {H}}(X_n,X_{n+1}) < \varepsilon _n$
for all
${\bar d}^{\mathrm {H}}(X_n,X_{n+1}) < \varepsilon _n$
for all
![]() $n \geq 1$
, which directly implies (39). Fix
$n \geq 1$
, which directly implies (39). Fix
![]() $n\ge 1$
and
$n\ge 1$
and
![]() $y\in X_n$
. Our goal is to find
$y\in X_n$
. Our goal is to find
![]() $z\in X_{n+1}$
such that
$z\in X_{n+1}$
such that
![]() $\bar {d}(y,z)<\varepsilon _n$
. Since
$\bar {d}(y,z)<\varepsilon _n$
. Since
![]() $\bar {d}$
is shift invariant, without loss of generality we assume that y is a concatenation of blocks from
$\bar {d}$
is shift invariant, without loss of generality we assume that y is a concatenation of blocks from
![]() $\mathcal {B}_n$
, that is, we have
$\mathcal {B}_n$
, that is, we have
We will construct z inductively. First, we note that the word
belongs to
![]() $\mathcal {B}_{n+1}$
. Let
$\mathcal {B}_{n+1}$
. Let
![]() $j \geq t(n)$
be the index with
$j \geq t(n)$
be the index with
and let a be the suffix of
![]() $b_{j+1}$
such that
$b_{j+1}$
such that
![]() $|b_1b_2\cdots b_j b_{j+1}| = |w| + |a|$
. We observe that there exist words
$|b_1b_2\cdots b_j b_{j+1}| = |w| + |a|$
. We observe that there exist words
![]() $b_{1}', b_{2}', b_{3}' \in \mathcal {B}_{n} \cup \{\unicode{x3bb} \}$
such that
$b_{1}', b_{2}', b_{3}' \in \mathcal {B}_{n} \cup \{\unicode{x3bb} \}$
such that
![]() $|b_{1}'b_{2}' b_{3}'| = |a b_{j+2} b_{j+3}|$
. Indeed, if
$|b_{1}'b_{2}' b_{3}'| = |a b_{j+2} b_{j+3}|$
. Indeed, if
![]() $2s(n) \leq |a b_{j+2} b_{j+3}| \leq 2\ell (n)$
then it follows from (35) that we can find
$2s(n) \leq |a b_{j+2} b_{j+3}| \leq 2\ell (n)$
then it follows from (35) that we can find
![]() $b_{1}', b_{2}' \in \mathcal {B}_{n}$
and
$b_{1}', b_{2}' \in \mathcal {B}_{n}$
and
![]() $ b_{3}' =\unicode{x3bb} $
with the required total length. Likewise, if
$ b_{3}' =\unicode{x3bb} $
with the required total length. Likewise, if
![]() $3s(n) \leq |a b_{j+2} b_{j+3}| \leq 3\ell (n)$
then we can apply the same argument with
$3s(n) \leq |a b_{j+2} b_{j+3}| \leq 3\ell (n)$
then we can apply the same argument with
![]() $b_{1}', b_{2}', b_{3}' \in \mathcal {B}_{n}$
. Since
$b_{1}', b_{2}', b_{3}' \in \mathcal {B}_{n}$
. Since
![]() $|a| < \ell (n)$
and
$|a| < \ell (n)$
and
![]() $3s(n) < 2\ell (n)$
(we assumed (37), which is equivalent to (36)), these two cases cover all possibilities.
$3s(n) < 2\ell (n)$
(we assumed (37), which is equivalent to (36)), these two cases cover all possibilities.
For
![]() $i \geq 3$
, define
$i \geq 3$
, define
![]() $b^{\prime }_{i} = b_{j+i}$
and let
$b^{\prime }_{i} = b_{j+i}$
and let
![]() $y' = b^{\prime }_{1}b^{\prime }_{2} b^{\prime }_{3}\ldots .$
Then y and
$y' = b^{\prime }_{1}b^{\prime }_{2} b^{\prime }_{3}\ldots .$
Then y and
![]() $w y'$
differ only on the positions where
$w y'$
differ only on the positions where
![]() $\tau (n)b^{\prime }_{1}b^{\prime }_{2} b^{\prime }_{3}$
appears in
$\tau (n)b^{\prime }_{1}b^{\prime }_{2} b^{\prime }_{3}$
appears in
![]() $w y'$
, that is, at most on positions between
$w y'$
, that is, at most on positions between
![]() $|w|-|\tau (n)|$
and
$|w|-|\tau (n)|$
and
![]() $|w|+ 3\ell (n)$
. Let us point out that
$|w|+ 3\ell (n)$
. Let us point out that
![]() $y'$
is again a concatenation of blocks from
$y'$
is again a concatenation of blocks from
![]() $\mathcal {B}_n$
, even in the case when
$\mathcal {B}_n$
, even in the case when
![]() $b_3'$
is the empty word. Hence, we can apply the same reasoning to
$b_3'$
is the empty word. Hence, we can apply the same reasoning to
![]() $y'$
,
$y'$
,
![]() $y"$
,
$y"$
,
![]() $y"'$
and so on, to obtain the word
$y"'$
and so on, to obtain the word
![]() $z = w w' w" \cdots $
. Here, we adopt the convention that
$z = w w' w" \cdots $
. Here, we adopt the convention that
![]() $y",w'$
are constructed from
$y",w'$
are constructed from
![]() $y'$
in the same way as
$y'$
in the same way as
![]() $y',w$
were constructed from y, and accordingly for further steps in the construction.
$y',w$
were constructed from y, and accordingly for further steps in the construction.
Since
![]() $w,w',w" \in \mathcal {B}_{n+1}$
, we have
$w,w',w" \in \mathcal {B}_{n+1}$
, we have
![]() $z \in X_{n+1}$
. Note that for each
$z \in X_{n+1}$
. Note that for each
![]() $i \geq 0$
we have
$i \geq 0$
we have
![]() $s(n)t(n) + \tau (n) \leq |w^{(i)}| \leq \ell (n)t(n) + \tau (n)$
, and consequently
$s(n)t(n) + \tau (n) \leq |w^{(i)}| \leq \ell (n)t(n) + \tau (n)$
, and consequently
In the last inequality we use condition (38), which is stronger.
Hence, z has all of the required properties.
Proposition 5.10. Let the sequence
![]() $t(n)$
,
$t(n)$
,
![]() $n\ge 1$
, satisfy (36) and
$n\ge 1$
, satisfy (36) and
Then X is mixing.
Proof. We need to show that for all
![]() $u,v \in \mathcal {B}(X)$
there exists M such that for each
$u,v \in \mathcal {B}(X)$
there exists M such that for each
![]() $m \geq M$
there exists a word w with
$m \geq M$
there exists a word w with
![]() $|{w}| = m$
such that
$|{w}| = m$
such that
![]() $uwv \in \mathcal {B}(X)$
. Note that we can freely replace
$uwv \in \mathcal {B}(X)$
. Note that we can freely replace
![]() $u,v$
with any other words
$u,v$
with any other words
![]() $u',v' \in \mathcal {B}(X)$
which contain
$u',v' \in \mathcal {B}(X)$
which contain
![]() $u,v$
as subwords. Hence (repeating an argument from the proof of Proposition 5.7), we may assume that
$u,v$
as subwords. Hence (repeating an argument from the proof of Proposition 5.7), we may assume that
![]() $u,v \in \mathcal {B}_n$
for some
$u,v \in \mathcal {B}_n$
for some
![]() $n \geq 1$
. Proceeding by induction on m, we will prove the following statement.
$n \geq 1$
. Proceeding by induction on m, we will prove the following statement.
Claim. For all
![]() $m \geq 0$
, for all
$m \geq 0$
, for all
![]() $n \geq 1$
such that
$n \geq 1$
such that
![]() $2s(n) \leq m$
, for all
$2s(n) \leq m$
, for all
![]() $u,v \in \mathcal {B}(X)$
, there exists w with
$u,v \in \mathcal {B}(X)$
, there exists w with
![]() $|{w}| = m$
and
$|{w}| = m$
and
![]() $uwv \in \mathcal {B}(X)$
.
$uwv \in \mathcal {B}(X)$
.
We may assume that the claim above has been proved for all
![]() $m' < m$
. We consider three cases depending on the magnitude of m.
$m' < m$
. We consider three cases depending on the magnitude of m.
Case 1. Suppose first that
![]() $2s(n) \leq m \leq (t(n)-2)\ell (n)$
. Note that for each
$2s(n) \leq m \leq (t(n)-2)\ell (n)$
. Note that for each
![]() $j \geq 2$
it follows from (36) that
$j \geq 2$
it follows from (36) that
As a consequence, the intervals
![]() $[ js(n), j\ell (n) ]$
for
$[ js(n), j\ell (n) ]$
for
![]() $2 \leq j \leq t(n)-2$
fully cover the interval
$2 \leq j \leq t(n)-2$
fully cover the interval
![]() $[2s(n),(t(n)-2) \ell (n)]$
. Hence, we can find j with
$[2s(n),(t(n)-2) \ell (n)]$
. Hence, we can find j with
![]() $2 \leq j \leq t(n)-2$
such that
$2 \leq j \leq t(n)-2$
such that
![]() $js(n) \leq m \leq j\ell (n)$
. It follows from (35) that there exists a word w with
$js(n) \leq m \leq j\ell (n)$
. It follows from (35) that there exists a word w with
![]() $|{w}|= m$
of the form
$|{w}|= m$
of the form
![]() $w = b_1b_2\cdots b_j$
with
$w = b_1b_2\cdots b_j$
with
![]() $b_1,b_2,\ldots ,b_j \in \mathcal {B}_n$
. Thus,
$b_1,b_2,\ldots ,b_j \in \mathcal {B}_n$
. Thus,
![]() $uwv$
is a prefix of
$uwv$
is a prefix of
![]() $\mathcal {B}_{n+1}$
(specifically, of any word of the form
$\mathcal {B}_{n+1}$
(specifically, of any word of the form
![]() $ub_1b_2\cdots b_j v b_1' b_2\cdots b_i \tau (n)$
, where
$ub_1b_2\cdots b_j v b_1' b_2\cdots b_i \tau (n)$
, where
![]() $i = t(n)-j-2$
and
$i = t(n)-j-2$
and
![]() $b_1',b_2',\ldots ,b_i'\in \mathcal {B}_n$
). It follows that
$b_1',b_2',\ldots ,b_i'\in \mathcal {B}_n$
). It follows that
![]() $uwv \in \mathcal {B}(X)$
.
$uwv \in \mathcal {B}(X)$
.
Case 2. Suppose next that
![]() $(t(n)-2)\ell (n) < m \leq (2t(n)-2)\ell (n)+|{\tau (n)}|$
. Then by (41) we have
$(t(n)-2)\ell (n) < m \leq (2t(n)-2)\ell (n)+|{\tau (n)}|$
. Then by (41) we have
![]() $m \geq 2 s(n) + |{\tau (n)}|$
. Arguing similarly as in the first case, we can find a word w with
$m \geq 2 s(n) + |{\tau (n)}|$
. Arguing similarly as in the first case, we can find a word w with
![]() $|{w}| = m$
of the form
$|{w}| = m$
of the form
where
![]() $1 \leq j,k \leq t(n)-1$
and
$1 \leq j,k \leq t(n)-1$
and
![]() $b_1,b_2,\ldots , b_j, b_1',b_2',\ldots , b_k' \in \mathcal {B}_n$
. Thus,
$b_1,b_2,\ldots , b_j, b_1',b_2',\ldots , b_k' \in \mathcal {B}_n$
. Thus,
![]() $uwv$
is a subword of the concatenation
$uwv$
is a subword of the concatenation
![]() $c_1c_2$
of two words
$c_1c_2$
of two words
![]() $c_1,c_2 \in \mathcal {B}_{n+1}$
. Hence,
$c_1,c_2 \in \mathcal {B}_{n+1}$
. Hence,
![]() $uwv$
is a subword of a word in
$uwv$
is a subword of a word in
![]() $\mathcal {B}_{n+2}$
and thus
$\mathcal {B}_{n+2}$
and thus
![]() $uwv \in \mathcal {B}(X)$
.
$uwv \in \mathcal {B}(X)$
.
Case 3. Suppose finally that
![]() $m> (t(n)-2)\ell (n) + \tau (n)$
. Put
$m> (t(n)-2)\ell (n) + \tau (n)$
. Put
 $$ \begin{align*} u' &= b_1b_2\cdots b_{t(n)-1} u \tau(n) \in \mathcal{B}_{n+1},\\ v' &= b_2'b_3' \cdots b_{t(n)}' \tau(n) \in \mathcal{B}_{n+1}, \end{align*} $$
$$ \begin{align*} u' &= b_1b_2\cdots b_{t(n)-1} u \tau(n) \in \mathcal{B}_{n+1},\\ v' &= b_2'b_3' \cdots b_{t(n)}' \tau(n) \in \mathcal{B}_{n+1}, \end{align*} $$
where
![]() $b_1,b_2,\ldots ,b_{t(n)-1},b_2',b_3',b_{t(n)}' \in \mathcal {B}_n$
are arbitrary. Put also
$b_1,b_2,\ldots ,b_{t(n)-1},b_2',b_3',b_{t(n)}' \in \mathcal {B}_n$
are arbitrary. Put also
![]() $n' = n+1$
and
$n' = n+1$
and
![]() ${m' = m-|{\tau (n)}|}$
. By (40) we have
${m' = m-|{\tau (n)}|}$
. By (40) we have
Hence, by the inductive assumption, there exists a word
![]() $w'$
with
$w'$
with
![]() $|{w'}| = m$
such that
$|{w'}| = m$
such that
![]() $u'w'v' \in \mathcal {B}(X)$
. It remains to observe that
$u'w'v' \in \mathcal {B}(X)$
. It remains to observe that
![]() $uwv$
is a subword of
$uwv$
is a subword of
![]() $u'w'v'$
, where
$u'w'v'$
, where
![]() $w = \tau (n) w$
has length
$w = \tau (n) w$
has length
![]() $|{w}| = |{\tau (n)}| + |{w'}|= m$
.
$|{w}| = |{\tau (n)}| + |{w'}|= m$
.
Theorem 5.11. There exists a sequence of positive integers
![]() $(t(n))_{n=1}^{\infty} $
such that X is minimal, mixing, and has positive entropy and
$(t(n))_{n=1}^{\infty} $
such that X is minimal, mixing, and has positive entropy and
![]() $\bar {d}$
-shadowing property. In particular, the shift space X has an entropy-dense and uncountable set of ergodic measures.
$\bar {d}$
-shadowing property. In particular, the shift space X has an entropy-dense and uncountable set of ergodic measures.
Proof. Since
![]() $X_1$
is a mixing sofic shift it has topological entropy
$X_1$
is a mixing sofic shift it has topological entropy
![]() $h>0$
. By the uniform continuity of the entropy function with respect to
$h>0$
. By the uniform continuity of the entropy function with respect to
![]() $\bar {d}_{\mathcal {M}}$
-distance, there is
$\bar {d}_{\mathcal {M}}$
-distance, there is
![]() $\varepsilon>0$
such that for every
$\varepsilon>0$
such that for every
![]() $\mu ,\mu '\in {\mathcal {M}_{\mathit \sigma }}(\{0,1\}^{\infty} )$
with
$\mu ,\mu '\in {\mathcal {M}_{\mathit \sigma }}(\{0,1\}^{\infty} )$
with
![]() $\bar {d}_{\mathcal {M}}(\mu ,\mu ')<\varepsilon $
we have |h(μ) − h(μ′)| < h/3. Fix this
$\bar {d}_{\mathcal {M}}(\mu ,\mu ')<\varepsilon $
we have |h(μ) − h(μ′)| < h/3. Fix this
![]() $\varepsilon $
.
$\varepsilon $
.
All the conditions (37), (38) with respect to
![]() $\varepsilon $
specified above, (40) and (41) can be satisfied simultaneously by one sequence
$\varepsilon $
specified above, (40) and (41) can be satisfied simultaneously by one sequence
![]() $t(n)$
,
$t(n)$
,
![]() $n\ge 1$
. Indeed, it is enough to construct the sequence inductively and take
$n\ge 1$
. Indeed, it is enough to construct the sequence inductively and take
![]() $t(n)$
large enough with respect to right-hand sides of the conditions which all depend only on the previously taken
$t(n)$
large enough with respect to right-hand sides of the conditions which all depend only on the previously taken
![]() $t(i)$
,
$t(i)$
,
![]() $i<n$
. Such a sequence then satisfies all assumptions of Propositions 5.9 and 5.10. Hence, X is mixing and
$i<n$
. Such a sequence then satisfies all assumptions of Propositions 5.9 and 5.10. Hence, X is mixing and
![]() $\bar {d}$
-approachable from above by mixing sofic shifts. In particular, the shift has an entropy-dense set of ergodic measure and is
$\bar {d}$
-approachable from above by mixing sofic shifts. In particular, the shift has an entropy-dense set of ergodic measure and is
![]() $\bar {d}$
-shadowing.
$\bar {d}$
-shadowing.
Now it suffices to find two invariant measures on X with different entropies. By the variational principle, there is an ergodic invariant measure
![]() $\nu _1$
on
$\nu _1$
on
![]() $X_1$
with entropy h. Since
$X_1$
with entropy h. Since
![]() $X_1$
contains a periodic point, there is also an ergodic invariant measure
$X_1$
contains a periodic point, there is also an ergodic invariant measure
![]() $\nu _2$
on
$\nu _2$
on
![]() $X_1$
of entropy zero. The inequality
$X_1$
of entropy zero. The inequality
![]() $\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}e(X_n),{\mathcal {M}_{\mathit \sigma }}e(X))<\varepsilon /2$
ensures that there is an invariant measure
$\bar {d}^{\mathrm {H}}_{\mathcal {M}}({\mathcal {M}_{\mathit \sigma }}e(X_n),{\mathcal {M}_{\mathit \sigma }}e(X))<\varepsilon /2$
ensures that there is an invariant measure
![]() $\nu ^{\prime }_1$
on X that is
$\nu ^{\prime }_1$
on X that is
![]() $\varepsilon $
-close to
$\varepsilon $
-close to
![]() $\nu _1$
in
$\nu _1$
in
![]() $\bar {d}_{\mathcal {M}}$
-distance and so
$\bar {d}_{\mathcal {M}}$
-distance and so
![]() $h/3$
-close to
$h/3$
-close to
![]() $\nu _1$
in entropy. By the same argument, there is an invariant measure
$\nu _1$
in entropy. By the same argument, there is an invariant measure
![]() $\nu ^{\prime }_2$
on X that is
$\nu ^{\prime }_2$
on X that is
![]() $h/3$
-close to
$h/3$
-close to
![]() $\nu _2$
in entropy. Since the difference between
$\nu _2$
in entropy. Since the difference between
![]() $h(\nu _1)$
and
$h(\nu _1)$
and
![]() $h(\nu _2)$
equals h, the measures
$h(\nu _2)$
equals h, the measures
![]() $\nu ^{\prime }_1$
and
$\nu ^{\prime }_1$
and
![]() $\nu ^{\prime }_2$
have different entropy and, in particular, are distinct.
$\nu ^{\prime }_2$
have different entropy and, in particular, are distinct.
Acknowledgments
The research cooperation between Michal Kupsa and Dominik Kwietniak was partially funded by the program Excellence Initiative—Research University under the Strategic Programme Excellence Initiative at Jagiellonian University in Kraków. The program supported the research stay of Michal Kupsa in Kraków in May–June 2023 during which the present paper was (almost) finished. The research of Melih Emin Can is part of the project no. 2021/43/P/ST1/02885 cofunded by the National Science Centre and the European Union’s Horizon 2020 research and innovation program under Marie Sklodowska-Curie grant agreement no. 945339. When this paper was being finished, D. Kwietniak was partially supported by the Flagship Project ‘Central European Mathematical Research Lab’ under the Strategic Programme Excellence Initiative at Jagiellonian University. J. Konieczny is supported by UKRI Fellowship EP/X033813/1, and during a significant part of the work on this project he was working within the framework of LABEX MILYON (ANR-10-LABX-0070) of Université de Lyon, within the program ‘Investissements d’Avenir’ (ANR-11-IDEX-0007) operated by the French National Research Agency (ANR). He also acknowledges support from the Foundation for Polish Science (FNP). The authors would like to express their gratitude to Tim Austin for sharing a very early version of his paper [Reference Austin1] and an enlightening discussion. We would like to thank Piotr Oprocha for allowing us to use his ideas that led to the construction of the minimal examples of
![]() $\bar {d}$
-approachable shift spaces in §5.2. Thanks are also due to Alexandre Trilles, who helped us to find and fix numerous minor faults in §§5.1 and 5.2. Melih Emin Can would like to thank Damla Buldağ Can for her constant love and support. Finally, we are grateful to the referee for a careful reading of our paper and for helpful corrections and suggestions.
$\bar {d}$
-approachable shift spaces in §5.2. Thanks are also due to Alexandre Trilles, who helped us to find and fix numerous minor faults in §§5.1 and 5.2. Melih Emin Can would like to thank Damla Buldağ Can for her constant love and support. Finally, we are grateful to the referee for a careful reading of our paper and for helpful corrections and suggestions.