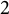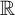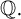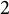This note was inspired by a perplexing remark that occurs in the classic 1960 treatise of Zariski and Samuel [Reference Zariski and Samuel15, p. 36], in the midst of a discussion of places and valuations of fields: “…The ambiguity may remain even if the value group
![]() $\Gamma $
is fixed, for
$\Gamma $
is fixed, for
![]() $\Gamma $
may very well possess non-identical order preserving automorphisms. It is well known that, with the exception of the additive group of integers, every totally-ordered abelian group does possess such automorphisms.”
$\Gamma $
may very well possess non-identical order preserving automorphisms. It is well known that, with the exception of the additive group of integers, every totally-ordered abelian group does possess such automorphisms.”
In fact, there are various known examples of totally ordered abelian groups without nontrivial order-preserving automorphisms. For example, A. M. W. Glass and P. Ribenboim [Reference Glass and Ribenboim10] have shown that the multiplicative group of positive rational numbers has trivial order-automorphism group. In this paper, we will construct new examples of totally ordered abelian groups with this property, en route to a classification of the order-automorphism group of every finitely generated Archimedean ordered group of rank
![]() $2.$
$2.$
As in [Reference Fuchs9], we define the rank of a torsion-free abelian group G as the cardinality of a maximal independent subset of G (equivalently, as the dimension of the
![]() $\hspace {-1.00pt} \mathbb {Q} \hspace {-1.00pt}$
-vector space
$\hspace {-1.00pt} \mathbb {Q} \hspace {-1.00pt}$
-vector space
![]() $G \otimes _{\mathbb {Z}} \mathbb {Q}$
). An ordered group G is said to be Archimedean if for all
$G \otimes _{\mathbb {Z}} \mathbb {Q}$
). An ordered group G is said to be Archimedean if for all
![]() $x, y \in G$
such that
$x, y \in G$
such that
![]() $x>1,$
there exists some
$x>1,$
there exists some
![]() $n \in \mathbb {N}$
such that
$n \in \mathbb {N}$
such that
![]() $x^n> y.$
It is known that every Archimedean group is abelian [Reference Cartan4].
$x^n> y.$
It is known that every Archimedean group is abelian [Reference Cartan4].
Example 1 Let
![]() $t \in \mathbb {R}$
satisfy
$t \in \mathbb {R}$
satisfy
![]() $[\mathbb {Q}(t) : \mathbb {Q}]> 2$
(possibly t transcendental). Let G be the subgroup of the additive group of real numbers generated by
$[\mathbb {Q}(t) : \mathbb {Q}]> 2$
(possibly t transcendental). Let G be the subgroup of the additive group of real numbers generated by
![]() $1$
and
$1$
and
![]() $t.$
Suppose
$t.$
Suppose
![]() $\psi \colon G \rightarrow G$
is any order-preserving group automorphism; we will show that
$\psi \colon G \rightarrow G$
is any order-preserving group automorphism; we will show that
![]() $\psi $
must be the identity map.
$\psi $
must be the identity map.
According to Hion’s Lemma [Reference Hion11, p. 238, Lemma 1], any order-preserving group homomorphism between two additive subgroups of
![]() $\mathbb {R},$
with the induced orderings, is given by multiplication by some nonnegative real number. Thus, there exists
$\mathbb {R},$
with the induced orderings, is given by multiplication by some nonnegative real number. Thus, there exists
![]() $g \in \mathbb {R}$
such that
$g \in \mathbb {R}$
such that
![]() $\psi (x) = gx$
for every
$\psi (x) = gx$
for every
![]() $x \in G.$
Taking
$x \in G.$
Taking
![]() $x=1,$
we see that
$x=1,$
we see that
![]() $g \in G.$
Write
$g \in G.$
Write
![]() $g = a + bt$
for some
$g = a + bt$
for some
![]() $a, b \in \mathbb {Z}.$
Since the element
$a, b \in \mathbb {Z}.$
Since the element
![]() $\psi (t) = at + bt^{2}$
is contained in
$\psi (t) = at + bt^{2}$
is contained in
![]() $G,$
and
$G,$
and
![]() $[\mathbb {Q}(t) : \mathbb {Q}]> 2,$
we must have
$[\mathbb {Q}(t) : \mathbb {Q}]> 2,$
we must have
![]() $b = 0.$
The surjectivity of
$b = 0.$
The surjectivity of
![]() $\psi $
now forces
$\psi $
now forces
![]() $g = a = \pm 1,$
and since
$g = a = \pm 1,$
and since
![]() $\psi $
is order-preserving,
$\psi $
is order-preserving,
![]() $g = 1.$
$g = 1.$
Thus, G is a totally ordered abelian group with no nonidentity order-preserving group automorphisms.
We will now show that in Example 1 the condition
![]() $[\mathbb {Q}(t) : \mathbb {Q}]> 2$
is indispensable. We begin with the following special case.
$[\mathbb {Q}(t) : \mathbb {Q}]> 2$
is indispensable. We begin with the following special case.
Lemma 2 Let
![]() $t \in \mathbb {R}$
satisfy
$t \in \mathbb {R}$
satisfy
![]() $[\mathbb {Q}(t) : \mathbb {Q}] = 2.$
Assume, in addition, that t is an algebraic integer. Let G be the subgroup of the additive group of real numbers generated by
$[\mathbb {Q}(t) : \mathbb {Q}] = 2.$
Assume, in addition, that t is an algebraic integer. Let G be the subgroup of the additive group of real numbers generated by
![]() $1$
and
$1$
and
![]() $t.$
Let A be the group of order-preserving automorphisms of
$t.$
Let A be the group of order-preserving automorphisms of
![]() $G.$
Then A is infinite cyclic.
$G.$
Then A is infinite cyclic.
Proof Let t be a root of the irreducible polynomial
![]() $p(x) = x^2 + bx + c \in \mathbb {Z}[x],$
where
$p(x) = x^2 + bx + c \in \mathbb {Z}[x],$
where
![]() $d = b^2 - 4c$
is a positive integer that is not a perfect square. Note that G is equal to the underlying additive group of the ring
$d = b^2 - 4c$
is a positive integer that is not a perfect square. Note that G is equal to the underlying additive group of the ring
![]() $\mathbb {Z}[t].$
Now,
$\mathbb {Z}[t].$
Now,
where
![]() $\mathcal {O}$
is the ring of integers of the quadratic number field
$\mathcal {O}$
is the ring of integers of the quadratic number field
![]() $\mathbb {Q}(\!\sqrt {d}\,).$
The group
$\mathbb {Q}(\!\sqrt {d}\,).$
The group
![]() $\operatorname {U}(\mathcal {O})$
of units of
$\operatorname {U}(\mathcal {O})$
of units of
![]() $\mathcal {O}$
is isomorphic to
$\mathcal {O}$
is isomorphic to
![]() $C_2 \oplus C_{\infty },$
where
$C_2 \oplus C_{\infty },$
where
![]() $C_k$
denotes the cyclic group of order
$C_k$
denotes the cyclic group of order
![]() $k.$
It is well known that the unit group
$k.$
It is well known that the unit group
![]() $\operatorname {U}(\mathbb {Z}[\!\sqrt {d}\,])$
takes the same form, even in the case where
$\operatorname {U}(\mathbb {Z}[\!\sqrt {d}\,])$
takes the same form, even in the case where
![]() $\mathbb {Z}[\!\sqrt {d}\,] \subsetneqq \mathcal {O}.$
(An elementary proof can be found in [Reference Baker1, pp. 64–65], observing that the argument there, given for
$\mathbb {Z}[\!\sqrt {d}\,] \subsetneqq \mathcal {O}.$
(An elementary proof can be found in [Reference Baker1, pp. 64–65], observing that the argument there, given for
![]() $\mathcal {O},$
applies equally to
$\mathcal {O},$
applies equally to
![]() $\mathbb {Z}[\!\sqrt {d}\,].$
) Consequently,
$\mathbb {Z}[\!\sqrt {d}\,].$
) Consequently,
![]() $\operatorname {U}(\mathbb {Z}[t]) = \{\pm \eta ^m\colon \ m \in \mathbb {Z}\}$
for some element
$\operatorname {U}(\mathbb {Z}[t]) = \{\pm \eta ^m\colon \ m \in \mathbb {Z}\}$
for some element
![]() $\eta> 1$
in
$\eta> 1$
in
![]() $\mathbb {Z}[t].$
$\mathbb {Z}[t].$
For each
![]() $m \in \mathbb {Z},$
multiplication by
$m \in \mathbb {Z},$
multiplication by
![]() $\eta ^m$
induces a distinct order-preserving automorphism of
$\eta ^m$
induces a distinct order-preserving automorphism of
![]() $G.$
By Hion’s Lemma, every element of A is of this form.
$G.$
By Hion’s Lemma, every element of A is of this form.
Remark In the case where d is square-free and
![]() $\mathbb {Z}[\!\sqrt {d}\,] \subsetneqq \mathcal {O},$
the group
$\mathbb {Z}[\!\sqrt {d}\,] \subsetneqq \mathcal {O},$
the group
![]() $\operatorname {U}(\mathbb {Z}[\!\sqrt {d}\,])$
is either equal to
$\operatorname {U}(\mathbb {Z}[\!\sqrt {d}\,])$
is either equal to
![]() $\operatorname {U}(\mathcal {O})$
or else it is a subgroup of index
$\operatorname {U}(\mathcal {O})$
or else it is a subgroup of index
![]() $3.$
A necessary condition for
$3.$
A necessary condition for
![]() $[\operatorname {U}(\mathcal {O}) : \operatorname {U}(\mathbb {Z}[\!\sqrt {d}\,])] = 3$
is
$[\operatorname {U}(\mathcal {O}) : \operatorname {U}(\mathbb {Z}[\!\sqrt {d}\,])] = 3$
is
![]() $d \equiv 5 \,\operatorname {mod}\, 8.$
This index
$d \equiv 5 \,\operatorname {mod}\, 8.$
This index
![]() $3$
scenario amounts to the Diophantine equation
$3$
scenario amounts to the Diophantine equation
![]() $x^2 - dy^2 = \pm 4$
having solutions where x and y are both odd. As proved in [Reference Williams and Buck14], there are infinitely many values of d for which this occurs, but a complete classification does not seem to be known.
$x^2 - dy^2 = \pm 4$
having solutions where x and y are both odd. As proved in [Reference Williams and Buck14], there are infinitely many values of d for which this occurs, but a complete classification does not seem to be known.
The general case of a subgroup of
![]() $\mathbb {R}$
generated by
$\mathbb {R}$
generated by
![]() $1$
and an algebraic element of degree
$1$
and an algebraic element of degree
![]() $2$
can be reduced to the case covered by Lemma 2, as we will now prove. The following theorem should be contrasted with Example 1, where there were no nontrivial order-preserving group automorphisms.
$2$
can be reduced to the case covered by Lemma 2, as we will now prove. The following theorem should be contrasted with Example 1, where there were no nontrivial order-preserving group automorphisms.
Theorem 3 Let t be a real number such that
![]() $[\mathbb {Q}(t) : \mathbb {Q}] = 2,$
and let G be the ordered additive subgroup of
$[\mathbb {Q}(t) : \mathbb {Q}] = 2,$
and let G be the ordered additive subgroup of
![]() $\mathbb {R}$
generated by
$\mathbb {R}$
generated by
![]() $1$
and
$1$
and
![]() $t.$
Let A be the group of order-preserving automorphisms of
$t.$
Let A be the group of order-preserving automorphisms of
![]() $G.$
Then A is infinite cyclic.
$G.$
Then A is infinite cyclic.
Proof The set of
![]() $n \in \mathbb {Z}$
for which
$n \in \mathbb {Z}$
for which
![]() $nt$
is an algebraic integer is a nonzero ideal of
$nt$
is an algebraic integer is a nonzero ideal of
![]() $\mathbb {Z}.$
Let
$\mathbb {Z}.$
Let
![]() $z \in \mathbb {N}$
generate this ideal. Put
$z \in \mathbb {N}$
generate this ideal. Put
![]() $\alpha = zt$
and
$\alpha = zt$
and
![]() $H = \mathbb {Z} \oplus \mathbb {Z}\alpha .$
By Lemma 2, the group B of order-preserving automorphisms of H is infinite cyclic, generated by multiplication by a unit of the ring
$H = \mathbb {Z} \oplus \mathbb {Z}\alpha .$
By Lemma 2, the group B of order-preserving automorphisms of H is infinite cyclic, generated by multiplication by a unit of the ring
![]() $R = \mathbb {Z}[\alpha ].$
We will show that A is isomorphic to a nontrivial subgroup of
$R = \mathbb {Z}[\alpha ].$
We will show that A is isomorphic to a nontrivial subgroup of
![]() $B.$
$B.$
The first step is to prove that A is isomorphic to a subgroup of
![]() $B.$
Hion’s Lemma implies that any nonidentity element of A is induced by multiplication by a real number
$B.$
Hion’s Lemma implies that any nonidentity element of A is induced by multiplication by a real number
![]() $m + nt$
for some
$m + nt$
for some
![]() $m, n \in \mathbb {Z}$
with
$m, n \in \mathbb {Z}$
with
![]() $n \neq 0.$
Then
$n \neq 0.$
Then
![]() $(m + nt)t \in G = \mathbb {Z} \oplus \mathbb {Z}t$
implies
$(m + nt)t \in G = \mathbb {Z} \oplus \mathbb {Z}t$
implies
![]() $nt^2 \in \mathbb {Z} \oplus \mathbb {Z}t,$
which implies that
$nt^2 \in \mathbb {Z} \oplus \mathbb {Z}t,$
which implies that
![]() $nt$
is an algebraic integer. Hence
$nt$
is an algebraic integer. Hence
![]() $z \mid n$
in
$z \mid n$
in
![]() $\mathbb {Z}.$
It follows that
$\mathbb {Z}.$
It follows that
![]() $m + nt$
is an (invertible) element of the ring
$m + nt$
is an (invertible) element of the ring
![]() $R.$
Thus, A embeds in
$R.$
Thus, A embeds in
![]() $B.$
$B.$
The final step is to show that A is nontrivial. Choose
![]() $a, b, c \in \mathbb {Z}$
such that
$a, b, c \in \mathbb {Z}$
such that
![]() $at^2 + bt + c = 0.$
As an ordered group,
$at^2 + bt + c = 0.$
As an ordered group,
![]() $G = \mathbb {Z} \oplus \mathbb {Z} t \cong \mathbb {Z}a \oplus \mathbb {Z} at \subseteq \mathbb {Z} \oplus \mathbb {Z} at.$
Since
$G = \mathbb {Z} \oplus \mathbb {Z} t \cong \mathbb {Z}a \oplus \mathbb {Z} at \subseteq \mathbb {Z} \oplus \mathbb {Z} at.$
Since
![]() $at$
is an algebraic integer, by Lemma 2 the group of order-preserving automorphisms of
$at$
is an algebraic integer, by Lemma 2 the group of order-preserving automorphisms of
![]() $\mathbb {Z} \oplus \mathbb {Z} at$
is infinite cyclic, and multiplication by any positive unit
$\mathbb {Z} \oplus \mathbb {Z} at$
is infinite cyclic, and multiplication by any positive unit
![]() $\tilde {u}$
of the ring
$\tilde {u}$
of the ring
![]() $\mathbb {Z}[at]$
leaves the subgroup
$\mathbb {Z}[at]$
leaves the subgroup
![]() $\mathbb {Z}a \oplus \mathbb {Z} at \subseteq \mathbb {Z} \oplus \mathbb {Z} at$
invariant. Thus the group of order-preserving automorphisms of
$\mathbb {Z}a \oplus \mathbb {Z} at \subseteq \mathbb {Z} \oplus \mathbb {Z} at$
invariant. Thus the group of order-preserving automorphisms of
![]() $\mathbb {Z} \oplus \mathbb {Z} at$
embeds in
$\mathbb {Z} \oplus \mathbb {Z} at$
embeds in
![]() $A,$
which completes the proof.
$A,$
which completes the proof.
We can now extend Example 1. The following theorem incorporates the non-finitely-generated case. Its proof makes use of Hölder’s theorem [Reference Hölder12], which states that every Archimedean group is order-isomorphic to an additive subgroup of
![]() $\mathbb {R}.$
A concise proof of Hölder’s theorem can be found in [Reference Botto Mura and Rhemtulla2, pp. 10–11].
$\mathbb {R}.$
A concise proof of Hölder’s theorem can be found in [Reference Botto Mura and Rhemtulla2, pp. 10–11].
Theorem 4 Let G be an Archimedean group of rank
![]() $2,$
and let
$2,$
and let
![]() $\{\alpha , \beta \} \subset G$
be a maximal independent set. There is an injective order-preserving group homomorphism
$\{\alpha , \beta \} \subset G$
be a maximal independent set. There is an injective order-preserving group homomorphism
![]() $G \rightarrow \mathbb {R};$
identifying G with the image, suppose
$G \rightarrow \mathbb {R};$
identifying G with the image, suppose
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2.$
Let
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2.$
Let
Let A be the group of order-preserving automorphisms of
![]() $G.$
Then A is free abelian of rank
$G.$
Then A is free abelian of rank
![]() $| \mathcal {P} |.$
In particular, if
$| \mathcal {P} |.$
In particular, if
![]() $\mathcal {P} = {\varnothing }$
then A is trivial.
$\mathcal {P} = {\varnothing }$
then A is trivial.
Proof By Hölder’s theorem, G is order-isomorphic to an additive subgroup of
![]() $\mathbb {R}.$
Letting
$\mathbb {R}.$
Letting
![]() $t = \alpha /\beta $
and replacing G by an order-isomorphic copy in which
$t = \alpha /\beta $
and replacing G by an order-isomorphic copy in which
![]() $\{1, t\}$
is a maximal independent set, we may assume
$\{1, t\}$
is a maximal independent set, we may assume
By the argument from Example 1, any order-preserving automorphism of G is of the form
![]() $\psi _g,$
for some
$\psi _g,$
for some
![]() $g \in \mathbb {Q}_{>0} \cap G,$
defined by
$g \in \mathbb {Q}_{>0} \cap G,$
defined by
![]() $\psi _g(x) = gx.$
For such a
$\psi _g(x) = gx.$
For such a
![]() $\psi _g,$
write
$\psi _g,$
write
![]() $g=m/n$
where
$g=m/n$
where
![]() $m,n \in \mathbb {N}$
are relatively prime. From
$m,n \in \mathbb {N}$
are relatively prime. From
![]() $gG = G$
we obtain
$gG = G$
we obtain
![]() $mG = nG.$
Then
$mG = nG.$
Then
![]() $\gcd (m,n) = 1$
implies that G is both
$\gcd (m,n) = 1$
implies that G is both
![]() $\hspace {-1.00pt} m \hspace {-1.00pt}$
-divisible and
$\hspace {-1.00pt} m \hspace {-1.00pt}$
-divisible and
![]() $\hspace {-1.00pt} n \hspace {-1.00pt}$
-divisible. In particular, every prime divisor of n belongs to
$\hspace {-1.00pt} n \hspace {-1.00pt}$
-divisible. In particular, every prime divisor of n belongs to
![]() $\mathcal {P}.$
We infer that the map
$\mathcal {P}.$
We infer that the map
![]() $\psi _g \mapsto g$
defines an isomorphism from A to the multiplicative subgroup of
$\psi _g \mapsto g$
defines an isomorphism from A to the multiplicative subgroup of
![]() $\mathbb {Q}_{>0}$
generated by
$\mathbb {Q}_{>0}$
generated by
![]() $\mathcal {P}.$
The theorem follows.
$\mathcal {P}.$
The theorem follows.
Note that for every
![]() $n \in \mathbb {N} \cup \{\infty \}$
there exists an Archimedean group G of rank
$n \in \mathbb {N} \cup \{\infty \}$
there exists an Archimedean group G of rank
![]() $2$
whose group of order-preserving automorphisms is free abelian of rank
$2$
whose group of order-preserving automorphisms is free abelian of rank
![]() $n.$
For an explicit example in the case
$n.$
For an explicit example in the case
![]() $n < \infty ,$
let
$n < \infty ,$
let
![]() $\{p_1, \ldots , p_n\}$
be a set of n distinct prime numbers, let
$\{p_1, \ldots , p_n\}$
be a set of n distinct prime numbers, let
![]() $p = p_1\ldots p_n$
be their product, and take
$p = p_1\ldots p_n$
be their product, and take
![]() $G \subset \mathbb {R}$
to be the additive group
$G \subset \mathbb {R}$
to be the additive group
![]() $G = \mathbb {Z}[1/p] + \mathbb {Z}[1/p] \pi .$
In the case
$G = \mathbb {Z}[1/p] + \mathbb {Z}[1/p] \pi .$
In the case
![]() $n = \infty ,$
one can take
$n = \infty ,$
one can take
![]() $G = \mathbb {Q} + \mathbb {Q} \pi .$
$G = \mathbb {Q} + \mathbb {Q} \pi .$
The classification of order-automorphism groups of rank
![]() $2$
Archimedean groups in Theorem 4 might be compared with results and techniques from A. M. W. Glass and P. Ribenboim’s paper [Reference Glass and Ribenboim10], mentioned in the introduction. Glass and Ribenboim show that every subgroup of the multiplicative group of positive real algebraic numbers has order-automorphism group isomorphic to a subgroup of the multiplicative group of positive rational numbers. The proof is based on an application of Hion’s lemma along with results in transcendental number theory. Glass and Ribenboim go on to produce a wide array of ordered abelian groups with trivial order-automorphism groups. For example, they show that if K is any algebraic number field, then any subgroup of the multiplicative group of positive real elements of K has trivial order-automorphism group. The contrast between that result and Theorem 3 of the present work highlights differences between the additive structure of K and the multiplicative structure of
$2$
Archimedean groups in Theorem 4 might be compared with results and techniques from A. M. W. Glass and P. Ribenboim’s paper [Reference Glass and Ribenboim10], mentioned in the introduction. Glass and Ribenboim show that every subgroup of the multiplicative group of positive real algebraic numbers has order-automorphism group isomorphic to a subgroup of the multiplicative group of positive rational numbers. The proof is based on an application of Hion’s lemma along with results in transcendental number theory. Glass and Ribenboim go on to produce a wide array of ordered abelian groups with trivial order-automorphism groups. For example, they show that if K is any algebraic number field, then any subgroup of the multiplicative group of positive real elements of K has trivial order-automorphism group. The contrast between that result and Theorem 3 of the present work highlights differences between the additive structure of K and the multiplicative structure of
![]() $K.$
$K.$
Returning to Theorem 4, we note that the hypothesis
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2$
is indispensable, as the following examples show.
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2$
is indispensable, as the following examples show.
Example 5 Localize the ring
![]() $R = \mathbb {Z}[\!\sqrt {2}\,]$
at the set
$R = \mathbb {Z}[\!\sqrt {2}\,]$
at the set
![]() $S = \{ (1+2\sqrt {2}\,)^n\colon \ n \in \mathbb {N} \}$
to obtain
$S = \{ (1+2\sqrt {2}\,)^n\colon \ n \in \mathbb {N} \}$
to obtain
![]() $T = S^{-1}R.$
Let G be the underlying additive group of the ring
$T = S^{-1}R.$
Let G be the underlying additive group of the ring
![]() $T.$
Let H be the underlying additive group of the ring
$T.$
Let H be the underlying additive group of the ring
![]() $R.$
Both G and its subgroup H are Archimedean groups of rank
$R.$
Both G and its subgroup H are Archimedean groups of rank
![]() $2.$
Neither G nor H is
$2.$
Neither G nor H is
![]() $\hspace {-1.00pt} p \hspace {-1.00pt}$
-divisible for any prime number
$\hspace {-1.00pt} p \hspace {-1.00pt}$
-divisible for any prime number
![]() $p.$
Thus, the set
$p.$
Thus, the set
![]() $\mathcal {P}$
defined in Theorem 4 is empty, both for G and when G is replaced by
$\mathcal {P}$
defined in Theorem 4 is empty, both for G and when G is replaced by
![]() $H.$
But the groups of order-preserving automorphisms of G and H are different, and neither is trivial. So the conclusion of Theorem 4 is false here, and indeed the conditions in Theorem 4 are insufficient to distinguish the order-automorphism groups. Let
$H.$
But the groups of order-preserving automorphisms of G and H are different, and neither is trivial. So the conclusion of Theorem 4 is false here, and indeed the conditions in Theorem 4 are insufficient to distinguish the order-automorphism groups. Let
A direct calculation shows that the group of order-preserving automorphisms of G is free abelian of rank
![]() $2,$
generated by the elements
$2,$
generated by the elements
![]() $\psi _{\varepsilon }$
and
$\psi _{\varepsilon }$
and
![]() $\psi _v$
defined by
$\psi _v$
defined by
![]() $\psi _{\varepsilon }(x) = \varepsilon x$
and
$\psi _{\varepsilon }(x) = \varepsilon x$
and
![]() $\psi _v(x) = vx.$
On the other hand, the group of order-preserving automorphisms of H is free abelian of rank
$\psi _v(x) = vx.$
On the other hand, the group of order-preserving automorphisms of H is free abelian of rank
![]() $1,$
generated by the element
$1,$
generated by the element
![]() $\psi _{\varepsilon }.$
$\psi _{\varepsilon }.$
Example 6 Analogously to the construction in the previous example, let
![]() $R = \mathbb {Z}[\!\sqrt {79}\,],$
localize R at the set
$R = \mathbb {Z}[\!\sqrt {79}\,],$
localize R at the set
![]() $S = \{ 3^m7^n\colon \ m,n \in \mathbb {N} \},$
and let G be the underlying additive group of the ring
$S = \{ 3^m7^n\colon \ m,n \in \mathbb {N} \},$
and let G be the underlying additive group of the ring
![]() $S^{-1} R.$
The class group of
$S^{-1} R.$
The class group of
![]() $\mathbb {Q}(\!\sqrt {79}\,)$
has order
$\mathbb {Q}(\!\sqrt {79}\,)$
has order
![]() $3;$
in particular, unlike in Example 5, R does not have unique factorization. A fundamental unit of
$3;$
in particular, unlike in Example 5, R does not have unique factorization. A fundamental unit of
![]() $\mathbb {Q}(\!\sqrt {79}\,)$
is
$\mathbb {Q}(\!\sqrt {79}\,)$
is
![]() $\varepsilon = 80+9\sqrt {79}\,.$
Let
$\varepsilon = 80+9\sqrt {79}\,.$
Let
![]() $\alpha = 10+\sqrt {79}$
and
$\alpha = 10+\sqrt {79}$
and
![]() $\beta = 10-\sqrt {79}\,.$
As before, let
$\beta = 10-\sqrt {79}\,.$
As before, let
![]() $\psi _a\colon G \rightarrow G$
denote the map given by multiplication by the element
$\psi _a\colon G \rightarrow G$
denote the map given by multiplication by the element
![]() $a \in R.$
The group of order-preserving automorphisms of G is generated by
$a \in R.$
The group of order-preserving automorphisms of G is generated by
subject to the relation
![]() $\psi _3\psi _7 = \psi _{\alpha }\psi _{\beta }.$
This group is free abelian of rank
$\psi _3\psi _7 = \psi _{\alpha }\psi _{\beta }.$
This group is free abelian of rank
![]() $4.$
$4.$
We now completely classify the order-automorphism groups of finitely generated Archimedean groups of rank
![]() $2.$
$2.$
Theorem 7 Let G be a finitely generated Archimedean group of rank
![]() $2,$
and let A be the group of order-preserving automorphisms of
$2,$
and let A be the group of order-preserving automorphisms of
![]() $G.$
Then G is isomorphic to an additive subgroup
$G.$
Then G is isomorphic to an additive subgroup
![]() $\langle \alpha , \beta \rangle $
of
$\langle \alpha , \beta \rangle $
of
![]() $\mathbb {R},$
and
$\mathbb {R},$
and
 $$ \begin{align*}A \cong \begin{cases} \;\mathbb{Z} & \text{if }\hspace{1.00pt} [\mathbb{Q}(\alpha/\beta) : \mathbb{Q}] = 2 \\ \{0\} & \text{if }\hspace{1.00pt} [\mathbb{Q}(\alpha/\beta) : \mathbb{Q}]> 2. \end{cases}\end{align*} $$
$$ \begin{align*}A \cong \begin{cases} \;\mathbb{Z} & \text{if }\hspace{1.00pt} [\mathbb{Q}(\alpha/\beta) : \mathbb{Q}] = 2 \\ \{0\} & \text{if }\hspace{1.00pt} [\mathbb{Q}(\alpha/\beta) : \mathbb{Q}]> 2. \end{cases}\end{align*} $$
Proof The case
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2$
follows from Theorem 4. The case
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2$
follows from Theorem 4. The case
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}] = 2$
is Theorem 3.
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}] = 2$
is Theorem 3.
If we drop the hypothesis that G is finitely generated, the simple characterization from Theorem 7 of the group of order-preserving automorphisms of G is not available; however, we do have the following general result.
Theorem 8 Let G be an Archimedean group of rank
![]() $2.$
Then the group of order-preserving automorphisms of G is a free abelian group.
$2.$
Then the group of order-preserving automorphisms of G is a free abelian group.
Proof By Hölder’s theorem, we may assume that G is an additive subgroup of
![]() $\mathbb {R}.$
Let
$\mathbb {R}.$
Let
![]() $\{\alpha , \beta \} \subset G$
be a maximal independent set. Theorem 4 covers the case
$\{\alpha , \beta \} \subset G$
be a maximal independent set. Theorem 4 covers the case
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2.$
In the case
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}]> 2.$
In the case
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}] = 2,$
the same argument shows that the group A of order-preserving automorphisms of G embeds in the multiplicative group consisting of all positive elements of the real quadratic number field
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}] = 2,$
the same argument shows that the group A of order-preserving automorphisms of G embeds in the multiplicative group consisting of all positive elements of the real quadratic number field
![]() $\mathbb {Q}(\alpha /\beta ) \subset \mathbb {R}.$
It is well known that the multiplicative group of any algebraic number field is free abelian modulo torsion [Reference Čarin5]. Thus, in the case
$\mathbb {Q}(\alpha /\beta ) \subset \mathbb {R}.$
It is well known that the multiplicative group of any algebraic number field is free abelian modulo torsion [Reference Čarin5]. Thus, in the case
![]() $[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}] = 2, A$
is a free abelian group.
$[\mathbb {Q}(\alpha /\beta ) : \mathbb {Q}] = 2, A$
is a free abelian group.
In the case of Theorem 8 where the group is not finitely generated (so Theorem 7 does not apply) and the extension field is quadratic (so Theorem 4 does not apply), the rank of the free abelian group of order-preserving automorphisms is determined by divisibility by irreducible elements of the ring of integers of the quadratic number field, where the rank can drop due to relations if unique factorization fails. Examples 5 and 6 are prototypes.
Although the primary focus of this note is Archimedean groups, it bears mention that Theorem 8 admits counterexamples absent the Archimedean hypothesis, such as the following.
Example 9 Let
![]() $G = \mathbb {Q} \oplus \mathbb {Q}$
as an additive group, with the lexicographic order on elements. Then G is a non-Archimedean ordered abelian group of rank
$G = \mathbb {Q} \oplus \mathbb {Q}$
as an additive group, with the lexicographic order on elements. Then G is a non-Archimedean ordered abelian group of rank
![]() $2.$
The group of order-preserving automorphisms of G is isomorphic to
$2.$
The group of order-preserving automorphisms of G is isomorphic to
 $$ \begin{align*}\left\{ \left(\begin{matrix} a & b \\ 0 & c \end{matrix}\right) \colon\ a,b,c \in \mathbb{Q}, \ a>0, \ c>0 \right\}\end{align*} $$
$$ \begin{align*}\left\{ \left(\begin{matrix} a & b \\ 0 & c \end{matrix}\right) \colon\ a,b,c \in \mathbb{Q}, \ a>0, \ c>0 \right\}\end{align*} $$
under the operation of matrix multiplication. This is not even an abelian group, much less free abelian.
Nevertheless, the study of order-preserving automorphisms of Archimedean groups has applications in a more general context. A totally ordered group G has Archimedean components, as defined in [Reference Conrad6], and the structure of the group of order-preserving automorphisms of G has been analyzed via the components of
![]() $G,$
for example, by P. F. Conrad [Reference Conrad7, Reference Conrad8] and more recently by R. H. Lafuente-Rodríguez [Reference Lafuente-Rodríguez13].
$G,$
for example, by P. F. Conrad [Reference Conrad7, Reference Conrad8] and more recently by R. H. Lafuente-Rodríguez [Reference Lafuente-Rodríguez13].
Returning, finally, to the perplexing remark cited at the beginning of this paper, “with the exception of the additive group of integers, every totally-ordered abelian group does possess [nonidentity order-preserving] automorphisms,” one might ask whether the quoted assertion is at least correct in the context in which it occurs in [Reference Zariski and Samuel15], namely, when the totally-ordered abelian groups under consideration are value groups of valuations on (commutative) fields. The answer is still no. As observed in [Reference Bourbaki3, Chapter VI, Section 3.4], every ordered abelian group occurs as the value group of a valuation on a field; indeed, every finitely generated Archimedean group of rank
![]() $2$
occurs as the value group of a valuation on a rational function field in two indeterminates.
$2$
occurs as the value group of a valuation on a rational function field in two indeterminates.