Article contents
CUTOFF AT THE ENTROPIC TIME FOR RANDOM WALKS ON COVERED EXPANDER GRAPHS
Published online by Cambridge University Press: 09 February 2021
Abstract
It is a fact simple to establish that the mixing time of the simple random walk on a d-regular graph 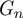 $G_n$ with n vertices is asymptotically bounded from below by
$G_n$ with n vertices is asymptotically bounded from below by 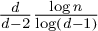 $\frac {d }{d-2 } \frac {\log n}{\log (d-1)}$. Such a bound is obtained by comparing the walk on
$\frac {d }{d-2 } \frac {\log n}{\log (d-1)}$. Such a bound is obtained by comparing the walk on 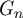 $G_n$ to the walk on d-regular tree
$G_n$ to the walk on d-regular tree  $\mathcal{T}_d$. If one can map another transitive graph
$\mathcal{T}_d$. If one can map another transitive graph 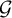 $\mathcal{G} $ onto
$\mathcal{G} $ onto 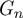 $G_n$, then we can improve the strategy by using a comparison with the random walk on
$G_n$, then we can improve the strategy by using a comparison with the random walk on 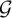 $\mathcal{G} $ (instead of that of
$\mathcal{G} $ (instead of that of 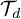 $\mathcal{T} _d$), and we obtain a lower bound of the form
$\mathcal{T} _d$), and we obtain a lower bound of the form 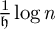 $\frac {1}{\mathfrak{h} }\log n$, where
$\frac {1}{\mathfrak{h} }\log n$, where  $\mathfrak{h} $ is the entropy rate associated with
$\mathfrak{h} $ is the entropy rate associated with 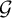 $\mathcal{G} $. We call this the entropic lower bound.
$\mathcal{G} $. We call this the entropic lower bound.
It was recently proved that in the case 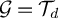 $\mathcal{G} =\mathcal{T} _d$, this entropic lower bound (in that case
$\mathcal{G} =\mathcal{T} _d$, this entropic lower bound (in that case 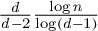 $\frac {d }{d-2 } \frac {\log n}{\log (d-1)}$) is sharp when graphs have minimal spectral radius and thus that in that case the random walk exhibits cutoff at the entropic time. In this article, we provide a generalisation of the result by providing a sufficient condition on the spectra of the random walks on
$\frac {d }{d-2 } \frac {\log n}{\log (d-1)}$) is sharp when graphs have minimal spectral radius and thus that in that case the random walk exhibits cutoff at the entropic time. In this article, we provide a generalisation of the result by providing a sufficient condition on the spectra of the random walks on 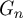 $G_n$ under which the random walk exhibits cutoff at the entropic time. It applies notably to anisotropic random walks on random d-regular graphs and to random walks on random n-lifts of a base graph (including nonreversible walks).
$G_n$ under which the random walk exhibits cutoff at the entropic time. It applies notably to anisotropic random walks on random d-regular graphs and to random walks on random n-lifts of a base graph (including nonreversible walks).
MSC classification
Information
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 21 , Issue 5 , September 2022 , pp. 1571 - 1616
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
- 8
- Cited by


