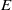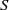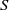Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
FAN, XINGKUI
CHEN, QIANHUA
and
KONG, XIANGJUN
2016.
COMPLETE LATTICE HOMOMORPHISM OF STRONGLY REGULAR CONGRUENCES ON -INVERSIVE SEMIGROUPS.
Journal of the Australian Mathematical Society,
Vol. 100,
Issue. 2,
p.
199.
Fan, Xingkui
Chen, Qianhua
and
Kong, Xiangjun
2016.
Weak inverses modulo Green’s relation $$\mathcal{{H}}$$ H on E-inversive and group-closed semigroups.
Semigroup Forum,
Vol. 92,
Issue. 3,
p.
691.