Published online by Cambridge University Press: 04 December 2014
In this paper we give explicit formulas for differential characteristic classes of principal 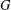 $G$-bundles with connections and prove their expected properties. In particular, we obtain explicit formulas for differential Chern classes, differential Pontryagin classes and the differential Euler class. Furthermore, we show that the differential Chern class is the unique natural transformation from (Simons–Sullivan) differential
$G$-bundles with connections and prove their expected properties. In particular, we obtain explicit formulas for differential Chern classes, differential Pontryagin classes and the differential Euler class. Furthermore, we show that the differential Chern class is the unique natural transformation from (Simons–Sullivan) differential 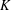 $K$-theory to (Cheeger–Simons) differential characters that is compatible with curvature and characteristic class. We also give the explicit formula for the differential Chern class on Freed–Lott differential
$K$-theory to (Cheeger–Simons) differential characters that is compatible with curvature and characteristic class. We also give the explicit formula for the differential Chern class on Freed–Lott differential 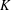 $K$-theory. Finally, we discuss the odd differential Chern classes.
$K$-theory. Finally, we discuss the odd differential Chern classes.