Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Calvaruso, Giovanni
and
Perrone, Domenico
2011.
Harmonic morphisms and Riemannian geometry of tangent bundles.
Annals of Global Analysis and Geometry,
Vol. 39,
Issue. 2,
p.
187.
CHUN, SUN HYANG
PARK, JEONGHYEONG
and
SEKIGAWA, KOUEI
2011.
H-CONTACT UNIT TANGENT SPHERE BUNDLES OF FOUR-DIMENSIONAL RIEMANNIAN MANIFOLDS.
Journal of the Australian Mathematical Society,
Vol. 91,
Issue. 2,
p.
243.
Markellos, Michael
2012.
The harmonicity of the Reeb vector field with respect to Riemannian g-natural metrics.
Differential Geometry and its Applications,
Vol. 30,
Issue. 3,
p.
274.
Calvaruso, Giovanni
and
Perrone, Domenico
2013.
Geometry of Kaluza–Klein metrics on the sphere
$${\mathbb{S}^3}$$
.
Annali di Matematica Pura ed Applicata,
Vol. 192,
Issue. 5,
p.
879.
Perrone, Domenico
2013.
Geodesic Ricci solitons on unit tangent sphere bundles.
Annals of Global Analysis and Geometry,
Vol. 44,
Issue. 2,
p.
91.
Calvaruso, Giovanni
and
Perrone, Domenico
2014.
Metrics of Kaluza–Klein type on the anti‐de Sitter space .
Mathematische Nachrichten,
Vol. 287,
Issue. 8-9,
p.
885.
Calvaruso, Giovanni
and
Martín-Molina, Verónica
2015.
Paracontact metric structures on the unit tangent sphere bundle.
Annali di Matematica Pura ed Applicata (1923 -),
Vol. 194,
Issue. 5,
p.
1359.
Perrone, Domenico
2016.
On the standard nondegenerate almost CR structure of tangent hyperquadric bundles.
Geometriae Dedicata,
Vol. 185,
Issue. 1,
p.
15.
Abbassi, M.T.K.
Amri, N.
and
Calvaruso, G.
2018.
Kaluza–Klein type Ricci solitons on unit tangent sphere bundles.
Differential Geometry and its Applications,
Vol. 59,
Issue. ,
p.
184.
Perrone, Domenico
2019.
Contact Semi-Riemannian Structures in CR Geometry: Some Aspects.
Axioms,
Vol. 8,
Issue. 1,
p.
6.
Kadaoui Abbassi, Mohamed Tahar
and
Amri, Noura
2020.
Natural Paracontact Magnetic Trajectories on Unit Tangent Bundles.
Axioms,
Vol. 9,
Issue. 3,
p.
72.
Abbassi, Mohamed Tahar Kadaoui
Amri, Noura
and
Calvaruso, Giovanni
2020.
g‐natural symmetries on tangent bundles.
Mathematische Nachrichten,
Vol. 293,
Issue. 10,
p.
1873.
Kadaoui Abbassi, Mohamed Tahar
and
Amri, Noura
2021.
Natural Ricci Solitons on Tangent and Unit Tangent Bundles.
Zurnal matematiceskoj fiziki, analiza, geometrii,
Vol. 17,
Issue. 1,
p.
3.
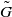 is a Riemannian g-natural metric on T1M of Kaluza–Klein type, then
is a Riemannian g-natural metric on T1M of Kaluza–Klein type, then  is H-contact if and only if (M,g) is 2-stein, so proving that the main result of Chun et al. [‘H-contact unit tangent sphere bundles of Einstein manifolds’, Q. J. Math., to appear. DOI: 10.1093/qmath/hap025] is invariant under a two-parameter deformation of the standard contact metric structure on T1M. Moreover, we completely characterize Riemannian manifolds admitting two distinct H-contact g-natural contact metric structures, with associated metric of Kaluza–Klein type.
is H-contact if and only if (M,g) is 2-stein, so proving that the main result of Chun et al. [‘H-contact unit tangent sphere bundles of Einstein manifolds’, Q. J. Math., to appear. DOI: 10.1093/qmath/hap025] is invariant under a two-parameter deformation of the standard contact metric structure on T1M. Moreover, we completely characterize Riemannian manifolds admitting two distinct H-contact g-natural contact metric structures, with associated metric of Kaluza–Klein type.