I falsely claimed, as an aside remark in [8] and also implicitly in the abstract [9], that the slow-growing hierarchy
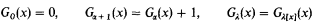
“catches up” with the fast-growing hierarchy
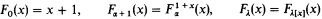
at level Γ0, i.e. that, for all x > 0,
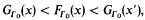
where x′ is some simple (even linear) function of x.
Girard [4] gave the first correct analysis of the deep relationship which exists between G and F, based on his extensive category-theoretic framework for 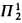 -logic. This analysis indicates that the first point at which G catches up with F is the ordinal of the theory ID<ω(0 of arbitrary finite iterations of an inductive definition. This is very far beyond Γ0! In particular, in order to capture F at level ∣IDn∣ the slow-growing hierarchy must be generated up to ∣IDn+1∣, i.e. one extra iteration of an inductive definition is needed in order to generate sufficient new ordinals.
-logic. This analysis indicates that the first point at which G catches up with F is the ordinal of the theory ID<ω(0 of arbitrary finite iterations of an inductive definition. This is very far beyond Γ0! In particular, in order to capture F at level ∣IDn∣ the slow-growing hierarchy must be generated up to ∣IDn+1∣, i.e. one extra iteration of an inductive definition is needed in order to generate sufficient new ordinals.