Article contents
PERTURBATION OF BANACH SPACE OPERATORS WITH A COMPLEMENTED RANGE
Published online by Cambridge University Press: 21 March 2017
Abstract
Let  ${\mathcal C}[{\mathcal X}]$ be any class of operators on a Banach space
${\mathcal C}[{\mathcal X}]$ be any class of operators on a Banach space 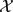 ${\mathcal X}$ , and let
${\mathcal X}$ , and let 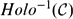 ${Holo}^{-1}({\mathcal C})$ denote the class of operators A for which there exists a holomorphic function f on a neighbourhood
${Holo}^{-1}({\mathcal C})$ denote the class of operators A for which there exists a holomorphic function f on a neighbourhood 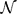 ${\mathcal N}$ of the spectrum σ(A) of A such that f is non-constant on connected components of
${\mathcal N}$ of the spectrum σ(A) of A such that f is non-constant on connected components of 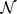 ${\mathcal N}$ and f(A) lies in
${\mathcal N}$ and f(A) lies in 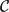 ${\mathcal C}$ . Let
${\mathcal C}$ . Let 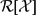 ${{\mathcal R}[{\mathcal X}]}$ denote the class of Riesz operators in
${{\mathcal R}[{\mathcal X}]}$ denote the class of Riesz operators in 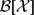 ${{\mathcal B}[{\mathcal X}]}$ . This paper considers perturbation of operators
${{\mathcal B}[{\mathcal X}]}$ . This paper considers perturbation of operators  $A\in\Phi_{+}({\mathcal X})\Cup\Phi_{-}({\mathcal X})$ (the class of all upper or lower [semi] Fredholm operators) by commuting operators in
$A\in\Phi_{+}({\mathcal X})\Cup\Phi_{-}({\mathcal X})$ (the class of all upper or lower [semi] Fredholm operators) by commuting operators in 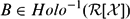 $B\in{Holo}^{-1}({\mathcal R}[{\mathcal X}])$ . We prove (amongst other results) that if πB (B) = ∏m i = 1(B − μi ) is Riesz, then there exist decompositions
$B\in{Holo}^{-1}({\mathcal R}[{\mathcal X}])$ . We prove (amongst other results) that if πB (B) = ∏m i = 1(B − μi ) is Riesz, then there exist decompositions 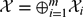 ${\mathcal X}=\oplus_{i=1}^m{{\mathcal X}_i}$ and
${\mathcal X}=\oplus_{i=1}^m{{\mathcal X}_i}$ and 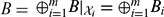 $B=\oplus_{i=1}^m{B|_{{\mathcal X}_i}}=\oplus_{i=1}^m{B_i}$ such that: (i) If λ ≠ 0, then
$B=\oplus_{i=1}^m{B|_{{\mathcal X}_i}}=\oplus_{i=1}^m{B_i}$ such that: (i) If λ ≠ 0, then 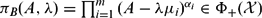 $\pi_B(A,\lambda)=\prod_{i=1}^m{(A-\lambda\mu_i)^{\alpha_i}} \in\Phi_{+}({\mathcal X})$ (resp.,
$\pi_B(A,\lambda)=\prod_{i=1}^m{(A-\lambda\mu_i)^{\alpha_i}} \in\Phi_{+}({\mathcal X})$ (resp., 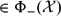 $\in\Phi_{-}({\mathcal X})$ ) if and only if
$\in\Phi_{-}({\mathcal X})$ ) if and only if  $A-\lambda B_0-\lambda\mu_i\in\Phi_{+}({\mathcal X})$ (resp.,
$A-\lambda B_0-\lambda\mu_i\in\Phi_{+}({\mathcal X})$ (resp.,  $\in\Phi_{-}({\mathcal X})$ ), and (ii) (case λ = 0)
$\in\Phi_{-}({\mathcal X})$ ), and (ii) (case λ = 0) 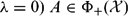 $A\in\Phi_{+}({\mathcal X})$ (resp.,
$A\in\Phi_{+}({\mathcal X})$ (resp., 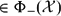 $\in\Phi_{-}({\mathcal X})$ ) if and only if
$\in\Phi_{-}({\mathcal X})$ ) if and only if 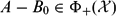 $A-B_0\in\Phi_{+}({\mathcal X})$ (resp.,
$A-B_0\in\Phi_{+}({\mathcal X})$ (resp., 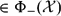 $\in\Phi_{-}({\mathcal X})$ ), where B 0 = ⊕m i = 1(Bi − μi ); (iii) if
$\in\Phi_{-}({\mathcal X})$ ), where B 0 = ⊕m i = 1(Bi − μi ); (iii) if 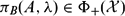 $\pi_B(A,\lambda)\in\Phi_{+}({\mathcal X})$ (resp.,
$\pi_B(A,\lambda)\in\Phi_{+}({\mathcal X})$ (resp., 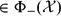 $\in\Phi_{-}({\mathcal X})$ ) for some λ ≠ 0, then
$\in\Phi_{-}({\mathcal X})$ ) for some λ ≠ 0, then  $A-\lambda B\in\Phi_{+}({\mathcal X})$ (resp.,
$A-\lambda B\in\Phi_{+}({\mathcal X})$ (resp., 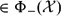 $\in\Phi_{-}({\mathcal X})$ ).
$\in\Phi_{-}({\mathcal X})$ ).
MSC classification
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Glasgow Mathematical Journal Trust 2017
References
REFERENCES
- 2
- Cited by

