Published online by Cambridge University Press: 23 November 2017
Let  $p$ be a prime number and
$p$ be a prime number and 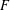 $F$ a totally real number field. For each prime
$F$ a totally real number field. For each prime 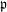 $\mathfrak{p}$ of
$\mathfrak{p}$ of 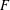 $F$ above
$F$ above 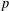 $p$ we construct a Hecke operator
$p$ we construct a Hecke operator 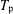 $T_{\mathfrak{p}}$ acting on
$T_{\mathfrak{p}}$ acting on 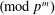 $(\text{mod}\,p^{m})$ Katz Hilbert modular classes which agrees with the classical Hecke operator at
$(\text{mod}\,p^{m})$ Katz Hilbert modular classes which agrees with the classical Hecke operator at 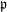 $\mathfrak{p}$ for global sections that lift to characteristic zero. Using these operators and the techniques of patching complexes of Calegari and Geraghty we prove that the Galois representations arising from torsion Hilbert modular classes of parallel weight
$\mathfrak{p}$ for global sections that lift to characteristic zero. Using these operators and the techniques of patching complexes of Calegari and Geraghty we prove that the Galois representations arising from torsion Hilbert modular classes of parallel weight  $\mathbf{1}$ are unramified at
$\mathbf{1}$ are unramified at 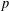 $p$ when
$p$ when 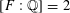 $[F:\mathbb{Q}]=2$. Some partial and some conjectural results are obtained when
$[F:\mathbb{Q}]=2$. Some partial and some conjectural results are obtained when 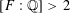 $[F:\mathbb{Q}]>2$.
$[F:\mathbb{Q}]>2$.