Published online by Cambridge University Press: 23 April 2020
We prove the Lipman–Zariski conjecture for complex surface singularities with 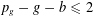 $p_{g}-g-b\leqslant 2$. Here
$p_{g}-g-b\leqslant 2$. Here 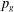 $p_{g}$ is the geometric genus,
$p_{g}$ is the geometric genus, 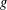 $g$ is the sum of the genera of exceptional curves and
$g$ is the sum of the genera of exceptional curves and 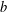 $b$ is the first Betti number of the dual graph. This improves on a previous result of the second author. As an application, we show that a compact complex surface with a locally free tangent sheaf is smooth as soon as it admits two generically linearly independent twisted vector fields and its canonical sheaf has at most two global sections.
$b$ is the first Betti number of the dual graph. This improves on a previous result of the second author. As an application, we show that a compact complex surface with a locally free tangent sheaf is smooth as soon as it admits two generically linearly independent twisted vector fields and its canonical sheaf has at most two global sections.