Published online by Cambridge University Press: 12 November 2021
In this paper, we classify the three-dimensional partially hyperbolic diffeomorphisms whose stable, unstable, and central distributions 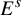 $E^s$,
$E^s$,  $E^u$, and
$E^u$, and 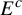 $E^c$ are smooth, such that
$E^c$ are smooth, such that  $E^s\oplus E^u$ is a contact distribution, and whose non-wandering set equals the whole manifold. We prove that up to a finite quotient or a finite power, they are smoothly conjugated either to a time-map of an algebraic contact-Anosov flow, or to an affine partially hyperbolic automorphism of a nil-
$E^s\oplus E^u$ is a contact distribution, and whose non-wandering set equals the whole manifold. We prove that up to a finite quotient or a finite power, they are smoothly conjugated either to a time-map of an algebraic contact-Anosov flow, or to an affine partially hyperbolic automorphism of a nil-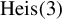 ${\mathrm {Heis}}{(3)}$-manifold. The rigid geometric structure induced by the invariant distributions plays a fundamental part in the proof.
${\mathrm {Heis}}{(3)}$-manifold. The rigid geometric structure induced by the invariant distributions plays a fundamental part in the proof.