Published online by Cambridge University Press: 25 July 2014
A conjecture of Manin predicts the distribution of rational points on Fano varieties. We provide a framework for proofs of Manin’s conjecture for del Pezzo surfaces over imaginary quadratic fields, using universal torsors. Some of our tools are formulated over arbitrary number fields. As an application, we prove Manin’s conjecture over imaginary quadratic fields 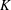 $K$ for the quartic del Pezzo surface
$K$ for the quartic del Pezzo surface 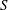 $S$ of singularity type
$S$ of singularity type 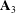 ${\boldsymbol{A}}_{3}$ with five lines given in
${\boldsymbol{A}}_{3}$ with five lines given in 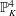 ${\mathbb{P}}_{K}^{4}$ by the equations
${\mathbb{P}}_{K}^{4}$ by the equations 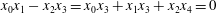 ${x}_{0}{x}_{1}-{x}_{2}{x}_{3}={x}_{0}{x}_{3}+{x}_{1}{x}_{3}+{x}_{2}{x}_{4}=0$.
${x}_{0}{x}_{1}-{x}_{2}{x}_{3}={x}_{0}{x}_{3}+{x}_{1}{x}_{3}+{x}_{2}{x}_{4}=0$.