Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Nussenzveig Lopes, Helena J
Seis, Christian
and
Wiedemann, Emil
2021.
On the vanishing viscosity limit for 2D incompressible flows with unbounded vorticity.
Nonlinearity,
Vol. 34,
Issue. 5,
p.
3112.
Ciampa, Gennaro
Crippa, Gianluca
and
Spirito, Stefano
2021.
Strong Convergence of the Vorticity for the 2D Euler Equations in the Inviscid Limit.
Archive for Rational Mechanics and Analysis,
Vol. 240,
Issue. 1,
p.
295.
Navarro-Fernández, Víctor
Schlichting, André
and
Seis, Christian
2022.
Optimal stability estimates and a new uniqueness result for advection-diffusion equations.
Pure and Applied Analysis,
Vol. 4,
Issue. 3,
p.
571.
Matharu, Pritpal
Protas, Bartosz
and
Yoneda, Tsuyoshi
2022.
On maximum enstrophy dissipation in 2D Navier–Stokes flows in the limit of vanishing viscosity.
Physica D: Nonlinear Phenomena,
Vol. 441,
Issue. ,
p.
133517.
Ceci, Stefano
and
Seis, Christian
2022.
On the dynamics of point vortices for the two-dimensional Euler equation with
L
p
vorticity
.
Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 380,
Issue. 2226,
Ceci, Stefano
and
Seis, Christian
2024.
On the dynamics of vortices in viscous 2D flows.
Mathematische Annalen,
Vol. 388,
Issue. 2,
p.
1937.
Franzoi, Luca
and
Montalto, Riccardo
2024.
A KAM Approach to the Inviscid Limit for the 2D Navier–Stokes Equations.
Annales Henri Poincaré,
Vol. 25,
Issue. 12,
p.
5231.
Ciampa, Gennaro
Crippa, Gianluca
and
Spirito, Stefano
2024.
Propagation of logarithmic regularity and inviscid limit for the 2D Euler equations.
Mathematics in Engineering,
Vol. 6,
Issue. 4,
p.
494.
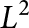 $L^2$ with an order proportional to the square root of the viscosity constant
$L^2$ with an order proportional to the square root of the viscosity constant 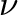 $\nu $. Here, we provide an order
$\nu $. Here, we provide an order 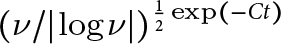 $ (\nu /|\log \nu | )^{\frac 12\exp (-Ct)}$ bound, which slightly improves upon earlier results by Chemin.
$ (\nu /|\log \nu | )^{\frac 12\exp (-Ct)}$ bound, which slightly improves upon earlier results by Chemin.