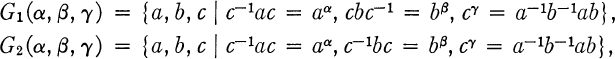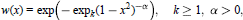Most cited
This page lists all time most cited articles for this title. Please use the publication date filters on the left if you would like to restrict this list to recently published content, for example to articles published in the last three years. The number of times each article was cited is displayed to the right of its title and can be clicked to access a list of all titles this article has been cited by.
- Cited by 13
Antichains and Finite Sets that Meet all Maximal Chains
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 619-632
-
- Article
-
- You have access
- Export citation
- Cited by 13
Subgroups of the Power Semigroup of a Finite Semigroup
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1077-1083
-
- Article
-
- You have access
- Export citation
- Cited by 13
Tensor Products of Analytic Continuations of Holomorphic Discrete Series
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1224-1241
-
- Article
-
- You have access
- Export citation
- Cited by 12
Shimura Varieties and the Selberg Trace Formula
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1292-1299
-
- Article
-
- You have access
- Export citation
- Cited by 12
Hall Higman Type Theorems, I
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 513-531
-
- Article
-
- You have access
- Export citation
- Cited by 12
A Classification of Homogeneous Surfaces
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1097-1110
-
- Article
-
- You have access
- Export citation
- Cited by 12
Common Fixed Points of Commuting Monotone Mappings
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 617-620
-
- Article
-
- You have access
- Export citation
- Cited by 12
A Short Proof of the Cartwright-Littlewood Fixed Point Theorem
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 522-524
-
- Article
-
- You have access
- Export citation
- Cited by 12
Dynamics and Regularization of the Kepler Problem on Surfaces of Constant Curvature
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 961-991
-
- Article
-
- You have access
- Export citation
- Cited by 12
Boundedness of Solutions of Parabolic Equations With Anisotropic Growth Conditions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 798-809
-
- Article
-
- You have access
- Export citation
- Cited by 12
Distance Matrices and Ridge Function Interpolation
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1313-1323
-
- Article
-
- You have access
- Export citation
- Cited by 12
Reconstruction of Entire Functions From Irregularly Spaced Sample Points
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 777-793
-
- Article
-
- You have access
- Export citation
- Cited by 12
On Dihedral Galois Coverings
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1299-1317
-
- Article
-
- You have access
- Export citation
- Cited by 12
The Maximum Genus of Cartesian Products of Graphs
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1025-1035
-
- Article
-
- You have access
- Export citation
- Cited by 12
On Kaehler Immersions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1178-1182
-
- Article
-
- You have access
- Export citation
- Cited by 12
Local Topological Properties and One Point Extensions
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 338-348
-
- Article
-
- You have access
- Export citation
- Cited by 12
An Abstract Concept of the Sum of a Numerical Series
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 863-874
-
- Article
-
- You have access
- Export citation
- Cited by 12
Extreme and Exposed Points in Orlicz Spaces
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 505-515
-
- Article
-
- You have access
- Export citation
- Cited by 12
A Class of Three-Generator, Three-Relation, Finite Groups
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 36-40
-
- Article
-
- You have access
- Export citation
- Cited by 12
Mean Convergence of Lagrange Interpolation for Exponential weights on [-1, 1]
-
- Published online by Cambridge University Press:
- 20 November 2018, pp. 1273-1297
-
- Article
-
- You have access
- Export citation
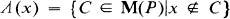

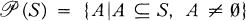 . If
. If 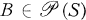 , then let
, then let 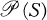 has been studied by many authors, including
has been studied by many authors, including 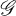 a subgroup of
a subgroup of 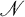 such that
such that  and
and  is isomorphic to a subgroup of
is isomorphic to a subgroup of 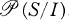 .
.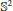
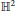
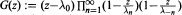 where {
where {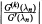 is bounded by a constant independent of
is bounded by a constant independent of 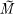 be an
be an  . B. O'Neill [
. B. O'Neill [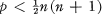 and if the holomorphic sectional curvature of M with respect to the induced Kaehler metric is constant, then M is totally geodesic.
and if the holomorphic sectional curvature of M with respect to the induced Kaehler metric is constant, then M is totally geodesic.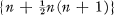 -dimensional complex projective space of constant holomorphic sectional curvature 1. This shows that Proposition A is the best possible.
-dimensional complex projective space of constant holomorphic sectional curvature 1. This shows that Proposition A is the best possible.