No CrossRef data available.
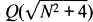
Published online by Cambridge University Press: 20 November 2018
The purpose of this paper is to address conjectures raised in [2]. We show that one of the conjectures is false and we advance the proof of another by proving it for an infinite set of cases. Furthermore, we give hard evidence as to why the conjecture is true and show what remains to be done to complete the proof. Finally, we prove a conjecture given by S. Louboutin, for Mathematical Reviews, in his discussion of the aforementioned paper.