Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Smith, Tara L.
1994.
Extra-Special Groups of Order 32 as Galois Groups.
Canadian Journal of Mathematics,
Vol. 46,
Issue. 4,
p.
886.
Dentzer, Ralf
1995.
On geometric embedding problems and semiabelian groups.
Manuscripta Mathematica,
Vol. 86,
Issue. 1,
p.
199.
Ledet, Arne
1995.
On 2-Groups as Galois Groups.
Canadian Journal of Mathematics,
Vol. 47,
Issue. 6,
p.
1253.
Drees, Christof
Epkenhans, Martin
and
Krüskemper, Martin
1997.
On the Computation of the Trace Form of Some Galois Extensions.
Journal of Algebra,
Vol. 192,
Issue. 1,
p.
209.
Ledet, Arne
1998.
Embedding problems with cyclic kernel of order 4.
Israel Journal of Mathematics,
Vol. 106,
Issue. 1,
p.
109.
Ledet, Arne
1999.
Generic polynomials for quasi-dihedral, dihedral and modular extensions of order 16.
Proceedings of the American Mathematical Society,
Vol. 128,
Issue. 8,
p.
2213.
Black, Elena
1999.
Deformations of dihedral 2-group extensions of fields.
Transactions of the American Mathematical Society,
Vol. 351,
Issue. 8,
p.
3229.
Ledet, Arne
2000.
Generic and Explicit Realization of Smallp-groups.
Journal of Symbolic Computation,
Vol. 30,
Issue. 6,
p.
859.
Ledet, Arne
2000.
Generic Extensions and Generic Polynomials.
Journal of Symbolic Computation,
Vol. 30,
Issue. 6,
p.
867.
Michailov, Ivo M.
and
Ziapkov, Nikola P.
2000.
Embedding Obstructions for the Generalized Quaternion Group.
Journal of Algebra,
Vol. 226,
Issue. 1,
p.
375.
Michailov, Ivo M
2001.
Embedding Obstructions for the Dihedral, Semidihedral, and Quaternion 2-Groups.
Journal of Algebra,
Vol. 245,
Issue. 1,
p.
355.
Swallow, John R.
2001.
QUADRATIC DESCENT FOR QUATERNION ALGEBRAS.
Communications in Algebra,
Vol. 29,
Issue. 10,
p.
4523.
Hwang, Y.-S.
Leep, David B.
and
Wadsworth, Adrian R.
2003.
Galois groups of order 2n that contain a cyclic subgroup of order n.
Pacific Journal of Mathematics,
Vol. 212,
Issue. 2,
p.
297.
Grundman, Helen G.
and
Stewart, Grisha L.
2004.
Galois realizability of non-split group extensions of C2 by (C2)r×(C4)s×(D4)t.
Journal of Algebra,
Vol. 272,
Issue. 2,
p.
425.
Geyer, Wulf-Dieter
and
Jensen, Christian U
2007.
Embeddability of quadratic extensions in cyclic extensions.
Forum Mathematicum,
Vol. 19,
Issue. 4,
Michailov, Ivo M.
2007.
Four non-abelian groups of order p4 as Galois groups.
Journal of Algebra,
Vol. 307,
Issue. 1,
p.
287.
Michailov, Ivo M.
2011.
On Galois cohomology and realizability of 2-groups as Galois groups.
Central European Journal of Mathematics,
Vol. 9,
Issue. 2,
p.
403.
Hoshi, Akinari
Kang, Ming-chang
and
Kitayama, Hidetaka
2014.
Quasi-monomial actions and some 4-dimensional rationality problems.
Journal of Algebra,
Vol. 403,
Issue. ,
p.
363.
SHANKAR, ARUL
SÖDERGREN, ANDERS
and
TEMPLIER, NICOLAS
2019.
SATO–TATE EQUIDISTRIBUTION OF CERTAIN FAMILIES OF ARTIN -FUNCTIONS.
Forum of Mathematics, Sigma,
Vol. 7,
Issue. ,
Bruin, Peter
and
Ferraguti, Andrea
2019.
Strongly modular models of ℚ-curves.
International Journal of Number Theory,
Vol. 15,
Issue. 03,
p.
505.

 be a finite group. Let
be a finite group. Let 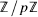 , where
, where 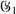 the Galois group of
the Galois group of