Published online by Cambridge University Press: 20 November 2018
We describe the set of numbers 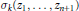 ${{\sigma }_{k}}\left( {{z}_{1}},\cdot \cdot \cdot ,{{z}_{n+1}} \right)$, where
${{\sigma }_{k}}\left( {{z}_{1}},\cdot \cdot \cdot ,{{z}_{n+1}} \right)$, where 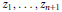 ${{z}_{1}},\cdot \cdot \cdot ,{{z}_{n+1}}$ are complex numbers of modulus 1 for which
${{z}_{1}},\cdot \cdot \cdot ,{{z}_{n+1}}$ are complex numbers of modulus 1 for which 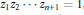 ${{z}_{1}}{{z}_{2}}\cdot \cdot \cdot {{z}_{n+1}}=1$, and
${{z}_{1}}{{z}_{2}}\cdot \cdot \cdot {{z}_{n+1}}=1$, and  ${{\sigma }_{k}}$ denotes the
${{\sigma }_{k}}$ denotes the  $k$-th elementary symmetric polynomial. Consequently, we give sharp constraints on the coefficients of a complex polynomial all of whose roots are of the same modulus. Another application is the calculation of the spectrum of certain adjacency operators arising naturally on a building of type
$k$-th elementary symmetric polynomial. Consequently, we give sharp constraints on the coefficients of a complex polynomial all of whose roots are of the same modulus. Another application is the calculation of the spectrum of certain adjacency operators arising naturally on a building of type 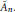 ${{\overset{\sim }{\mathop{\text{A}}}\,}_{n}}$.
${{\overset{\sim }{\mathop{\text{A}}}\,}_{n}}$.