No CrossRef data available.
Article contents
Descending Rational Points on Elliptic Curves to Smaller Fields
Published online by Cambridge University Press: 20 November 2018
Abstract
In this paper, we study the Mordell-Weil group of an elliptic curve as a Galois module. We consider an elliptic curve 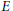 $E$ defined over a number field
$E$ defined over a number field 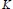 $K$ whose Mordell-Weil rank over a Galois extension
$K$ whose Mordell-Weil rank over a Galois extension  $F$ is 1, 2 or 3. We show that
$F$ is 1, 2 or 3. We show that 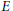 $E$ acquires a point (points) of infinite order over a field whose Galois group is one of
$E$ acquires a point (points) of infinite order over a field whose Galois group is one of 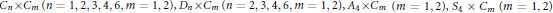 ${{C}_{n}}\times {{C}_{m}}(n=1,\,2,\,3,\,4,\,6,\,m\,=\,1,\,2),\,{{D}_{n}}\times {{C}_{m}}(n=2,\,3,\,4,\,6,\,m=\,1,\,2),\,{{A}_{4}}\times {{C}_{m}}(m=1,\,2),\,{{S}_{4}}\,\times \,{{C}_{m}}(m=1,\,2)$ . Next, we consider the case where
${{C}_{n}}\times {{C}_{m}}(n=1,\,2,\,3,\,4,\,6,\,m\,=\,1,\,2),\,{{D}_{n}}\times {{C}_{m}}(n=2,\,3,\,4,\,6,\,m=\,1,\,2),\,{{A}_{4}}\times {{C}_{m}}(m=1,\,2),\,{{S}_{4}}\,\times \,{{C}_{m}}(m=1,\,2)$ . Next, we consider the case where 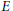 $E$ has complex multiplication by the ring of integers
$E$ has complex multiplication by the ring of integers 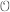 $\mathcal{O}$ of an imaginary quadratic field
$\mathcal{O}$ of an imaginary quadratic field 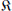 $\Re $ contained in
$\Re $ contained in 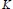 $K$ . Suppose that the
$K$ . Suppose that the 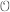 $\mathcal{O}$ -rank over a Galois extension
$\mathcal{O}$ -rank over a Galois extension  $F$ is 1 or 2. If
$F$ is 1 or 2. If 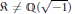 $\Re \ne \mathbb{Q}(\sqrt{-1})$ and
$\Re \ne \mathbb{Q}(\sqrt{-1})$ and 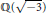 $\mathbb{Q}(\sqrt{-3})$ and
$\mathbb{Q}(\sqrt{-3})$ and 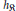 ${{h}_{\Re }}$ (class number of
${{h}_{\Re }}$ (class number of 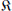 $\Re $ ) is odd, we show that
$\Re $ ) is odd, we show that 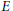 $E$ acquires positive
$E$ acquires positive 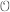 $\mathcal{O}$ -rank over a cyclic extension of
$\mathcal{O}$ -rank over a cyclic extension of 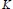 $K$ or over a field whose Galois group is one of
$K$ or over a field whose Galois group is one of 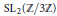 $\text{S}{{\text{L}}_{2}}(\mathbb{Z}/3\mathbb{Z})$ , an extension of
$\text{S}{{\text{L}}_{2}}(\mathbb{Z}/3\mathbb{Z})$ , an extension of 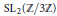 $\text{S}{{\text{L}}_{2}}(\mathbb{Z}/3\mathbb{Z})$ by
$\text{S}{{\text{L}}_{2}}(\mathbb{Z}/3\mathbb{Z})$ by 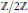 $\mathbb{Z}/2\mathbb{Z}$ , or a central extension by the dihedral group. Finally, we discuss the relation of the above results to the vanishing of
$\mathbb{Z}/2\mathbb{Z}$ , or a central extension by the dihedral group. Finally, we discuss the relation of the above results to the vanishing of 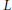 $L$ -functions.
$L$ -functions.
Information
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2001

