Published online by Cambridge University Press: 20 March 2019
Motivated by Ramanujan’s continued fraction and the work of Richmond and Szekeres [‘The Taylor coefficients of certain infinite products’, Acta Sci. Math. (Szeged)40(3–4) (1978), 347–369], we investigate vanishing coefficients along arithmetic progressions in four quotients of infinite product expansions and obtain similar results. For example, 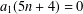 $a_{1}(5n+4)=0$, where
$a_{1}(5n+4)=0$, where 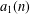 $a_{1}(n)$ is defined by
$a_{1}(n)$ is defined by 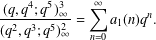 $$\begin{eqnarray}\displaystyle {\displaystyle \frac{(q,q^{4};q^{5})_{\infty }^{3}}{(q^{2},q^{3};q^{5})_{\infty }^{2}}}=\mathop{\sum }_{n=0}^{\infty }a_{1}(n)q^{n}. & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle {\displaystyle \frac{(q,q^{4};q^{5})_{\infty }^{3}}{(q^{2},q^{3};q^{5})_{\infty }^{2}}}=\mathop{\sum }_{n=0}^{\infty }a_{1}(n)q^{n}. & & \displaystyle \nonumber\end{eqnarray}$$
This work was supported by the National Natural Science Foundation of China (No. 11501061) and the Fundamental Research Funds for the Central Universities (No. 2018CDXYST0024).