No CrossRef data available.
Published online by Cambridge University Press: 10 July 2019
A polynomial 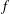 $f$ over a finite field
$f$ over a finite field 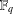 $\mathbb{F}_{q}$ can be classified as a permutation polynomial by the Hermite–Dickson criterion, which consists of conditions on the powers
$\mathbb{F}_{q}$ can be classified as a permutation polynomial by the Hermite–Dickson criterion, which consists of conditions on the powers 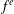 $f^{e}$ for each
$f^{e}$ for each  $e$ from
$e$ from 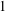 $1$ to
$1$ to  $q-2$, as well as the existence of a unique solution to
$q-2$, as well as the existence of a unique solution to  $f(x)=0$ in
$f(x)=0$ in 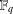 $\mathbb{F}_{q}$. Carlitz and Lutz gave a variant of the criterion. In this paper, we provide an alternate proof to the theorem of Carlitz and Lutz.
$\mathbb{F}_{q}$. Carlitz and Lutz gave a variant of the criterion. In this paper, we provide an alternate proof to the theorem of Carlitz and Lutz.
The second and third authors were supported by NSERC.