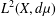Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Bui, The Anh
Duong, Xuan Thinh
and
Ly, Fu Ken
2018.
Maximal function characterizations for new local Hardy-type spaces on spaces of homogeneous type.
Transactions of the American Mathematical Society,
Vol. 370,
Issue. 10,
p.
7229.
He, Yao
and
Liao, Jianquan
2025.
Maximal Function Characterizations for Local Variable Hardy Spaces Associated to Non-negative Self-Adjoint Operators on Spaces of Homogeneous Type.
Complex Analysis and Operator Theory,
Vol. 19,
Issue. 4,